The surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as ρ(r)=A+Br. Find its mment of inertia about the line perpendicular to the plane of the disc through its centre.Solution in Gujrati
The correct Answer is:A, B, D
The surface density of a circular disc of radius a depends upon the distance from the centre as P(r)=A+Br.
Therefore the mass of the ring of radius r will be
θ=(a+Br)×2πr×dr
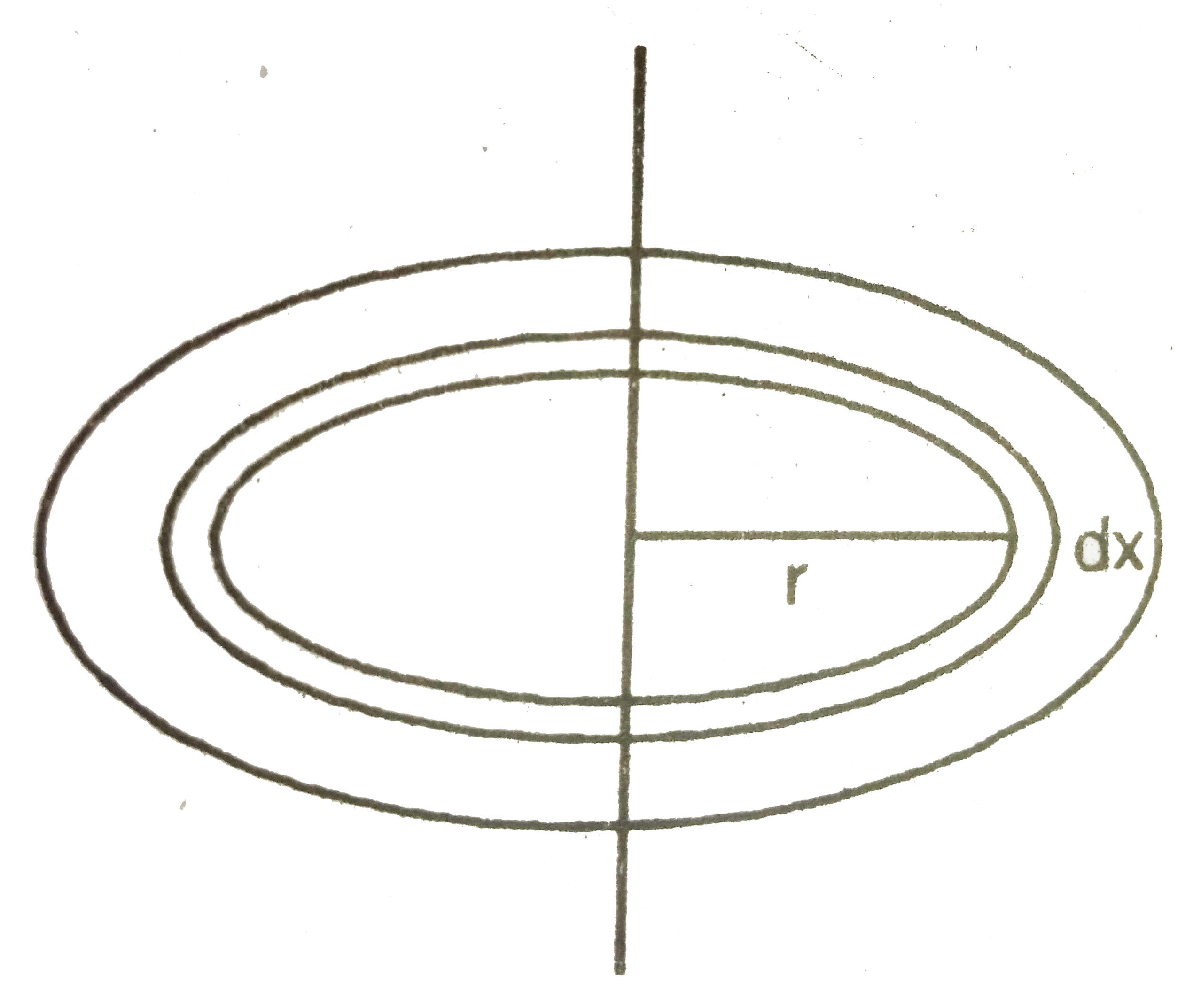
Therefore moment of inertila about the centre
=∫0a(A+Br)2πr.dr×r2
=a∫02aπAr3dr+∫0a2πBr4dr
=[2πA(r44)+2πB(r55)]0a
=2π(Aa44+Ba55)
Similar Questions
The radius of gyration of a uniform disc about a line perpendicular to the disc equals to its radius. Find the distance of the line from the centre.
View SolutionThe surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as ρ(r)=A+Br. Find its moment of inertia about the line perpendicular to the plane of the disc through its centre.
View SolutionThe surface mass density of a disc of radius a varies with radial distance as sigma = A + Br where A & B are positive constants then moment of inertia of the disc about an axis passing through its centre and perpendicular to the plane
View SolutionThe surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as ρ(r)=A+Br. Find its moment of inertia about the line perpendicular to the plane of the disc through its centre.
View SolutionCalculate the moment of inertia of uniform circular disc of mass 500 g, radius 10 cm about : the axis through the centre of the disc and perpendicular to its plane
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the centre is ………..
View SolutionThe moment of inertia of a uniform semi circular disc of mass M and radius .r. about a line perpendicular to the plane of disc through the centre is:
View SolutionA solid disc has a radius R. Density of its material Is given by ρ=ρ0(2−rR) where ρ0,is a constant and r is the radial distance measured from the centre. Then the moment of inertia of the disc about an axis passing through its centre and perpendicular to the plane of the disc is
View Solution

