From a circular disc of radius R and 9M , a small disc of mass M and radius R3 is removed concentrically .The moment of inertia of the remaining disc about and axis perpendicular to the plane of the disc and passing through its centre is
409MR2
MR2
4MR2
49MR2
The correct Answer is:A
To find the moment of inertia of the remaining disc after removing a smaller disc from a larger disc, we will follow these steps:
Step 1: Understand the Problem
We have a larger disc of radius R and mass 9M. A smaller disc of radius R3 and mass M is removed from the center of the larger disc. We need to calculate the moment of inertia of the remaining part of the disc about an axis perpendicular to the plane of the disc and passing through its center.
Step 2: Calculate the Moment of Inertia of the Larger Disc
The moment of inertia I1 of a solid disc about an axis perpendicular to its plane and passing through its center is given by the formula:
I=12MR2
For the larger disc:
I1=12×9M×R2=9MR22
Step 3: Calculate the Moment of Inertia of the Smaller Disc
The moment of inertia I2 of the smaller disc (which we will treat as a negative mass since it is removed) is calculated using the same formula, but with its radius R3 and mass M:
I2=12M(R3)2=12MR29=MR218
Step 4: Adjust for the Removal of the Smaller Disc
Since we are removing the smaller disc, we treat its moment of inertia as negative:
Iremaining=I1−I2=9MR22−MR218
Step 5: Find a Common Denominator and Simplify
To combine these fractions, we need a common denominator. The least common multiple of 2 and 18 is 18:
Iremaining=9MR2⋅918−MR218=81MR218−MR218=80MR218
Step 6: Simplify the Result
Now we can simplify 80MR218:
Iremaining=40MR29
Final Answer
The moment of inertia of the remaining disc about the specified axis is:
40MR29
---
We have a larger disc of radius R and mass 9M. A smaller disc of radius R3 and mass M is removed from the center of the larger disc. We need to calculate the moment of inertia of the remaining part of the disc about an axis perpendicular to the plane of the disc and passing through its center.
The moment of inertia I1 of a solid disc about an axis perpendicular to its plane and passing through its center is given by the formula:
I=12MR2
For the larger disc:
I1=12×9M×R2=9MR22
The moment of inertia I2 of the smaller disc (which we will treat as a negative mass since it is removed) is calculated using the same formula, but with its radius R3 and mass M:
I2=12M(R3)2=12MR29=MR218
Since we are removing the smaller disc, we treat its moment of inertia as negative:
Iremaining=I1−I2=9MR22−MR218
To combine these fractions, we need a common denominator. The least common multiple of 2 and 18 is 18:
Iremaining=9MR2⋅918−MR218=81MR218−MR218=80MR218
Now we can simplify 80MR218:
Iremaining=40MR29
The moment of inertia of the remaining disc about the specified axis is:
40MR29
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - IV
Explore 39 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - II (H.W)
Explore 60 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-VI
Explore 78 Videos
- THERMAL PROPERTIES OF MATTERBook:NARAYNAChapter:THERMAL PROPERTIES OF MATTERExercise:LEVEL - II (H.W.)
Explore 19 Videos
Similar Questions
From a circular disc of radius R and mass 9M, a small disc of radius R/3 is removed as shown in figure. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is

View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View Solution
Knowledge Check
From a circular disc of radius R and mass 9 M , a small disc of mass M and radius R3 is removed concentrically . The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through its centre is
A409MR2BMR2C4MR2D49MR2Form a circular disc of radius R and mass 9M , a small disc of mass M and radius R/3 is removed concentrically . The moment of inertia of the remaining disc about an axis perpendicular to the the plane of the disc and passing its centre is :-
AMR2B4MR2C49MR2D409MR2From a circular disc of radius R and mass 9M, a small disc of radius R/3 is removed from the disc. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is
 A4M2B409MR2C10MR2D379MR2
A4M2B409MR2C10MR2D379MR2
The M.I. of a uniform semicircular disc of mass M and radius R about a line perpendicular to the plane of the disc and passing through the centre is
View SolutionA thin disc of mass 9M and radius R from which a disc of radius R/3 is cut shown in figure. Then moment of inertia of the remaining disc about O, perpendicular to the plane of disc is -
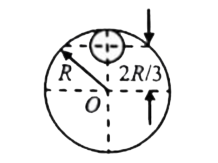
View SolutionA rectangular piece of dimension l×b is cut out of central portion of a uniform circular disc of mass m and radius r. The moment of inertia of the remaining piece about an axis perpendicular to the plane of the disc and passing through its centre is :
View SolutionThe moment of inertia of a circular disc about an axis passing through the circumstances perpendicular to the plane of the disc is
View SolutionTwo thin discs each of mass M and radius r are attached as shown in figure, to from a rigid body. The rotational inertia of this body about an axis perpendicular to the plane of disc B and passing through its centre is :
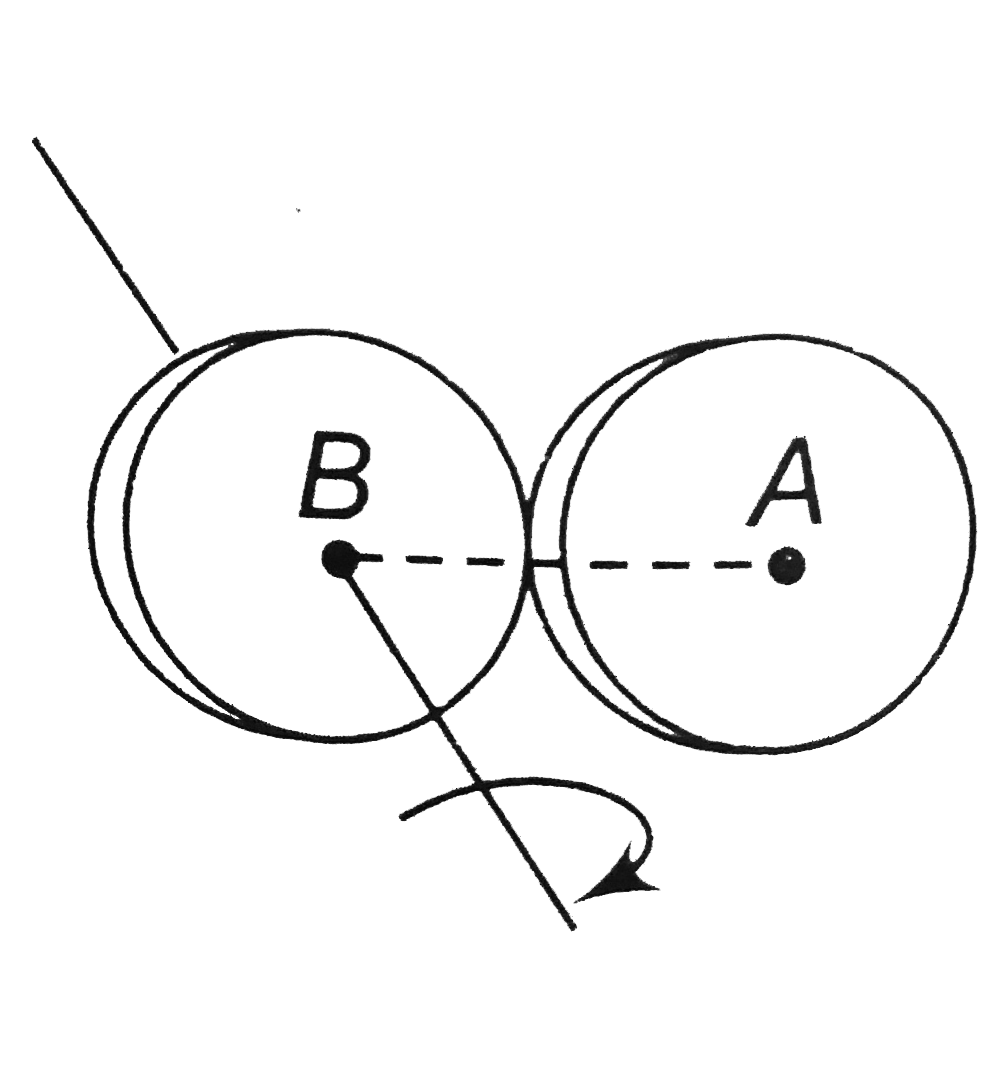 .
.
View Solution