A 2μF Capacitor is connected to a.d.c source of 100 volt through 1MΩ series resistance . Calculate initial charging current .
To solve the problem of calculating the initial charging current of a capacitor connected to a DC source, we can follow these steps:
Step 1: Identify the given values
- Capacitance (C) = 2 µF = 2×10−6 F
- Voltage (V₀) = 100 V
- Resistance (R) = 1 MΩ = 1×106 Ω
Step 2: Use the formula for initial charging current
The initial charging current (I₀) can be calculated using the formula:
I0=V0R
Step 3: Substitute the values into the formula
Now, substituting the known values into the formula:
I0=100V1×106Ω
Step 4: Calculate the initial charging current
Perform the division:
I0=100106=100×10−6A
This can be expressed in microamperes:
I0=100μA
Final Answer
The initial charging current is 100μA.
---
- Capacitance (C) = 2 µF = 2×10−6 F
- Voltage (V₀) = 100 V
- Resistance (R) = 1 MΩ = 1×106 Ω
The initial charging current (I₀) can be calculated using the formula:
I0=V0R
Now, substituting the known values into the formula:
I0=100V1×106Ω
Perform the division:
I0=100106=100×10−6A
This can be expressed in microamperes:
I0=100μA
The initial charging current is 100μA.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE (LONG ANSWER QUESTIONS)
12 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE (SHORT ANSWER QUESTIONS)
12 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH SERIESCHAPTER - COMMUNICATION SYSTEMS EXERCISE - PRACTICE EXERCISE (TV Antenna)
16 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE - IA
2 Videos
Similar Questions
A parallel combination of 0.1MΩ resistor and a 10μF capacitor is connected across a 1.5V source of negligible resistance. The time required for the capacitor to get charged up to 0.75 V is approximately (in seconds).
View SolutionA parallel combination of 0.1 MΩ resistor and a 10μF capacitor is connected across a 1.5 V source of negligible resistance. The time (in sec) required for the capacitor to get charged upto 0.75 V is approximately
View SolutionA 20(μ)Fcapacitor is joined to a battery of emf 6.0V through a resistance of 100(Ω).find the charge on the capacitor 2.0ms after the connections are made .
View SolutionAn inductor coil is connected to an source through a 60Ω resistance in series. The source voltage, voltage across the coil and voltage across the resistance are found to be 33V, 27V and 12V respectively. The resistance of the coil is
A. 30Ω
B. 45Ω
C. 105Ω
D. 75Ω
View SolutionTwo capacitors of capacitance 2μFand5μF are charged to a potential difference 100V and 50 V respectively and connected such that the positive plate of one capacitor is connected to the negative plate of the other capacitor after the switch is closed, the initial current in the circuit is 50 mA. the total resistance of the connecting wires is (in Ohm):
View SolutionA charged capacitor of unknown capacitance is connected in series with 100kΩ resistance and an ideal ammeter. The initial current in the circuit is found to be 0.2mA and the current after 7 sec is found to be 0.1mA. The potential energy that was stored in the capacitor before it was connected in the circuit is ______mJ. [Take log22=0.7]
View SolutionFive capacitors of 10μf capacity each are connected to a.d.c potential of 100 volts as shown in the adjoining figure. The equivalent capacitance between the points A and B will be equal to
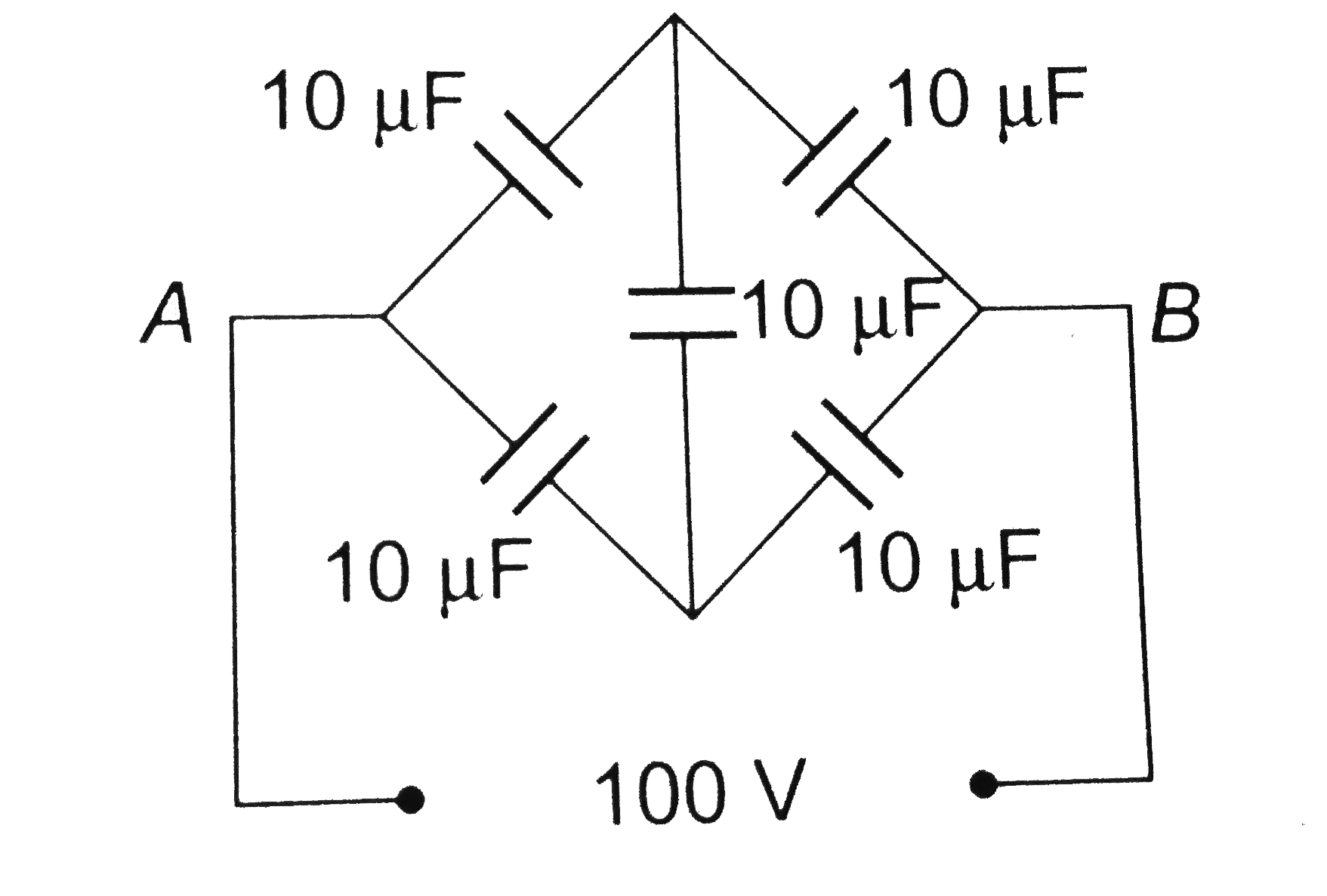
View SolutionA cell of e.m.f. 1.5 V, internal resistance 1 Ω is connected to the resistors of 4 Ω and 20Ω in series. Draw a circuit diagram and calculate :
the current in the circuit,
View SolutionA capacitor of capacitance 2μF is charged to a potential difference of 5V. Now, the charging battery is disconected and the capacitor is connected in parallel to a resistor of 5Ω and another unknown resistor of resistance R as shown in figure. If the total heat produced in 5Ω resistance is 10μJ then the unknown resistance R is equal to
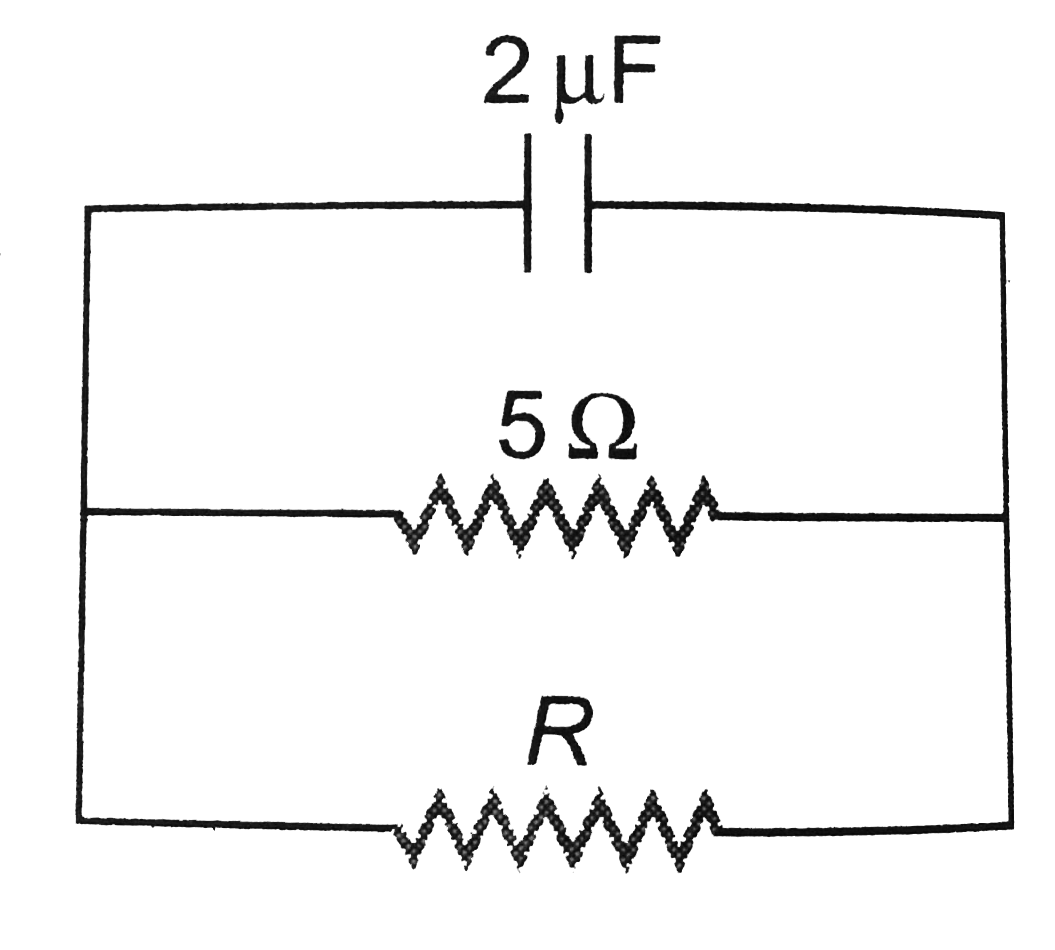
View SolutionA capacitor of capacitance 2μF is charged to a potential difference of 5V. Now, the charging battery is disconected and the capacitor is connected in parallel to a resistor of 5Ω and another unknown resistor of resistance R as shown in figure. If the total heat produced in 5Ω resistance is 10μJ then the unknown resistance R is equal to

View Solution
