When a current drawn from a battery is 0.5 A, its terminal potential difference is 20 V. And when current drawn from it is 2.0 A , the terminal voltage reduces to 16 V . Find out e.m.f and internal resistance of the battery.
To solve the problem, we will use the relationship between the electromotive force (e.m.f.), terminal voltage, current, and internal resistance of the battery. The formula we will use is:
V=E−i⋅r
Where:
- V is the terminal voltage,
- E is the e.m.f. of the battery,
- i is the current drawn from the battery,
- r is the internal resistance of the battery.
Step 1: Set up the equations based on the given conditions.
1. When the current i1=0.5A, the terminal voltage V1=20V:
20=E−0.5r(Equation 1)
2. When the current i2=2.0A, the terminal voltage V2=16V:
16=E−2r(Equation 2)
Step 2: Solve the equations simultaneously.
We have two equations:
1. E−0.5r=20
2. E−2r=16
We can rearrange both equations to express E:
- From Equation 1:
E=20+0.5r(Equation 3)
- From Equation 2:
E=16+2r(Equation 4)
Step 3: Set Equations 3 and 4 equal to each other.
Since both equations equal E, we can set them equal to each other:
20+0.5r=16+2r
Step 4: Solve for r.
Rearranging the equation gives:
20−16=2r−0.5r
4=1.5r
r=41.5=4015=83Ω≈2.67Ω
Step 5: Substitute r back to find E.
Now, substitute r back into either Equation 3 or Equation 4 to find E. Using Equation 3:
E=20+0.5(83)
E=20+43
E=603+43=643V≈21.33V
Final Results:
- The internal resistance r of the battery is 83Ω or approximately 2.67Ω.
- The e.m.f. E of the battery is 643V or approximately 21.33V.
- V is the terminal voltage,
- E is the e.m.f. of the battery,
- i is the current drawn from the battery,
- r is the internal resistance of the battery.
20=E−0.5r(Equation 1)
16=E−2r(Equation 2)
1. E−0.5r=20
2. E−2r=16
- From Equation 1:
E=20+0.5r(Equation 3)
E=16+2r(Equation 4)
20+0.5r=16+2r
20−16=2r−0.5r
4=1.5r
r=41.5=4015=83Ω≈2.67Ω
E=20+0.5(83)
E=20+43
E=603+43=643V≈21.33V
- The internal resistance r of the battery is 83Ω or approximately 2.67Ω.
- The e.m.f. E of the battery is 643V or approximately 21.33V.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:AAKASH SERIESChapter:CURRENT ELECTRICITYExercise:EXERCISE (LONG ANSWER QUESTIONS)
Explore 12 Videos
- CURRENT ELECTRICITYBook:AAKASH SERIESChapter:CURRENT ELECTRICITYExercise:EXERCISE (SHORT ANSWER QUESTIONS)
Explore 12 Videos
- COMMUNICATION SYSTEMS Book:AAKASH SERIESChapter:COMMUNICATION SYSTEMS Exercise:PRACTICE EXERCISE (TV Antenna)
Explore 16 Videos
- CURRENT ELECTRICITYBook:AAKASH SERIESChapter:CURRENT ELECTRICITYExercise:EXERCISE - IA
Explore 2 Videos
Similar Questions
What is the current drawn from the battery of 6 V ?
View SolutionWhen current supplied by a cell to a circuit is 0.3A, its terminal potential difference is 0.9 A. When the current supplied becomes 0.2 A, its terminal potential difference becomes 1.0 V . The internal resistance of the cell is
View Solution
Knowledge Check
When a current of 2 A flows in a battery from negative to positive terminal, the potential difference across it is 12 V. If a current of 3 A flowing in the opposite direction produces a potential difference of 15 V, the emf of the battery is
A12.6VB13.2VC13.5VD14.0V
For a cell, the terminal potential difference is 2.2V, when circuit is open and reduces to 1.8V. When cell is connected to a resistance R=5Ω, the internal resistance of cell (R) is
View SolutionFor a cell, the terminal potential difference is 2.2V, when circuit is open and reduces to 1.8V. When cell is connected to a resistance R=5Ω, the internal resistance of cell (R) is
View SolutionThe potential difference between the terminals of a 6.0V battery is 7.2 V when it is being charged by a current of 2.0A.What is the internal resistance of the battery?
View SolutionFor a cell, the graph between the potential difference (V) across the terminals of the cells and the current I drawn from the cell is as shown in figure. Calculate the e.m.f. and the internal resistance of the cell.
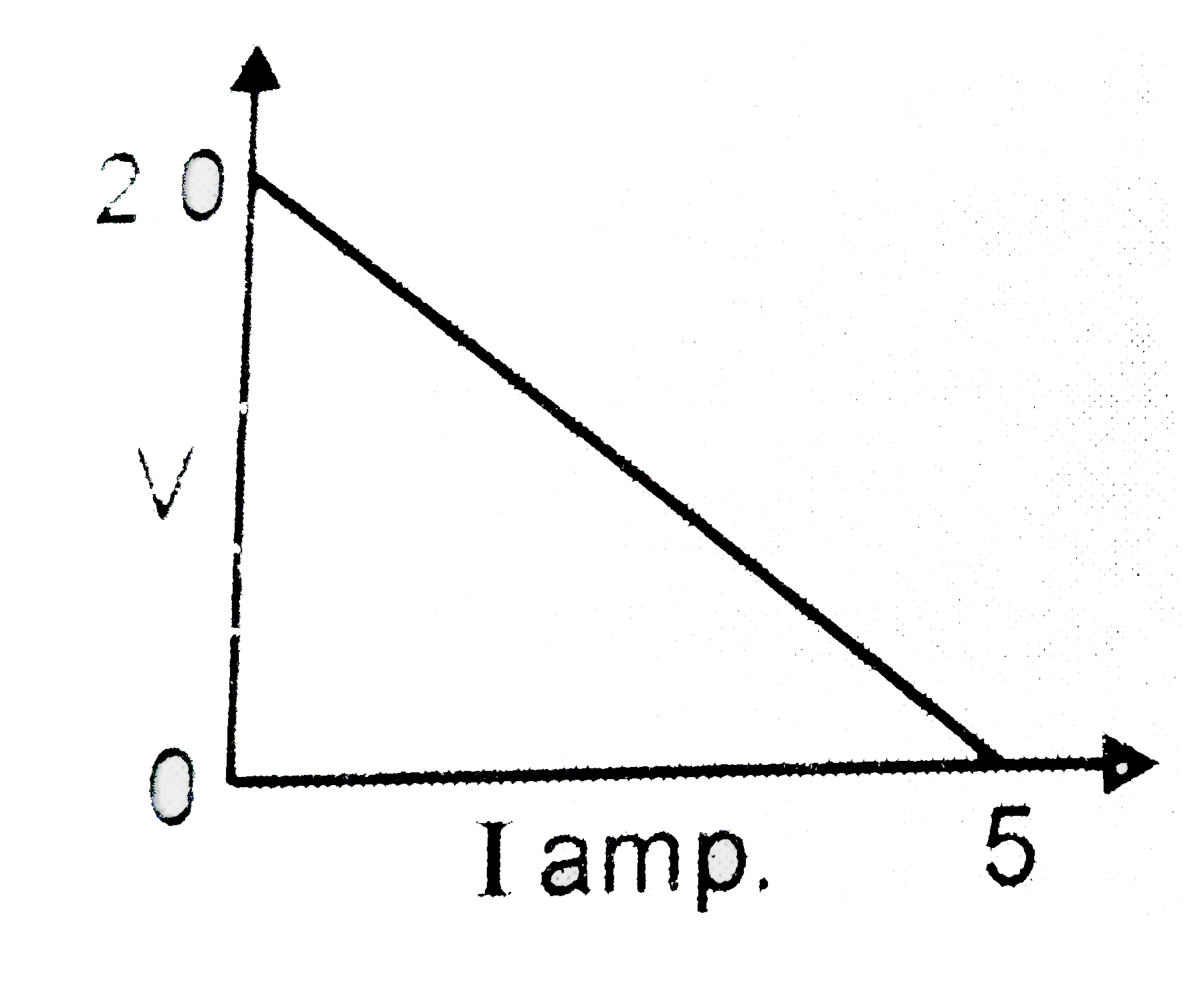
View SolutionA battery of e.m.f 3 V supplies current through a circuit in which the resistance can be changed. A high resistance voltmeter is connected across the battery. When the current is 1.5 A, the voltmeter reads 2.7 V. Find the internal resistance of the battery.
View SolutionThe potential difference across the terminals of a battery is 10 V when there is a current of 3 A in the battery from the negative to the positive terminal. When the current is 2A in the reverse direction, the potential difference becomes 15 V. The internal resistance of the battery is
View SolutionThe potential difference across the terminals of a battery is 8.4V when there is a current of 1.50A in the battery from the negative to the positive terminal. When the current is 3.50Air, the reverse direction, the potential difference becomes 9.4V.
(a) What is the internal resistance of the battery?
(b) What is the emf of the battery?
View Solution