Four charges Q, q, Q and q are placed at the corners A, B, C and D of a square ABCD. If the resultant electric force on the charge at the corner C is zero, find the value of Q/q.
The correct Answer is:−2√2
To solve the problem of finding the value of Qq such that the resultant electric force on the charge at corner C of square ABCD is zero, we can follow these steps:
Step 1: Understand the Configuration
We have four charges placed at the corners of a square ABCD:
- Charge Q at corner A
- Charge q at corner B
- Charge Q at corner C
- Charge q at corner D
Step 2: Identify Forces Acting on Charge at C
The charge at corner C experiences forces due to the charges at corners A, B, and D. We need to calculate these forces:
1. Force due to charge at A (F_AC)
2. Force due to charge at B (F_BC)
3. Force due to charge at D (F_DC)
Step 3: Calculate the Force F_AC
The distance between A and C is the diagonal of the square, which is √2a (where a is the side length of the square). The force FAC can be calculated using Coulomb's law:
FAC=kQ⋅q(√2a)2=kQ⋅q2a2
This force acts along the diagonal from A to C.
Step 4: Calculate the Force F_BC
The distance between B and C is a. The force FBC is:
FBC=kq⋅Qa2
This force acts horizontally towards B.
Step 5: Calculate the Force F_DC
The distance between D and C is also a. The force FDC is:
FDC=kq⋅Qa2
This force acts vertically towards D.
Step 6: Set Up the Force Balance Equation
For the resultant force on charge C to be zero, the sum of the forces acting on it must equal zero. The forces F_BC and F_DC are both repulsive and act at right angles to each other. Thus, we can express the balance of forces as:
FAC=FBC+FDC
Step 7: Substitute the Forces into the Equation
Substituting the expressions for the forces:
kQ⋅q2a2=kq⋅Qa2+kq⋅Qa2
This simplifies to:
kQ⋅q2a2=2kq⋅Qa2
Step 8: Cancel Common Terms
We can cancel k and Q from both sides (assuming Q≠0 and k≠0):
q2a2=2qa2
Step 9: Simplify the Equation
This leads to:
Q=4q
Step 10: Find the Ratio Qq
Thus, we find:
Qq=4
Final Answer
The value of Qq is 4.
---
We have four charges placed at the corners of a square ABCD:
- Charge Q at corner A
- Charge q at corner B
- Charge Q at corner C
- Charge q at corner D
The charge at corner C experiences forces due to the charges at corners A, B, and D. We need to calculate these forces:
1. Force due to charge at A (F_AC)
2. Force due to charge at B (F_BC)
3. Force due to charge at D (F_DC)
The distance between A and C is the diagonal of the square, which is √2a (where a is the side length of the square). The force FAC can be calculated using Coulomb's law:
FAC=kQ⋅q(√2a)2=kQ⋅q2a2
This force acts along the diagonal from A to C.
The distance between B and C is a. The force FBC is:
FBC=kq⋅Qa2
This force acts horizontally towards B.
The distance between D and C is also a. The force FDC is:
FDC=kq⋅Qa2
This force acts vertically towards D.
For the resultant force on charge C to be zero, the sum of the forces acting on it must equal zero. The forces F_BC and F_DC are both repulsive and act at right angles to each other. Thus, we can express the balance of forces as:
FAC=FBC+FDC
Substituting the expressions for the forces:
kQ⋅q2a2=kq⋅Qa2+kq⋅Qa2
This simplifies to:
kQ⋅q2a2=2kq⋅Qa2
We can cancel k and Q from both sides (assuming Q≠0 and k≠0):
q2a2=2qa2
This leads to:
Q=4q
Thus, we find:
Qq=4
The value of Qq is 4.
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBook:AAKASH SERIESChapter:ELECTRIC FIELD AND POTENTIALExercise:PROBLEMS (LEVEL-I)
Explore 13 Videos
- ELECTRIC CHARGES AND FIELDSBook:AAKASH SERIESChapter:ELECTRIC CHARGES AND FIELDSExercise:Practice Exercise
Explore 57 Videos
- ELECTROMAGNETIC INDUCTIONBook:AAKASH SERIESChapter:ELECTROMAGNETIC INDUCTIONExercise:Additional Exercise
Explore 11 Videos
Similar Questions
Four charges of +q, +q +q and +q are placed at the corners A, B, C and D of s square. The resultant force on the charge at D
View SolutionFour charges of +q, +q +q and +q are placed at the corners A, B, C and D of s square. The resultant force on the charge at D
View SolutionEqual charges Q are placed at the four corners A, B, C, D of a square of length a. The magnitude of the force on the charge at B will be
View SolutionFour point charge q,−q,2Q and Q are placed in order at the corners A, B, C and D of a square. If the field at the midpoint of CD is zero then the value of q/Q is 5√5x. Find the value of x.
View SolutionThree charges +q, -q and +q are kept at the corners of an equilateral triangle of side d. Find the resultant electric force on a charge +q placed at the centroid O of the triangle.
View SolutionCharges q,2q,3q and 4q are placed at the corners A,B,C and D of a square as shown in the following figure. The directon of electric field at the centre of the square is along

View SolutionCharges q,2q,3q and 4q are placed at the corners A,B,C and D of a square as shown in the following figure. The directon of electric field at the centre of the square is along

View SolutionFour point charges Q, q, Q and q are placed at the corners of a square of side' a' as shown in the figure.
Find the .
(a) resultnat electric force on a charge Q and .
(b) potential energy of this system.
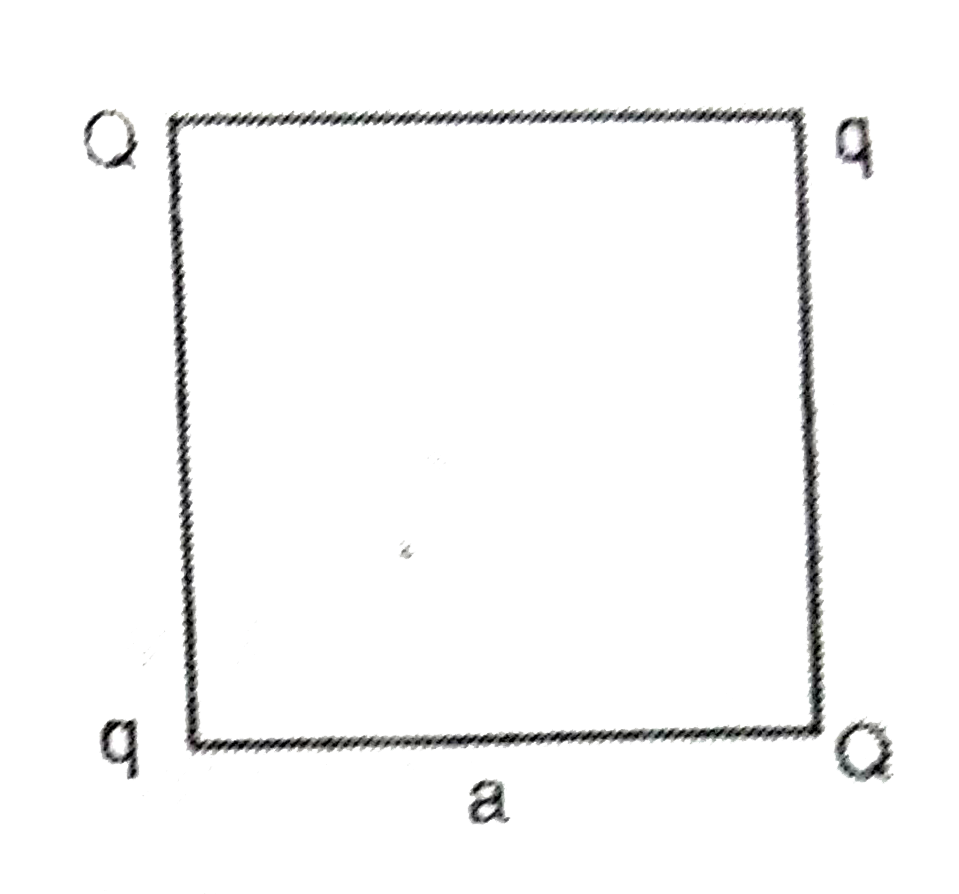
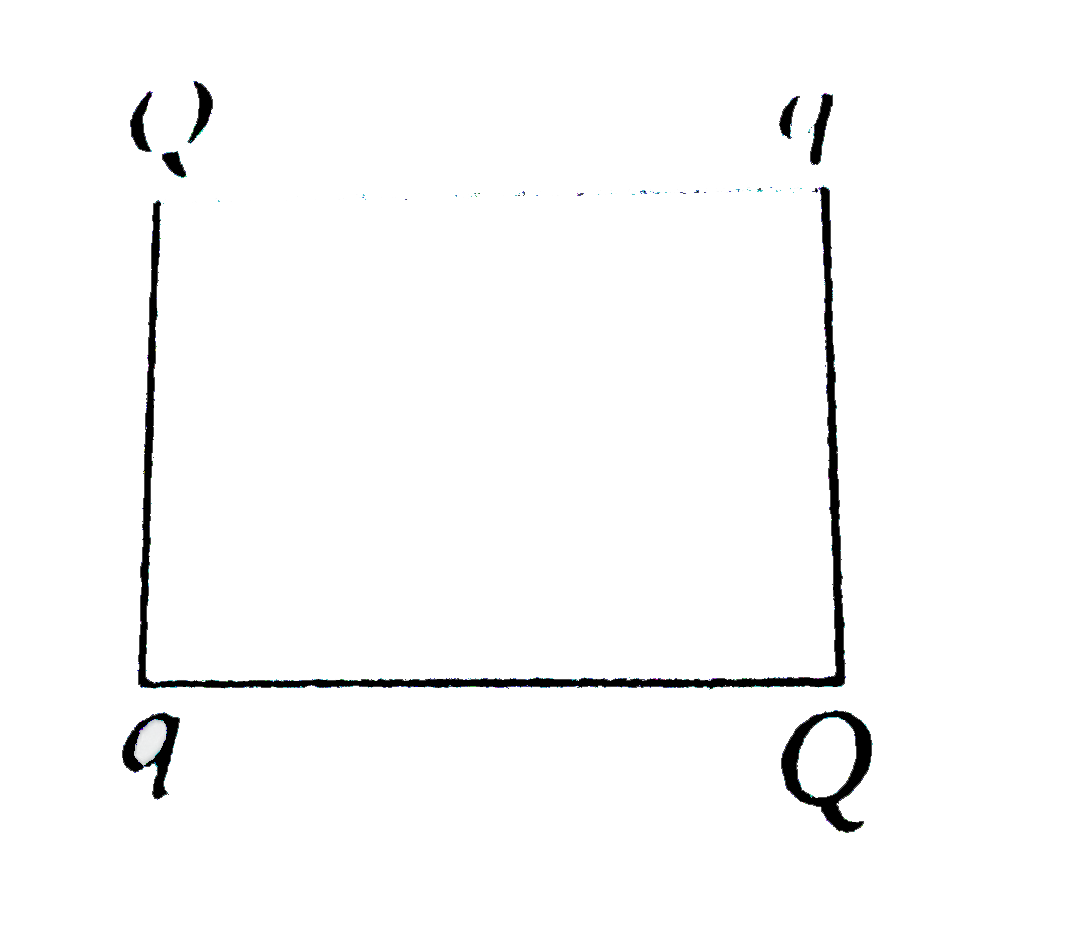
View SolutionFour charge Q,q,Q and q are kept at the four corners of a square as shown below. What is the relation between Q and q, so that the net force on a charge q is zero ?
View SolutionFour identical charges q are placed at the corners of a square of side a. Find the potential energy of one of the charges due to the remaining charges.
 .
.
View Solution