A long straight conductor carrying a current of 4 A is in parallel to another conductor of length 5 cm and carrying a current 9 A. They are separated by a distance of 10 cm. Calculate (a) B due to first conductor at second conductor (b) The force on the short conductor.
To solve the problem, we will break it down into two parts as requested:
Part (a): Calculate the Magnetic Field (B) due to the first conductor at the location of the second conductor.
1. Identify the given values:
- Current in the first conductor, I1=4A
- Current in the second conductor, I2=9A
- Length of the second conductor, l=5cm=0.05m
- Distance between the two conductors, d=10cm=0.1m
2. Use the formula for the magnetic field (B) due to a long straight conductor:
B=μ0I2πd
where μ0=4π×10−7T m/A.
3. Substituting the values into the formula:
B1=4π×10−7T m/A⋅4A2π⋅0.1m
4. Simplifying the expression:
- The π cancels out:
B1=4×10−7⋅42⋅0.1
B1=16×10−70.2=80×10−7=8×10−6T
5. Final result for part (a):
B1=8×10−6T
Part (b): Calculate the force on the short conductor.
1. Use the formula for the magnetic force (F) on a current-carrying conductor:
F=I⋅l⋅B⋅sin(θ)
where θ=90∘ (since the current and magnetic field are perpendicular).
2. Substituting the known values:
- I2=9A
- l=0.05m
- B1=8×10−6T
- sin(90∘)=1
Thus, the force can be calculated as:
F=9A⋅0.05m⋅8×10−6T⋅1
3. Calculating the force:
F=9⋅0.05⋅8×10−6
F=0.36×10−5N=3.6×10−6N
4. Final result for part (b):
F=3.6×10−6N
Summary of Results:
- (a) The magnetic field B due to the first conductor at the location of the second conductor is 8×10−6T.
- (b) The force on the short conductor is 3.6×10−6N.
- Current in the first conductor, I1=4A
- Current in the second conductor, I2=9A
- Length of the second conductor, l=5cm=0.05m
- Distance between the two conductors, d=10cm=0.1m
B=μ0I2πd
where μ0=4π×10−7T m/A.
B1=4π×10−7T m/A⋅4A2π⋅0.1m
- The π cancels out:
B1=4×10−7⋅42⋅0.1
B1=16×10−70.2=80×10−7=8×10−6T
B1=8×10−6T
F=I⋅l⋅B⋅sin(θ)
where θ=90∘ (since the current and magnetic field are perpendicular).
- I2=9A
- l=0.05m
- B1=8×10−6T
- sin(90∘)=1
F=9A⋅0.05m⋅8×10−6T⋅1
F=9⋅0.05⋅8×10−6
F=0.36×10−5N=3.6×10−6N
F=3.6×10−6N
- (a) The magnetic field B due to the first conductor at the location of the second conductor is 8×10−6T.
- (b) The force on the short conductor is 3.6×10−6N.
Topper's Solved these Questions
- ELECTROMAGNETICSBook:AAKASH SERIESChapter:ELECTROMAGNETICSExercise:PROBLEMS (LEVEL -II)
Explore 40 Videos
- ELECTROMAGNETICSBook:AAKASH SERIESChapter:ELECTROMAGNETICSExercise:ADDITIONAL EXERCISE
Explore 13 Videos
- ELECTROMAGNETICSBook:AAKASH SERIESChapter:ELECTROMAGNETICSExercise:EXERCISE (VERY SHORT ANSWER QUESTIONS )
Explore 19 Videos
- ELECTROMAGNETIC WAVESBook:AAKASH SERIESChapter:ELECTROMAGNETIC WAVESExercise:EXERCISE -II
Explore 22 Videos
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
Explore 5 Videos
Similar Questions
A Long straight conductor carrying a current of 2 A is in parallel to another conductor of length 5 cm. and carrying a current 3A. They are separated by a distance of 10 cm. Calculate (a) B due to first conductor at second conductor (b) the force on the short conductor.
View SolutionA conductor PQ, carrying a current i is placed perpendicular to a long conductor xy carrying a current i. The direction of force on PQ will be

View Solution
Knowledge Check
A conductor of length 2 m carrying current 2 A is held parallel to an infinitely long conductor carrying current of 12 A at a distance of 100 mm, the force on small conductor is
A8.6×10−5NB6.6×10−5NC7.6×10−5ND9.6×10−5N
Find the magnetic induction due to a straight conductor of length 16cm carrying current of 5A at a distance of 6cm from the midpoint of conductor
View SolutionA square loop ABCD , carrying a current I, is placed near and coplanar with a long straight conductor XY carrying a current I.

View SolutionA conductor AB of length l carrying current i is placed perpendicular to a long straight conductor carrying a current I as shown. Force on AB will be
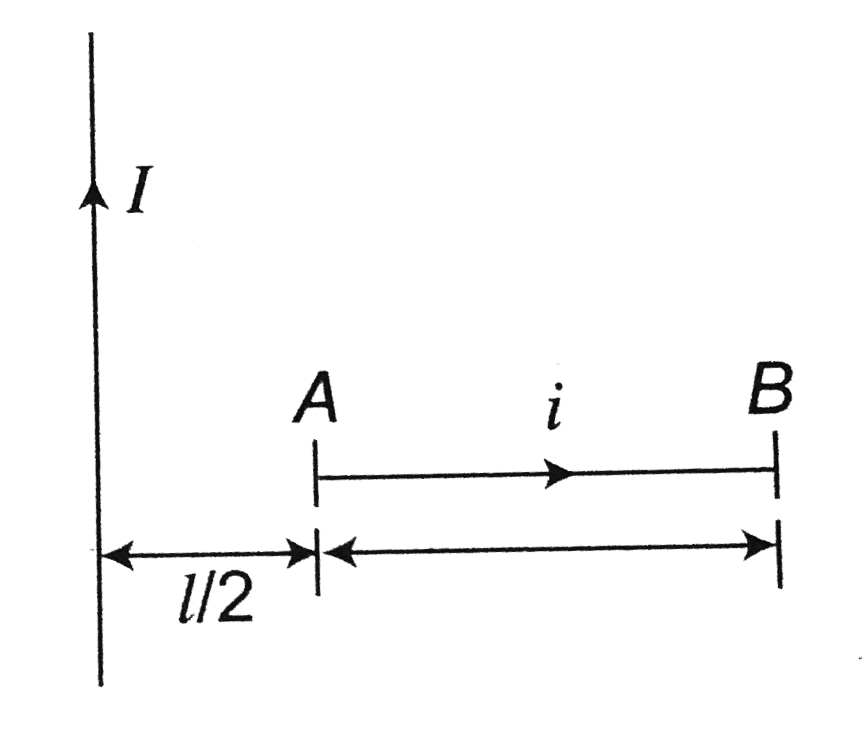
View SolutionAB and CD are long straight conductor, distance d apart, carrying a current l. The magnetic field at the midpoint of BC is

View SolutionA long straight vertical conductor carries a current of 8A in the upward direction. What the magnitude of the resultant magnetic induction at a point in the horizonatal plane at a distance of 4 cm from the conductor towards South? (The horizontal compo-nent of earth's magnetic induction =4×10−5T)
View SolutionA large straight current carrying conductor is bent in the form of Lshape. Find B at P
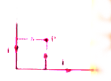
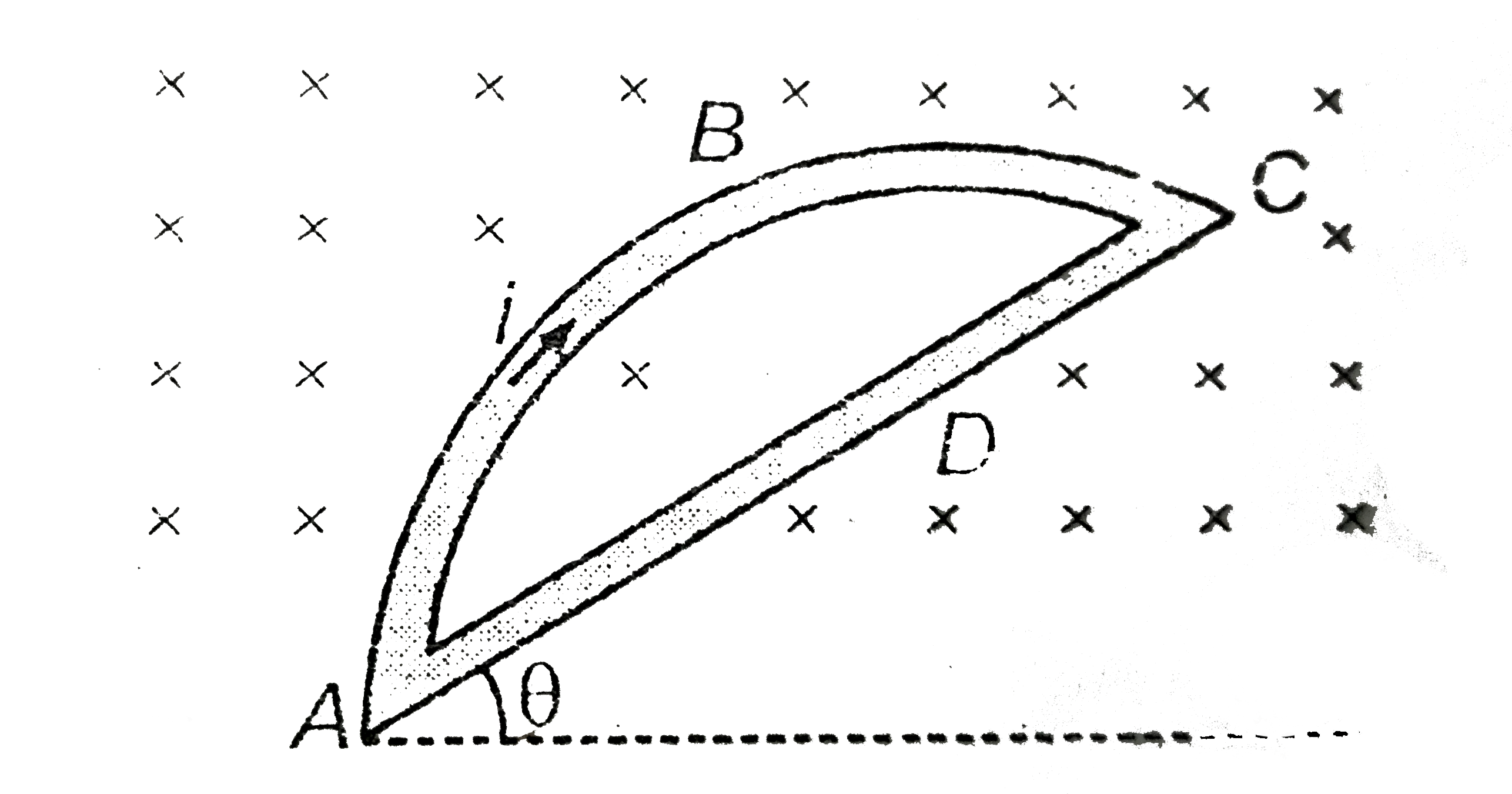
View SolutionA circular wire ABC and a straight conductor ADC are carrying current i and are kept in the magnetic field B then
View Solution
