A large straight current carrying conductor is bent in the form of Lshape. Find B at P
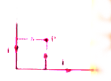
Let us divide the conductor into two semi infinite segments 1 and 2.
Then, induction at P is
→B=→B1+→B2
→B1=μ0i4πa(sin(90−θ1)+sin90)ˆk ……(ii)
→B2=μ0i4πa(sin(90−θ2)+sin90)ˆk ….(iii)
then →B=μ0i4πa(cosθ1+cosθ2+2)ˆk,
where cosθ1=cosθ2=1√2
Hence, →B=(2+√2)μ0iˆk4πa
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-IA
142 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-IB
71 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- NUCLEAR PHYSICSBOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE PRACTICE SHEET (ADVANCED) Integer Type Questions
3 Videos
Similar Questions
A long straight current carrying conductor has a current from -z to +z, then find the magnetic induction at a point P(x, y, 0) in vectorial notation (ˆi,ˆj,ˆk) are unit vector along x,y, z axis)

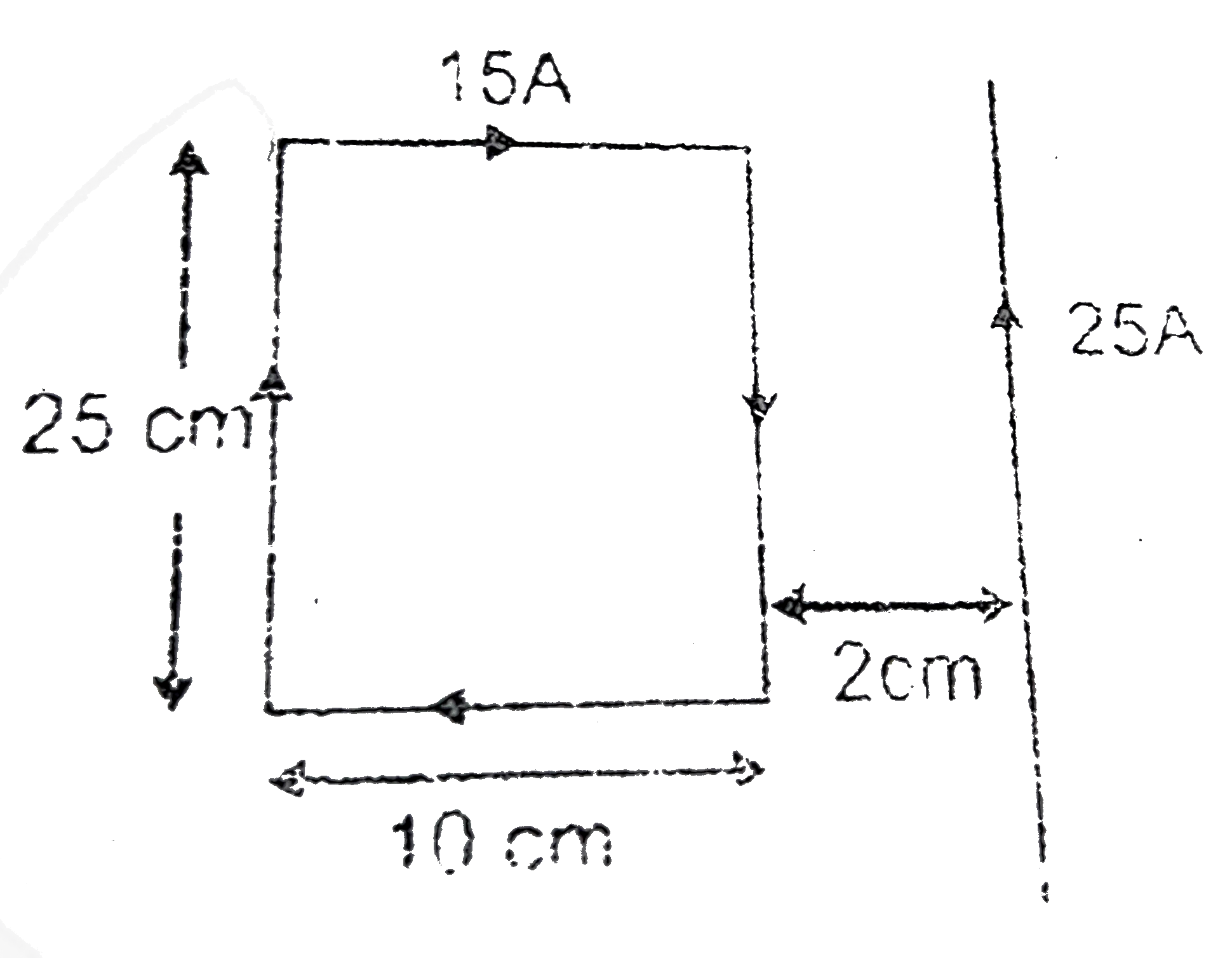
View SolutionFigure shows a rectangular current carrying loop placed 2cm away from a long, straight, current-carrying conductor. What is the direction of the net force acting on the loop?
View SolutionThe magnetic field in a straight current carrying conductor wire is:
View SolutionThe magnetic flux density at a point distant d from a long straight current carrying conductor is B, then its value at distance d2 will be-
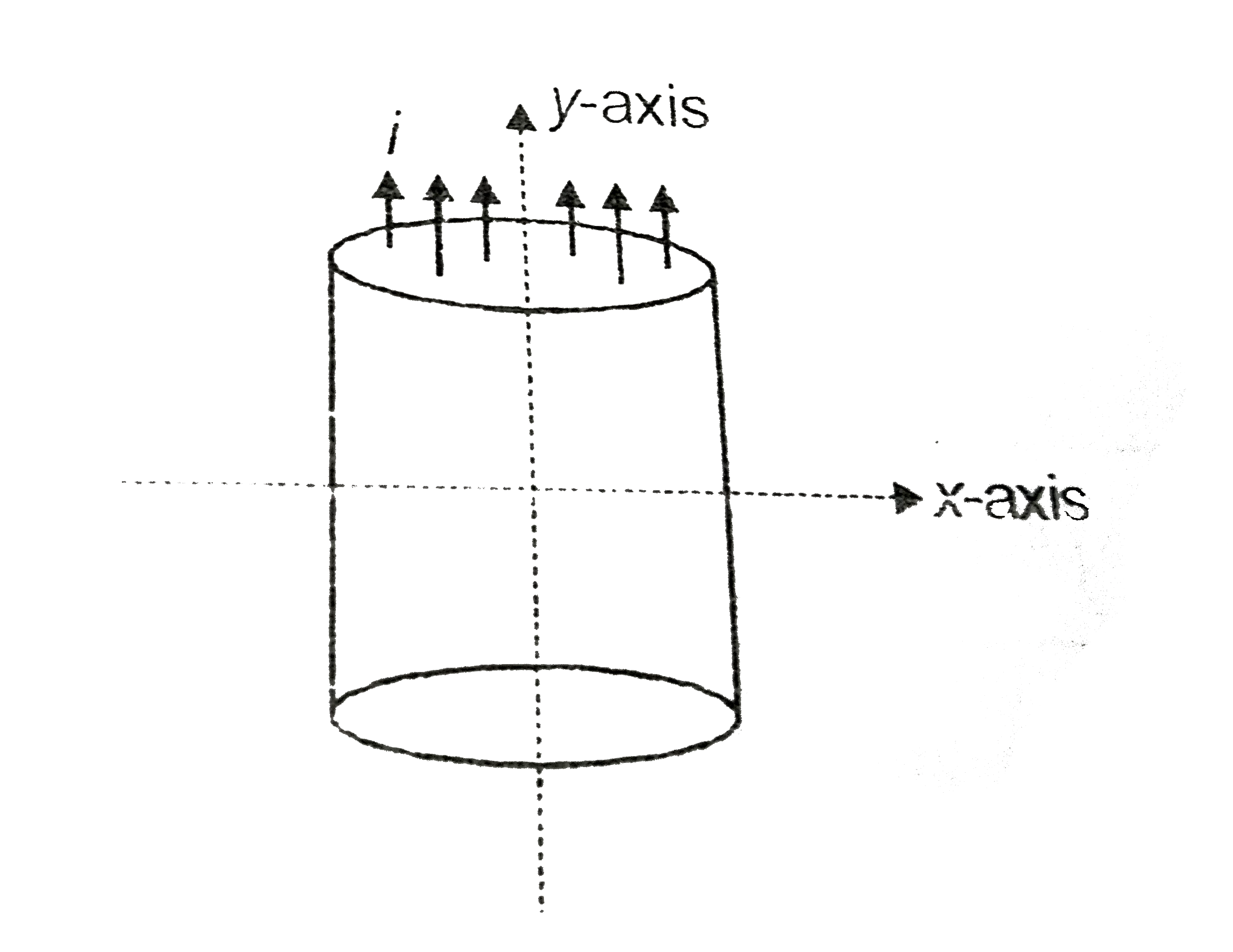
View SolutionThe figure shows a long straight current carrying conductor. Can you plot the variation of magnetic field on x-axis ?
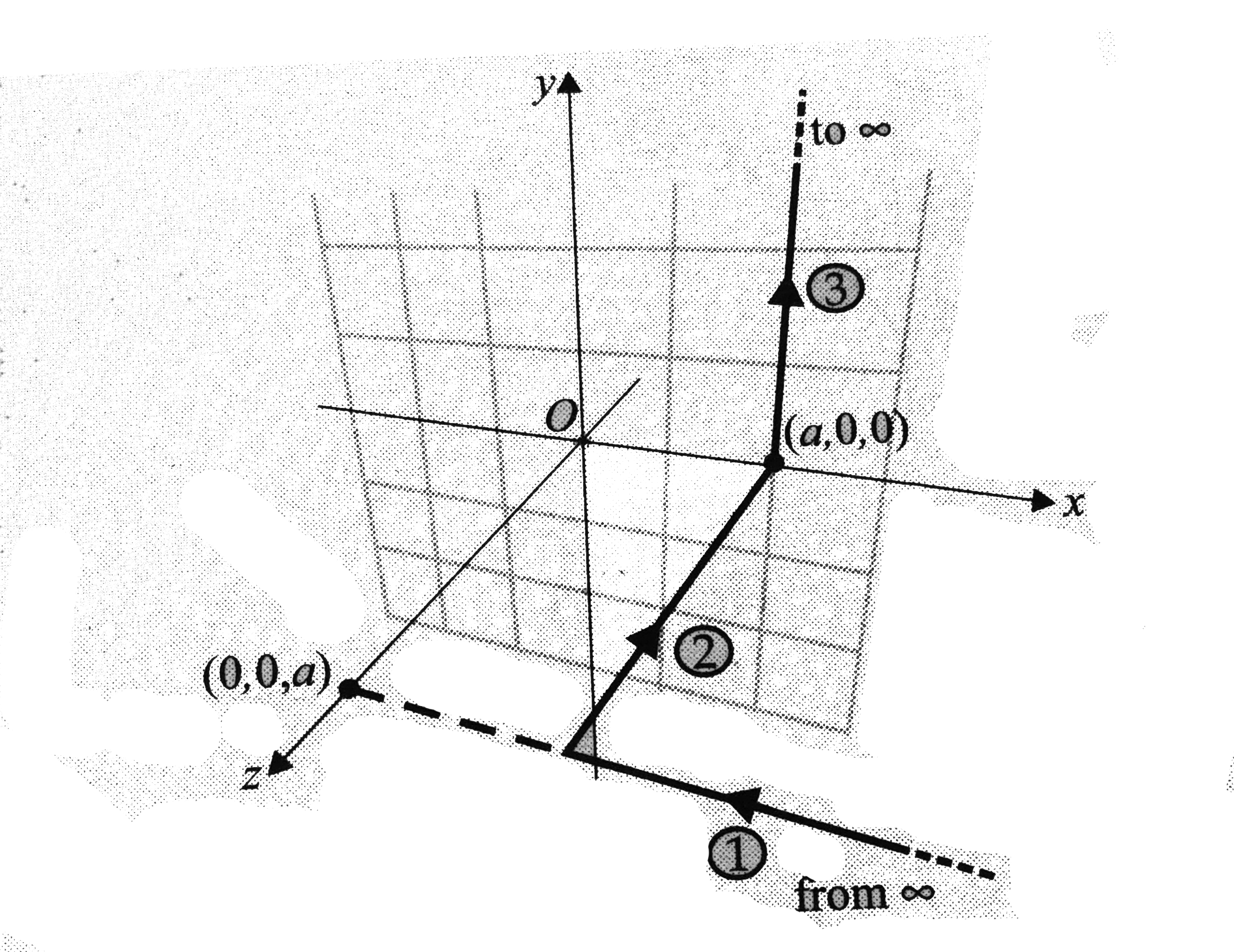
View SolutionAn infinite current carrying conductor is bent into three segment (1), (2) and (3) as shown in Fig. If it carries a current i, find the magnetic induction at the origin.
View SolutionA current carrying conductor is in the form of a sine curve as shown, which carries current I. If the equation of this curve is Y=2sin(πxL) and a uniform magnetic field B exists in space.

View SolutionDerive the expression for force per unit length between two long straight parallel current carrying conductors. Hence define one ampere.
View SolutionA circular loop is placed near a current carrying conductor as shown in figure. Find the direction of induced current, if the current, in the wire is decreasing.

View SolutionIf a straight conductor of length 40cm bent in the form of a square and the current 2A is allowed to pass through square, then find the magnetic induction at the centre of the square loop
View Solution
