Diatomic molecules like hydrogen have energies due to both translations as well as rotational motion. From the equation in kinetic theory pV=23E,E is
the total energy per unit volume
only the translational part of energy because rotational energy is very small compared to the translational energy
only the translational part of the energy because during collisions with the wall pressure rotates to change in linear momentum
the translational part of the energy because rotational energies of molecules can be of either sign and its average over all the molecules is zero.
The correct Answer is:C
Step by step video solution for Diatomic molecules like hydrogen have energies due to both translations as well as rotational motion. From the equation in kinetic theory pV =2/3 E,E " is " by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
For a diatomic gas having 3 translational and 2 rotational degree of freedom ,the energy is given by ?
View SolutionThe key feature of Bohr's spectrum of hydrogen atom is the quantization of angular momentum when an electron is revolving around a proton. We will extend this to a general rotational motion to find quantized rotational energy of a diatomic molecule assuming it to be rigid.The rule to be applied is Bohr's quantization condition.
it is found that the excitation from ground to the first excited state of rotation for the CO molecule is close to 4π×1011Hz then the moment of inertia of CO molecule about its center of mass is close to
(Takeh=2π×10−34Js)
View SolutionThe key feature of Bohr's spectrum of hydrogen atom is the quantization of angular momentum when an electron is revolving around a proton. We will extend this to a general rotational motion to find quantized rotational energy of a diatomic molecule assuming it to be rigid.The rule to be applied is Bohr's quantization condition.
In a CO molecule, the distance between C(mass=12a.m.u)andO(mass=16a.m.u) where 1a.m.u=53×10−27kg,is close to
View SolutionThe key feature of Bohr's spectrum of hydrogen atom is the quantization of angular momentum when an electron is revolving around a proton. We will extend this to a general rotational motion to find quantized rotational energy of a diatomic molecule assuming it to be rigid.The rule to be applied is Bohr's quantization condition.
In a CO molecule, the distance between C(mass=12a.m.u)andO(mass=16a.m.u) where 1a.m.u=53×10−27kg,is close to
View SolutionAt ordinary temperatures, the molecules of an ideal gas have only translational and rotational kinetic energies. At high temperatures they may also have vibrational energy.
As a result of this, at higher temperature
View SolutionAt ordinary temperatures, the molecules of an ideal gas have only translational and rotational kinetic energies. At high temperatures they may also have vibrational energy.
As a result of this, at higher temperature
View SolutionRotational energy levels of diatomic molecules are well described by the formula EJ=BJ(J+1), where J is the rotational quantum number of the molecule and B its rotational constant. B is related to the reduced
mass μ and the bond length R of the molecule through the equation B=h28π2μR2.
In general, spectroscopic transitions appear at photon energies which are equal to the energy difference between appropriate states of a molecule (hv=ΔE). The observed rotational transitions occur between adjacent rotational levels, hence ΔE=EJ+1−EJ=2B(J+1). Consequently, successive rotational transitions that appear on the spectrum (such as the one shown here) follow the equation h(Δυ)=2B.
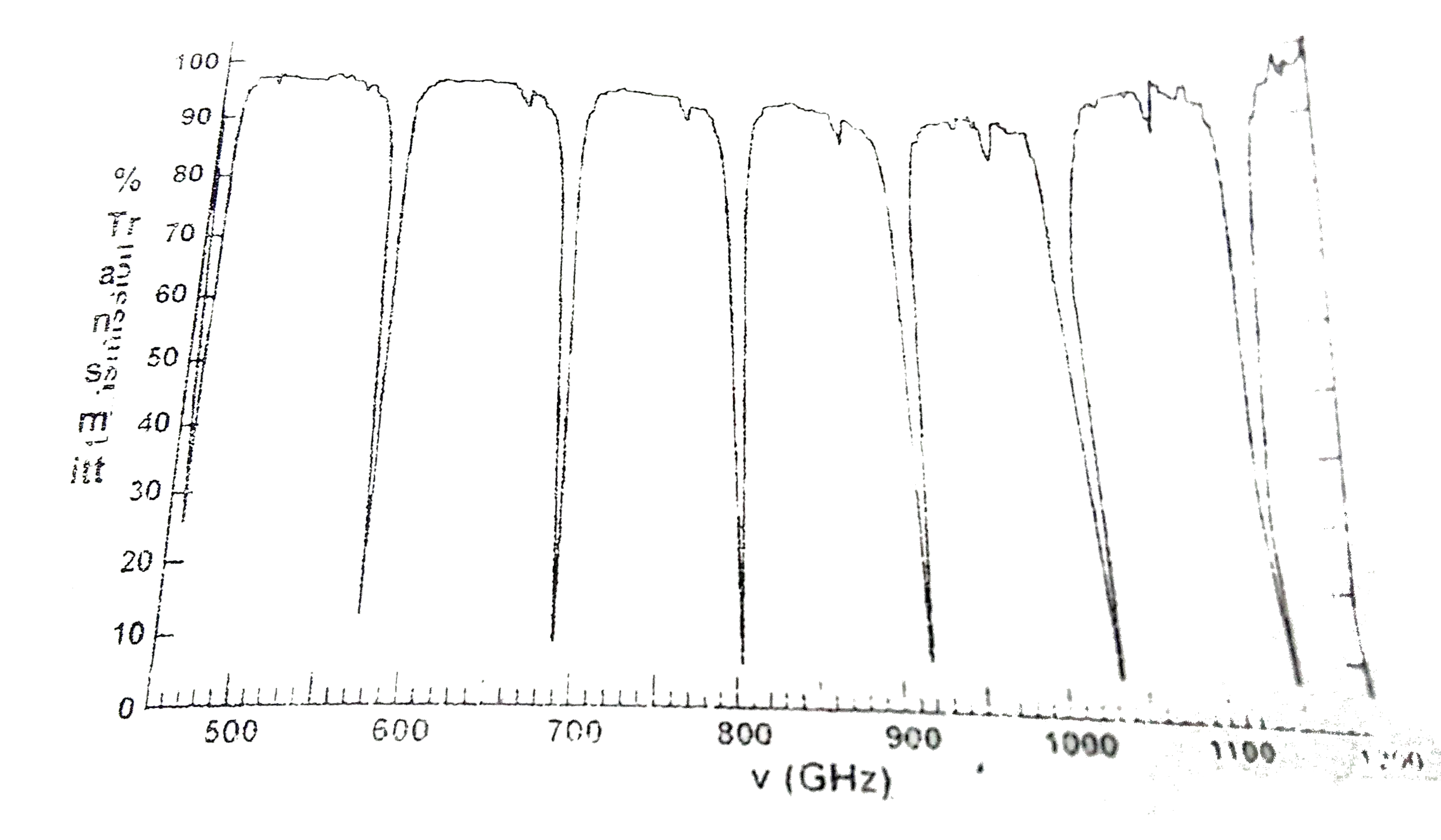
By inspecting the spectrum provided, determine the following quantities for ^(2)CpC with appropriate units
(a) Δυ (b) B (c) R
View SolutionAt ordinary temperatures, the molecules of an ideal gas have only translational and rotational kinetic energies. At high temperatures, they may also have vibrational energy. As a result of this, at higher temperatures, molar specific heat capacity at constant volume, CV is
View SolutionCalculate the dimensional formula of energy from the equation E=12mv2.
View SolutionA vessel contains a mixture of 2 moles o hydrogen and 3 moles of oxygen at a constant temperature. The ratio of average translational kinetic energy per H2 molecule to that per O2 molecule is
View Solution
