A thin equiconvex lens of glass(μ=1.5) with radius of curvature 4m is placed on a horizontal plane mirror. When the space between the lens and mirror is filled with a liquid, an object held at a distance 6m vertically above the lens is found to coincide with its own image. What is the refractive index of the liquid.
To solve the problem step by step, we will follow the concepts of optics, particularly focusing on lenses and their properties.
1. Understanding the Setup:
We have a thin equiconvex lens made of glass with a refractive index (μ) of 1.5 and a radius of curvature (R) of 4 m. The lens is placed on a horizontal plane mirror. An object is positioned 6 m vertically above the lens.
2. Coincidence of Object and Image:
The problem states that the object coincides with its own image. This means that the light rays from the object must retrace their path after passing through the lens and reflecting off the mirror.
3. Using the Lens Maker's Formula:
The focal length (f) of the lens can be calculated using the lens maker's formula:
1f=(μ−1)(1R1−1R2)
For an equiconvex lens, R1=R and R2=−R. Thus, we have:
1f=(1.5−1)(14−(−14))=0.5(14+14)=0.5(24)=0.5⋅12=14
Therefore, the focal length f is:
f=4 m
4. Finding the Equivalent Focal Length:
Since the object coincides with its image, the distance from the lens to the object (D) is equal to the focal length (f) of the system. Thus:
D=6 m
The equivalent focal length of the system when filled with a liquid can be expressed as:
1feq=1fglass+1fliquid
Since the image coincides with the object, we have feq=D=6 m.
5. Setting Up the Equation:
We know:
16=14+1fliquid
Rearranging gives:
1fliquid=16−14
Finding a common denominator (12):
1fliquid=212−312=−112
Thus, fliquid=−12 m.
6. Finding the Refractive Index of the Liquid:
The focal length of the liquid can also be expressed using its refractive index (μ_liquid):
1fliquid=(μliquid−1)(1R)
Substituting R=4 m:
−112=(μliquid−1)(14)
Solving for μ_liquid:
μliquid−1=−112⋅4=−13
Thus:
μliquid=1−13=23
Final Answer:
The refractive index of the liquid is 43.
We have a thin equiconvex lens made of glass with a refractive index (μ) of 1.5 and a radius of curvature (R) of 4 m. The lens is placed on a horizontal plane mirror. An object is positioned 6 m vertically above the lens.
The problem states that the object coincides with its own image. This means that the light rays from the object must retrace their path after passing through the lens and reflecting off the mirror.
The focal length (f) of the lens can be calculated using the lens maker's formula:
1f=(μ−1)(1R1−1R2)
For an equiconvex lens, R1=R and R2=−R. Thus, we have:
1f=(1.5−1)(14−(−14))=0.5(14+14)=0.5(24)=0.5⋅12=14
Therefore, the focal length f is:
f=4 m
Since the object coincides with its image, the distance from the lens to the object (D) is equal to the focal length (f) of the system. Thus:
D=6 m
The equivalent focal length of the system when filled with a liquid can be expressed as:
1feq=1fglass+1fliquid
Since the image coincides with the object, we have feq=D=6 m.
We know:
16=14+1fliquid
Rearranging gives:
1fliquid=16−14
Finding a common denominator (12):
1fliquid=212−312=−112
Thus, fliquid=−12 m.
The focal length of the liquid can also be expressed using its refractive index (μ_liquid):
1fliquid=(μliquid−1)(1R)
Substituting R=4 m:
−112=(μliquid−1)(14)
Solving for μ_liquid:
μliquid−1=−112⋅4=−13
Thus:
μliquid=1−13=23
The refractive index of the liquid is 43.
Topper's Solved these Questions
- RAY OPTICSBOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-I)
57 Videos
- PRACTICAL PHYSICSBOOK - AAKASH SERIESCHAPTER - PRACTICAL PHYSICSEXERCISE - LECTURE SHEET LEVEL-II (ADVANCED) Linked Comprehension Type Questions
4 Videos
- SEMICONDUCTOR DEVICESBOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE - III
3 Videos
Similar Questions
On a horizontal plane mirror, a thin equiconvex lens of glass is placed and when the space between the lens and mirror is filled with a liquid, an object held at a distance D which is 30cm vertically above the lens is found to coincide with its own image as shown in Figure. If equiconvex lens of glass has refractive index μ=1.5 and radius of curvature R=20cm then find refractive index of the liquid.

View SolutionA thin equiconvex lens of refractive index 3/2 is placed on a horizontal plane mirror as shown in figure. The space between the lens and the mirror is filled with a liquid of refractive index 4/3 . It is found that when a point object is placed 15 cm above the lens on its priincipal axis, the object coincides with its own image.
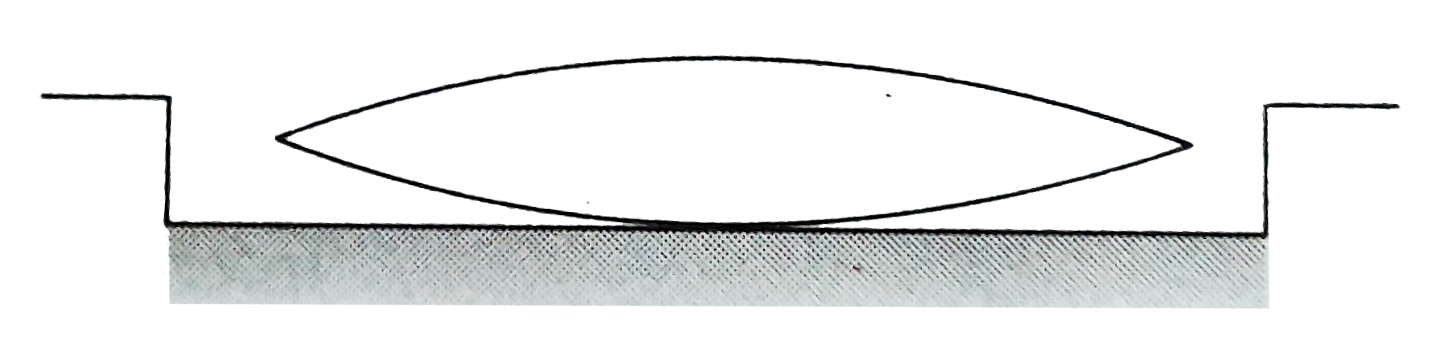
Q. If another liquid is filled instead of water, the object and the image coincide at a distance 25 cm from the lens.
Calculate the refractive index of the liquid.
View SolutionA thin equiconvex lens of refractive index 3/2 is placed on a horizontal plane mirror as shown in figure. The space between the lens and the mirror is filled with a liquid of refractive index 4/3 . It is found that when a point object is placed 15 cm above the lens on its priincipal axis, the object coincides with its own image.
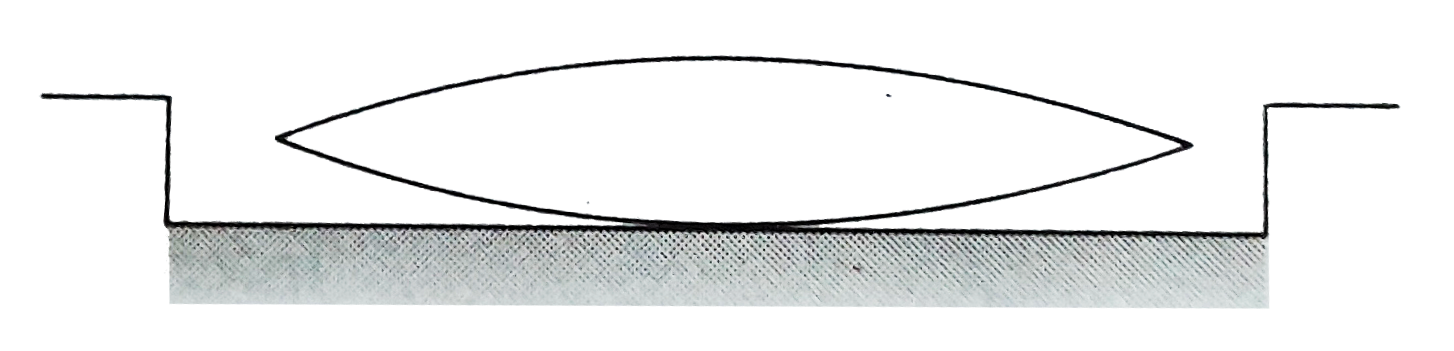
Q. The radius of curvature of the convex surface is
View SolutionBehind a thin converging lens having both the surfaces of the same radius 10cm, a plane mirror has been placed. The image of an object at a distance of 40 cm from the lens is formed at the same position. What is the refractive index of the lens?
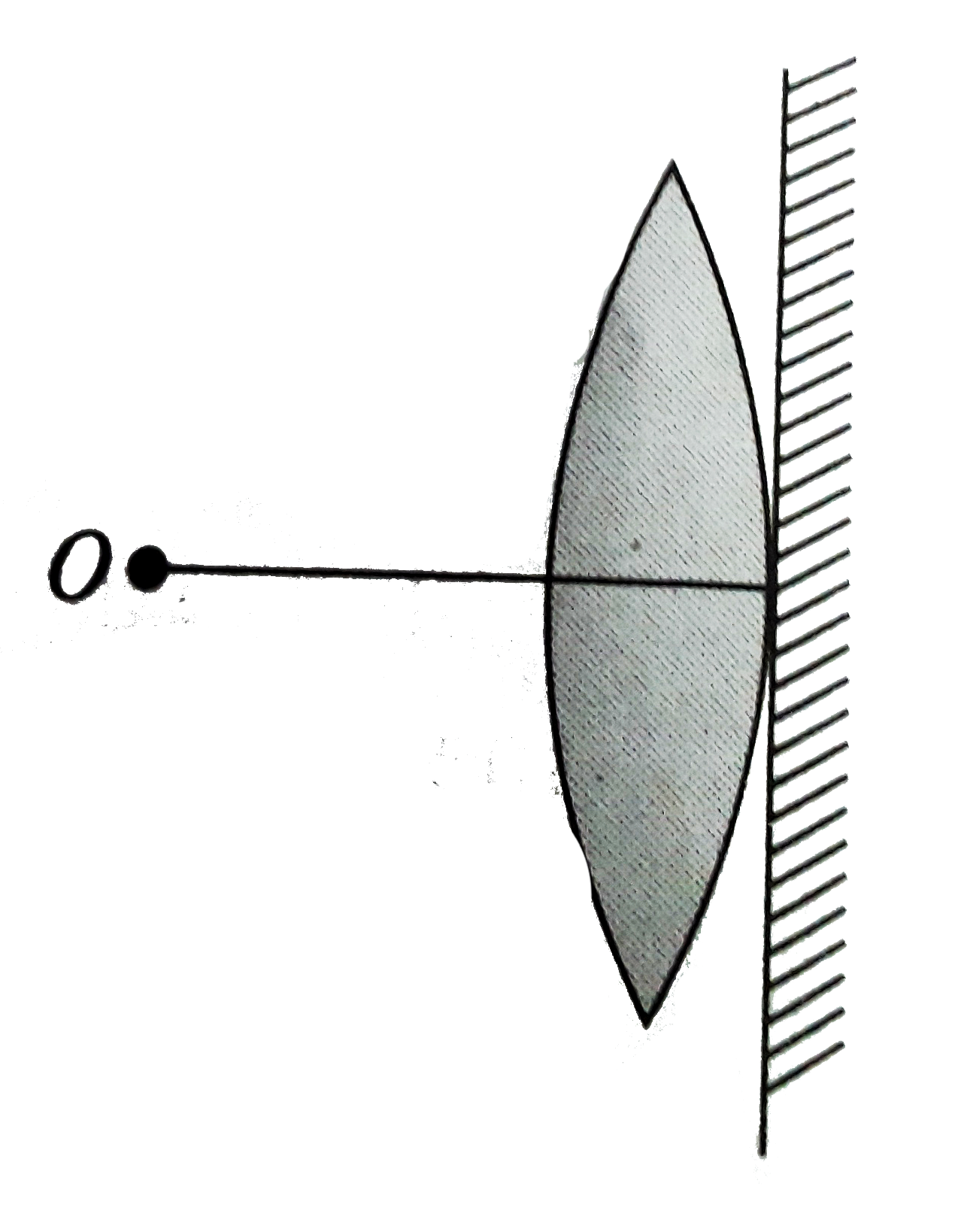
View SolutionA biconvex lens of focal length 15cm is in front of a plane mirror. The distance between the lens and the mirror is 10cm. A small object is kept at distance of 30cm from the lens. The final image is
View SolutionA plano-concave lens is made of glass of refractive index 1.5 and the radius of curvature of its curved face is 100 cm. What is the power of the lens?
View SolutionA thin bi-convex lens made up of glass of refractive index 3/2 is placed is front of a plane mirror. The space between the lens and the mirror is filled with water of refractive index 4/3 . The radii of curvatures of the lens are R1=15cm and R2=25cm . A point object is placed at distance x from the surface whose curvature is R1. The distance x is greater than the separation between the lens and the mirror. Find the value of x so that image must coincide with the object.
View SolutionAn equiconvex lens is made from glass of refractive index 1.5. If the radius of each surface is changed from 5cm to 6cm, then the power
View SolutionA thin equiconvex glass lens (μ1=1.5) of radius of curvature 'R' and a thin plano-concave lens (μ2=1.4) are kept in contact and the plane surface is silvered. This lens system is placed in air. When a point object is placed at a distance 20cm from the lens system on its principal axis, the image formed by the lens system coincides with the object. Then the value of radius of curvature 'R' is

View SolutionIn figure , L is half part of an equiconvex glass lens (μ=1.5) whose surfaces have radius of curvature R =40cm and its right surface is silvered . Normal to its principal axis a plane mirror M is placed on right of the lens . Distance between lens L and mirror M is b. A small object O is placed on left of the lens such that there is no parallax between final images formed by the lens and mirror. If transverse length of final image formed by lens is twice that of image formed by the mirror , calculate distance'a' in cm between lens and object.
_S01_072_Q01.png)
View Solution
