A cubical block of ice of mass m and edge L is placed in a large tray of mass M. If the ice melts, how far does the centre of mass of the system ''ice plus tray'' come down?
mL2(M+m)
(M−m)L2(M+m)
(M+2m)L2(M+m)
2ML(M+m)
Step by step video solution for A cubical block of ice of mass m and edge L is placed in a large tray of mass M. If the ice melts, how far does the centre of mass of the system ''ice plus tray'' come down? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Consider a gravity free hall in which a tray of mas M, carrying a cubical block of ice of mass m and edge L, is at rest in the middle.. If the ice melts, by what distance does the centre of mass of the tray plus the ice system descend?
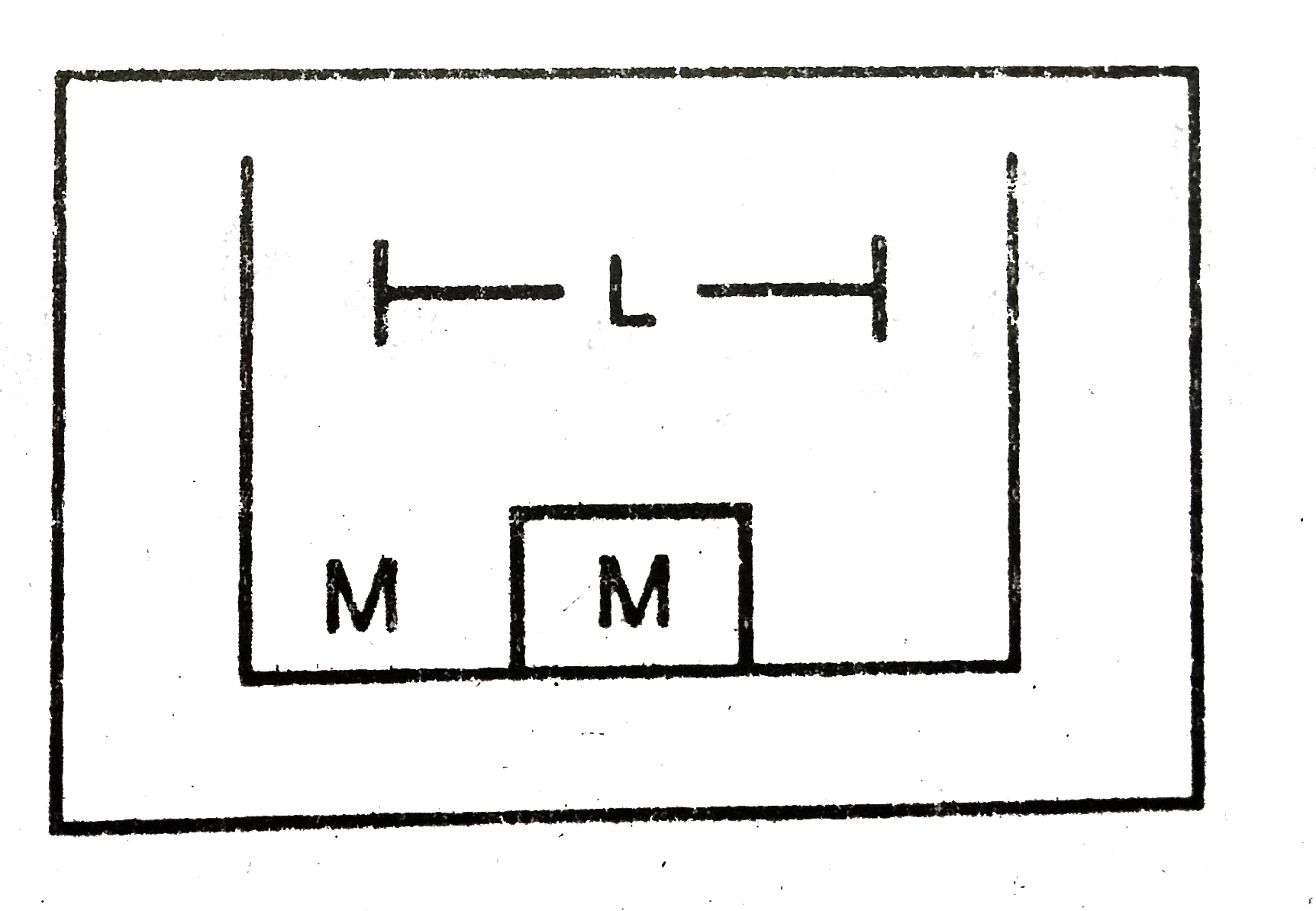
View SolutionA bullet of mass m and velocity v is fired into a large block of mass M. The final velocity of the system is
View SolutionA block of mass m slides over a smooth wedge of mass M which is placed over a rough horizontal surface . The centre of mass of the system will move towards left

Here μ = coefficient of friction between the wedge and the ground .
View SolutionFour point masses are placed at the corners of a square of side 2 m as shown in the figure. Find the centre of mass of the system w.r.t. the centre of square.

View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

View SolutionA block of mass m is placed on a smooth wedge of mass M, as shown in the figure . Just after placiing block m over wedge M.
View SolutionA cubical block of mass M and edge a slides down a rougg inclined plane of inclination θ with a uniform velocity. The torque of the normal force on the block about its centre has magnitude.
View SolutionA cubical block of mass M and edge a slides down a rougg inclined plane of inclination θ with a uniform velocity. The torque of the normal force on the block about its centre has magnitude.
View SolutionIn a free space a rifle on mass M shoots a bullet of mass m at a stationary block of mass M distance D away from it . When the bullet has moved through a distance d towards the block the centre of mass of the bullet − block system is at a distance of :
View SolutionA block of mass 3M connected to a massless rod of length L lies at rest on a fixed frictionless table. A second block of mass M moving with speed v0 strikes the opposite end of the rod at right angle and sticks. The velocity of centre of mass of the system after collision is
_PHY_E05_239_Q01.png)
View Solution
