A simple pendulum of length .l. carries a bob of mass m. If the breaking strenght of the string is 2 mg, the maximum angular amplitude from the vertical can be
The correct Answer is:C
To solve the problem, we need to analyze the forces acting on the bob of the pendulum and apply the principles of energy conservation. Let's break down the solution step by step.
Step 1: Understand the Forces Acting on the Bob
The forces acting on the bob of mass m when it is at an angle θ from the vertical are:
- The gravitational force mg acting downward.
- The tension T in the string acting along the string.
At the maximum angular amplitude, the tension in the string must not exceed its breaking strength, which is given as 2mg.
Step 2: Analyze the Forces at Maximum Amplitude
At the maximum amplitude, the tension in the string can be expressed as:
T=mgcosθ+mv2l
where v is the speed of the bob at the lowest point of the swing, and l is the length of the pendulum.
Step 3: Set Up the Equation for Maximum Tension
Since the maximum tension T cannot exceed 2mg, we set up the equation:
2mg=mgcosθ+mv2l
Step 4: Solve for Speed v
Rearranging the equation gives:
2mg−mgcosθ=mv2l
Factoring out mg:
mg(2−cosθ)=mv2l
Dividing both sides by m:
g(2−cosθ)=v2l
Thus, we can express v2 as:
v2=gl(2−cosθ)
Step 5: Apply the Work-Energy Theorem
Using the work-energy theorem, the change in potential energy as the bob moves from the maximum height to the lowest point is equal to the kinetic energy at the lowest point:
mgh=12mv2
The height h can be expressed as:
h=l(1−cosθ)
Thus, we have:
mgl(1−cosθ)=12mv2
Substituting v2 from the previous step:
mgl(1−cosθ)=12m(gl(2−cosθ))
Step 6: Simplify the Equation
Cancelling m and l from both sides gives:
g(1−cosθ)=12g(2−cosθ)
Dividing both sides by g (assuming g≠0):
1−cosθ=12(2−cosθ)
Expanding the right side:
1−cosθ=1−12cosθ
Step 7: Solve for cosθ
Rearranging gives:
1−1=−12cosθ+cosθ
0=12cosθ
Thus, we find:
cosθ=12
Step 8: Find the Maximum Angular Amplitude
The angle θ corresponding to cosθ=12 is:
θ=60∘
Final Answer
The maximum angular amplitude from the vertical can be 60∘.
---
The forces acting on the bob of mass m when it is at an angle θ from the vertical are:
- The gravitational force mg acting downward.
- The tension T in the string acting along the string.
At the maximum amplitude, the tension in the string can be expressed as:
T=mgcosθ+mv2l
where v is the speed of the bob at the lowest point of the swing, and l is the length of the pendulum.
Since the maximum tension T cannot exceed 2mg, we set up the equation:
2mg=mgcosθ+mv2l
Rearranging the equation gives:
2mg−mgcosθ=mv2l
Factoring out mg:
mg(2−cosθ)=mv2l
Dividing both sides by m:
g(2−cosθ)=v2l
Thus, we can express v2 as:
v2=gl(2−cosθ)
Using the work-energy theorem, the change in potential energy as the bob moves from the maximum height to the lowest point is equal to the kinetic energy at the lowest point:
mgh=12mv2
The height h can be expressed as:
h=l(1−cosθ)
Thus, we have:
mgl(1−cosθ)=12mv2
Substituting v2 from the previous step:
mgl(1−cosθ)=12m(gl(2−cosθ))
Cancelling m and l from both sides gives:
g(1−cosθ)=12g(2−cosθ)
Dividing both sides by g (assuming g≠0):
1−cosθ=12(2−cosθ)
Expanding the right side:
1−cosθ=1−12cosθ
Rearranging gives:
1−1=−12cosθ+cosθ
0=12cosθ
Thus, we find:
cosθ=12
The angle θ corresponding to cosθ=12 is:
θ=60∘
The maximum angular amplitude from the vertical can be 60∘.
Similar Questions
A pendulum consisting of a small sphere of mass m, suspended by a inextensible and massless string of length 1 , is made to swing in a vertical plane. If the breaking strength of the string is 2 mg, then the maximum angular amplitude of the displacement from the vertical can be
View SolutionA pendulum consisting of a small sphere of mass m, suspended by a inextensible and massless string of length 1 , is made to swing in a verticle plane. If the breaking strength of the string is 2 mg, then the maximum angular amplitude of the displacement from the verticle can be
View SolutionA simple pendulum with a bob of mass m and a conducting wire of length L, swings under gravity with an angular amplitude 2θ. If the horizontal component of the earth's magnetic field perpendicular to the plane of motion of the pendulum is B, then the maximum emf induced across the pendulum is
View SolutionA simple pendulm has a length L and a bob of mass M. The bob is vibrating with amplitude a .What is the maximum tension in the string?
View SolutionA simple pendulum of length 1 m with a bob of mass m swings with an angular amplitude 30∘. Then (g=9.8m/s2
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
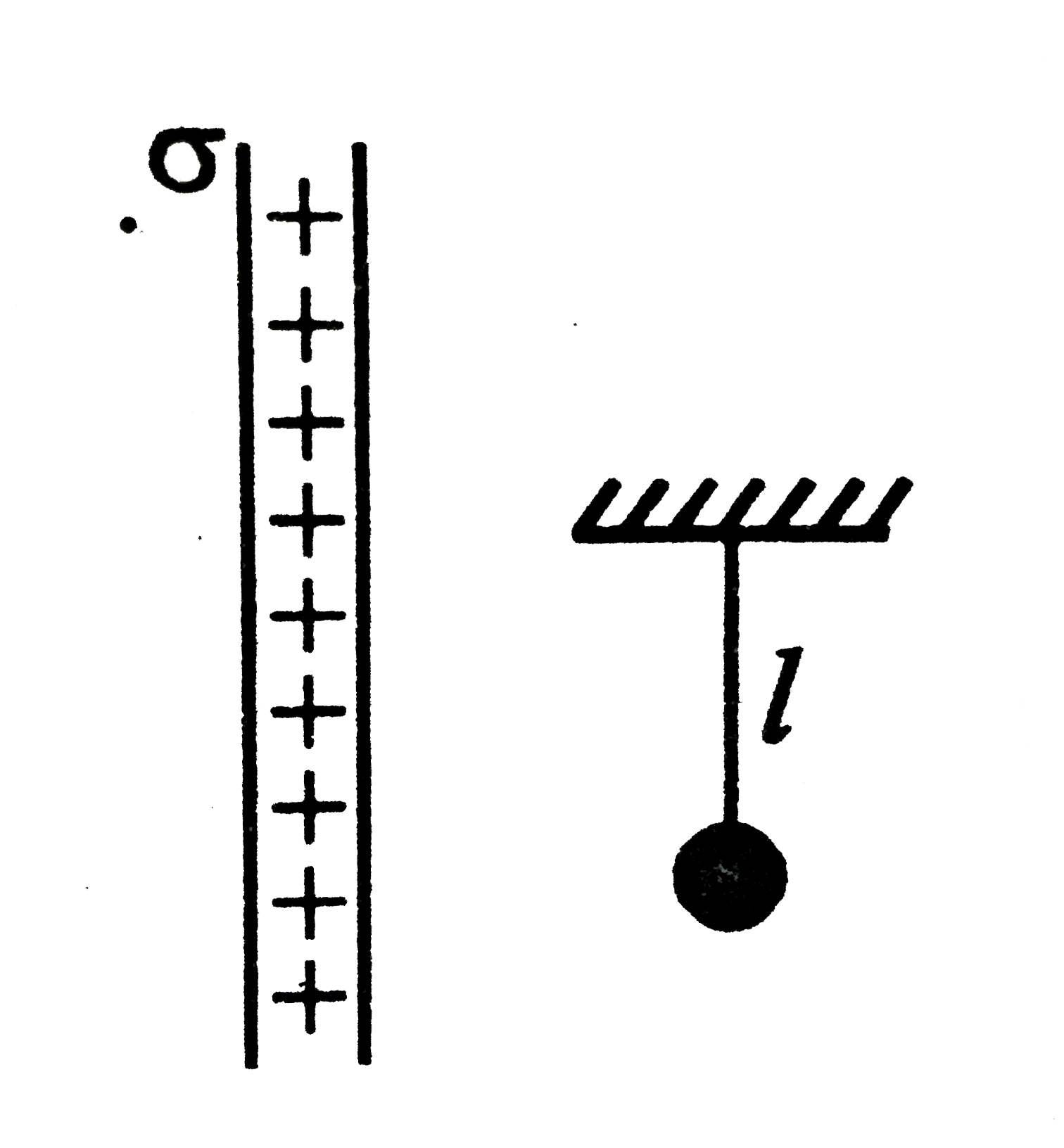 .
.
View SolutionA simple pendulum of length l and mass m is initially at its lowest position. It is given the minimum horizontal speed necessary after to move in a circular path about the point of suspension. The tension in the string at the lowest positive of the bob is
View SolutionA simple pendulum of length l has a maximum angular displacement θ. The maximum kinetic energy of the bob of mass m will be
View SolutionA simple pendulum of length 40 cm oscillates with an angular amplitude of 0.04 rad. Find a. the time period b. the linear amplitude of the bob, c. The speed of the bob when the string makes 0.02 rad with the vertical and d. the angular acceleration when the bob is in momentarily rest. Take g=10ms−2.
View Solution