Electric potential inside hollow charged sphere of radius .r. is ______ [k=14πε0]
zero
kqr
kqr2
kq2r
The correct Answer is:B
To find the electric potential inside a hollow charged sphere of radius r, we can follow these steps:
Step 1: Understand the configuration
We have a hollow charged sphere with a uniform charge distributed over its outer surface. The sphere has a radius r.
Step 2: Recognize the properties of electric fields in hollow spheres
According to Gauss's law, the electric field inside a hollow charged sphere is zero. This is because the charge resides only on the outer surface, and by symmetry, any point inside the hollow region experiences no net electric field.
Step 3: Relate electric field to electric potential
The electric potential V is related to the electric field E by the equation:
V=−∫E⋅dr
Since the electric field E inside the hollow sphere is zero, the integral simplifies.
Step 4: Calculate the electric potential inside the sphere
Since the electric field E is zero inside the hollow sphere, the potential V remains constant throughout the hollow region. The potential inside the sphere is equal to the potential at the surface of the sphere.
Step 5: Calculate the potential at the surface
The electric potential V at the surface of the sphere (at radius r) due to the charge Q on the sphere is given by:
V=kQr
where k=14πϵ0.
Step 6: Conclude the potential inside the hollow sphere
Since the potential is constant inside the hollow sphere and equal to the potential at the surface, we conclude:
Vinside=Vsurface=kQr
Thus, the electric potential inside the hollow charged sphere of radius r is:
kQr
We have a hollow charged sphere with a uniform charge distributed over its outer surface. The sphere has a radius r.
According to Gauss's law, the electric field inside a hollow charged sphere is zero. This is because the charge resides only on the outer surface, and by symmetry, any point inside the hollow region experiences no net electric field.
The electric potential V is related to the electric field E by the equation:
V=−∫E⋅dr
Since the electric field E inside the hollow sphere is zero, the integral simplifies.
Since the electric field E is zero inside the hollow sphere, the potential V remains constant throughout the hollow region. The potential inside the sphere is equal to the potential at the surface of the sphere.
The electric potential V at the surface of the sphere (at radius r) due to the charge Q on the sphere is given by:
V=kQr
where k=14πϵ0.
Since the potential is constant inside the hollow sphere and equal to the potential at the surface, we conclude:
Vinside=Vsurface=kQr
kQr
Topper's Solved these Questions
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:PRACTICE SHEET (EXERCISE -IV ELECTRIC POTENTIAL & POTENTIAL ENERGY) (LEVEL - II (ADVANCED) MORE THAN ONE CORRECT ANSWER TYPE QUESTION)
Explore 1 Video
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:PRACTICE SHEET (EXERCISE -IV ELECTRIC POTENTIAL & POTENTIAL ENERGY) (LEVEL - II (ADVANCED) LINKED COMPREHENSION TYPE QUESTION)
Explore 2 Videos
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:PRACTICE SHEET (EXERCISE -IV ELECTRIC POTENTIAL & POTENTIAL ENERGY) (LEVEL - I (MAIN) STRAIGHT OBJECTIVE TYPE QUESTION)
Explore 19 Videos
- ELECTROMAGNETICSBook:AAKASH SERIESChapter:ELECTROMAGNETICSExercise:ADDITIONAL EXERCISE
Explore 13 Videos
- ELEMENTS OF VECTORSBook:AAKASH SERIESChapter:ELEMENTS OF VECTORSExercise:QUESTIONS FOR DESCRIPTIVE ANSWERS
Explore 10 Videos
Similar Questions
A ring of radius 3 m has charge density λ. Electric potential at 4 m from its centre on its axis is ____ [k=14πε0]
View SolutionElectric potentiall at centre of non conducting sphere of radii .r. is ____ [q is charge in itk=14πε0]
View SolutionFind the electric potential energy of a uniformly charged sphere.
View SolutionElectric potential due to a dipole at a position →r from its centre is: where (K=14πε0
View SolutionFour charges of same magnitude q are placed at four corners of a square of side a . The value electric potential at the centre of the souare will be (where k = 14πε0 )
View SolutionFor spherical symmetrical charge distribution, variation of electric potential with distance from centre is given in diagram Given that : V=q4πε0R0 for r≤R0andV=q4πε0r for r≥R0
Then which option (s) are correct :
(1) Total charge within 2R0 is q
(2) Total electrosstatic energy for r≤R0 is non-zero
(3) At r=R0 electric field is discontinuous
(4) There will be no charge anywhere except at r<R0

View SolutionFive charges each q are placed at five corners regular pentagon. Distance from corner to the centre of pontagon is r. Then (K=14πε0)
View SolutionThe electric potential due to uniformly charged sphere of radius R,having volume charge density rho having a spherical cavity of radius R2 as shown as in figure at point p is
_PHY_E05_288_Q01.png)
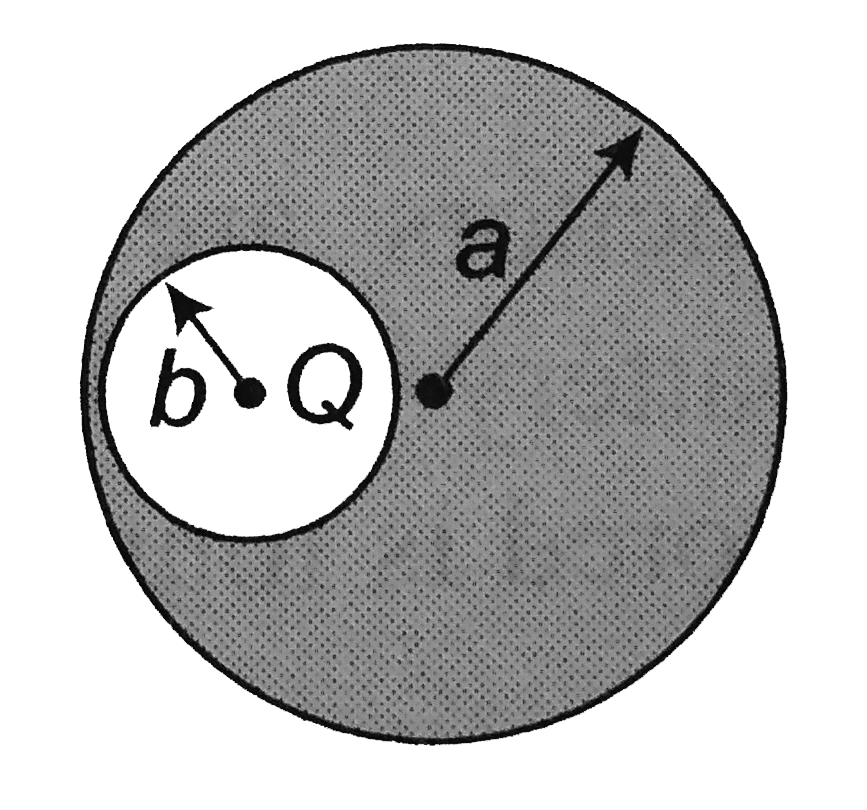
View SolutionA point charge 'Q' is placed at the centre of a spherical cavity of radius 'b' carved inside a solid conducting sphere of radius 'a'. Then total energy of the system is:
[k=14π∈0]
View SolutionA dipole of dipole moment P is kept at the centre of a ring of radius R and charge Q. If the dipole lies along the axis of the ring. Electric force on the ring due to the dipole is : (K=14πε0)
View Solution