What will be the minimum frequency of light source to get photocurrent, from a metal surface having work function 2 eV ?
4.8×1014Hz
5.8×1014Hz
7.8×1014Hz
2.8×1014Hz
The correct Answer is:A
To find the minimum frequency of light required to produce photocurrent from a metal surface with a work function of 2 eV, we can follow these steps:
1. Understand the Work Function:
The work function (ϕ) is the minimum energy required to remove an electron from the surface of a metal. In this case, the work function is given as 2 eV.
2. Convert Work Function to Joules:
Since the energy is given in electron volts (eV), we need to convert it to joules (J).
1 eV=1.6×10−19 J
Therefore,
ϕ=2 eV=2×1.6×10−19 J=3.2×10−19 J
3. Use the Photoelectric Equation:
The photoelectric effect can be described by the equation:
K.E.=hν−ϕ
where K.E. is the kinetic energy of the emitted electron, h is Planck's constant, and ν is the frequency of the incident light. For photocurrent to be generated, the kinetic energy must be greater than or equal to zero:
0≤hν−ϕ
Rearranging gives:
hν≥ϕ
4. Substitute Known Values:
Planck's constant h is approximately 6.63×10−34 J s. We can substitute the values into the equation:
ν≥ϕh=3.2×10−19 J6.63×10−34 J s
5. Calculate the Frequency:
Performing the division:
ν≥3.2×10−196.63×10−34≈4.82×1014 Hz
6. Conclusion:
The minimum frequency of the light source required to produce photocurrent from the metal surface is approximately 4.82×1014 Hz.
Final Answer:
The minimum frequency of the light source to get photocurrent is 4.82×1014 Hz.
---
The work function (ϕ) is the minimum energy required to remove an electron from the surface of a metal. In this case, the work function is given as 2 eV.
Since the energy is given in electron volts (eV), we need to convert it to joules (J).
1 eV=1.6×10−19 J
Therefore,
ϕ=2 eV=2×1.6×10−19 J=3.2×10−19 J
The photoelectric effect can be described by the equation:
K.E.=hν−ϕ
where K.E. is the kinetic energy of the emitted electron, h is Planck's constant, and ν is the frequency of the incident light. For photocurrent to be generated, the kinetic energy must be greater than or equal to zero:
0≤hν−ϕ
Rearranging gives:
hν≥ϕ
Planck's constant h is approximately 6.63×10−34 J s. We can substitute the values into the equation:
ν≥ϕh=3.2×10−19 J6.63×10−34 J s
Performing the division:
ν≥3.2×10−196.63×10−34≈4.82×1014 Hz
The minimum frequency of the light source required to produce photocurrent from the metal surface is approximately 4.82×1014 Hz.
The minimum frequency of the light source to get photocurrent is 4.82×1014 Hz.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - EXERCISE=II
42 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE - IA
2 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise
57 Videos
Similar Questions
Calculate the value of retarding potential in volt needed to stop the photoelectron ejected from a metal surface of work function 1.29 eV with light frequency of 5.5×1014sec−1 (nearly)
View SolutionFind the retarding potential requred to stop the escape of photo electrons from the metal surface of work function 1.07 eV , which is irradiated with light of wavelength 332 mn
View SolutionThe material having minimum work function is
View SolutionIn photoelectric effect light of certain frequency (> threshold frequency ) is incident on a metal surfave whereby , an e− ( with certain K.E.) moves towards the collector plate and a flow of current is initiated . In order to stop the current flow, an opposite potential , on the two metal plates, is applied
A light of frequency 2.5×1015Hz is incident on a metal surface having work function 4 eV. The velocity of photoelectron is ( in cm s−1)
View SolutionWhat will be the maximum kinetic energy of the photoelectrons ejected from magnesium (for which the work -function W=3.7 eV) when irradiated by ultraviolet light of frequency 1.5×1015s−1.
View SolutionLight from balmer series of hydrogen is able to eject photoelectron from a metal what can be the maximum work function of the metal?
View SolutionThe following figure shows a graph for the stopping potential as a function of the frequency of incident light illuminating a metal surface. Find the photoelectric work function for this surface.
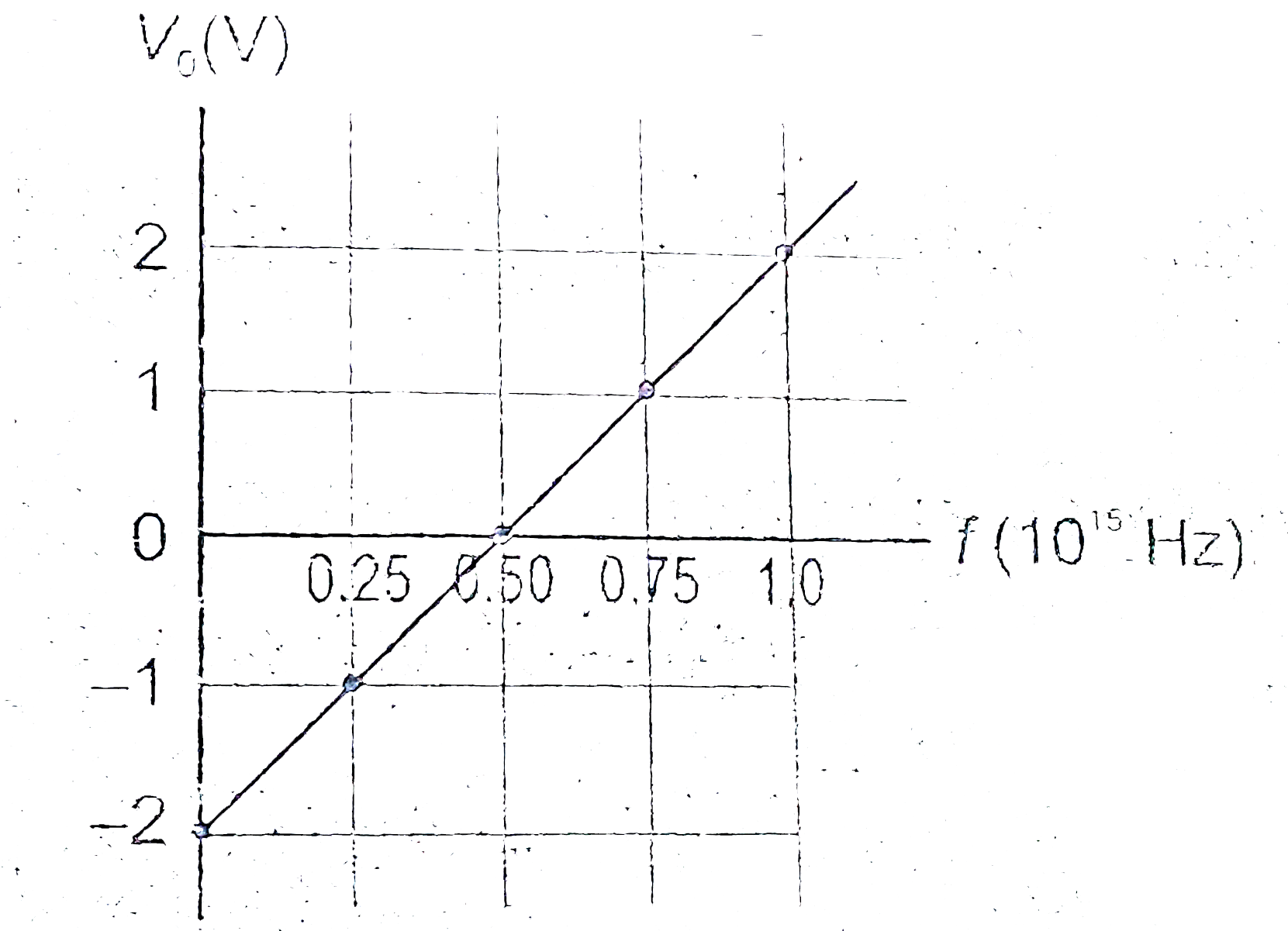
View Solutionif the speed of photoelectrons is 104 m/s, what should be the frequency of the incident radiation on a potassium metal ? Given : Work function of potassium =2.3 eV
View SolutionFind the threshold wavelength of light that would produce photoelectrons for a silver surface . The work function for silver is 4.8 eV
View SolutionThe threshold wavelength for emission of photoelectrons from a metal surface is 6×10−7 m. What is the work function of the material of the metal surface ?
View Solution
