if the speed of photoelectrons is 104 m/s, what should be the frequency of the incident radiation on a potassium metal ? Given : Work function of potassium =2.3 eV
To find the frequency of the incident radiation on potassium metal when the speed of photoelectrons is 104 m/s and the work function of potassium is 2.3 eV, we can use Einstein's photoelectric equation:
E=W0+KE
Where:
- E is the energy of the incident radiation,
- W0 is the work function of the metal,
- KE is the maximum kinetic energy of the emitted photoelectrons.
Step 1: Convert the work function from eV to Joules
The work function W0 is given as 2.3 eV. We need to convert this into Joules using the conversion factor 1 eV=1.6×10−19 J.
W0=2.3eV×1.6×10−19J/eV=3.68×10−19J
Step 2: Calculate the maximum kinetic energy (KE) of the photoelectrons
The maximum kinetic energy KE can be calculated using the formula:
KE=12mv2
Where:
- m is the mass of an electron, approximately 9.1×10−31kg,
- v is the speed of the photoelectrons, given as 104m/s.
Substituting the values:
KE=12×9.1×10−31kg×(104m/s)2
Calculating KE:
KE=12×9.1×10−31×108=4.55×10−23J
Step 3: Use Einstein's equation to find the energy of the incident radiation
Now, we can substitute W0 and KE into Einstein's equation:
E=W0+KE
Substituting the values we have:
E=3.68×10−19J+4.55×10−23J≈3.68×10−19J
(Note: The KE term is very small compared to W0, so it can be approximated.)
Step 4: Relate the energy of the incident radiation to its frequency
The energy of the incident radiation can also be expressed in terms of frequency:
E=hν
Where:
- h is Planck's constant, approximately 6.63×10−34J s,
- ν is the frequency.
Rearranging to find ν:
ν=Eh
Step 5: Substitute the values to find the frequency
Substituting E and h:
ν=3.68×10−19J6.63×10−34J s≈5.56×1014Hz
Final Answer
The frequency of the incident radiation is approximately:
ν≈5.56×1014Hz
---
- E is the energy of the incident radiation,
- W0 is the work function of the metal,
- KE is the maximum kinetic energy of the emitted photoelectrons.
The work function W0 is given as 2.3 eV. We need to convert this into Joules using the conversion factor 1 eV=1.6×10−19 J.
The maximum kinetic energy KE can be calculated using the formula:
- m is the mass of an electron, approximately 9.1×10−31kg,
- v is the speed of the photoelectrons, given as 104m/s.
Now, we can substitute W0 and KE into Einstein's equation:
The energy of the incident radiation can also be expressed in terms of frequency:
- h is Planck's constant, approximately 6.63×10−34J s,
- ν is the frequency.
Substituting E and h:
The frequency of the incident radiation is approximately:
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Try Yourself
15 Videos
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - A) ( Objective Type Questions ( One option is correct))
40 Videos
- ATOMSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ATOMSEXERCISE - ASSIGNMENT SECTION J (Aakash Challengers )
5 Videos
Similar Questions
The work function of a metal is 3.4 eV. If the frequency of incident radiation is increased to twice, then the work function of the metal becomes.
View SolutionThreshold frequency for a certain metal is v0. When light of frequency 2v0 is incident on it, the maximum velocity of photoelectrons is 4×108cms−1. If frequency of incident radiation is increased to 5v0, then the maximum velocity of photoelectrons, in cm s−1, will be
View SolutionThe threshold frequency for certain metal is v0. When light of frequency 2v0 is incident on it, the maximum velocity of photoelectrons is 4×106ms−1. If the frequency of incident radiation is increaed to 5v0, then the maximum velocity of photoelectrons will be
View SolutionWhat will be the minimum frequency of light source to get photocurrent, from a metal surface having work function 2 eV ?
View SolutionThe kinetic energy of the most energetic photoelectrons emitted from a metal surface is doubled when the wavelength of the incident radiation is reduced from λ1 to λ2 The work function of the metal is
View SolutionThe maximum kinetic energy of photoelectrons emitted from a metal surface increses from 0.4 eV to 1.2 eV when the frequency of the incident radiation is increased by 40% . What is the work function (in eV) of the metal surface?
View SolutionWhen ultraviolet radiation of a certain frequency falls on a potassium target, the photoelectrons released can be stopped completely by a retarding potential of 0.5 V. If the frequency of the radiation is increased by 10 %, this stopping potential rises to 0.9 V. The work function of potassium is
View Solution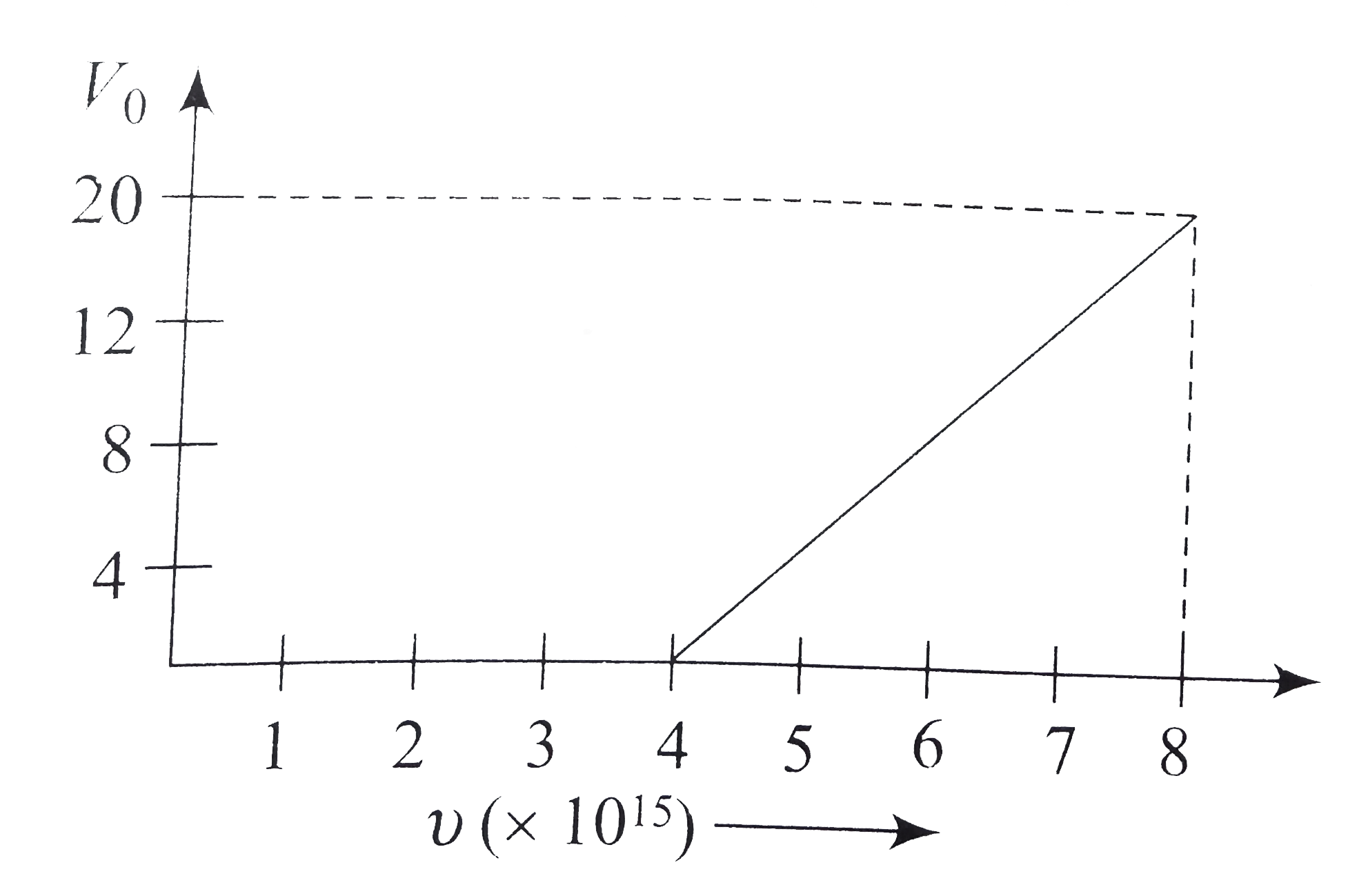
When a high frequency electromagnetic radiation is incident on a metallic surface, electrons are emitted from the surface. Energy of emitted photoelectrons depends only on the frequency of incident electromagnetic radiation and the number of emitted electrons depends only on the intensity of incident light.
Einstein's protoelectron equation [Kmax=hv−ϕ] correctly ecplains the PE, where υ= frequency of incident light and ϕ= work function.
Q. For photoelectric effect in a metal, the graph of the stopping potential V0 (in volt) versus frequency υ (in hertz) of the incident radiation is shown in Fig. The work function of the metal (in eV) is.
View SolutionIf the work function of a metal is 'ϕ' and the frequency of the incident light is 'v', there is no emission of photoelectron if
View SolutionThe threshold frequency of a material is 2×1014 Hz. What is its work function in eV ?
View Solution
