Three identical spheres each of radius .R. are placed touching each other so that their centres A, B and C lie on a straight line. The position of their centre of mass from A is
2R3
2R
5R3
4R3
The correct Answer is:B
To find the position of the center of mass of three identical spheres placed touching each other in a straight line, we can follow these steps:
1. Understanding the Arrangement:
- We have three identical spheres, each with a radius R.
- The centers of the spheres are labeled as A, B, and C.
- The spheres are touching each other, so the distance between the centers of adjacent spheres is 2R.
2. Identifying Distances:
- The distance from center A to center B is 2R.
- The distance from center A to center C is 4R (since A to B is 2R and B to C is another 2R).
3. Assigning Masses:
- Let the mass of each sphere be m.
- Therefore, we have:
- Mass at A = m
- Mass at B = m
- Mass at C = m
4. Using the Center of Mass Formula:
- The formula for the center of mass xcm of a system of particles is given by:
xcm=m1x1+m2x2+m3x3m1+m2+m3
- Here, we take point A as the reference point (i.e., xA=0):
- x1=0 (for mass at A)
- x2=2R (for mass at B)
- x3=4R (for mass at C)
5. Substituting Values into the Formula:
- Plugging in the values:
xcm=m⋅0+m⋅2R+m⋅4Rm+m+m
- This simplifies to:
xcm=0+2mR+4mR3m=6mR3m
6. Final Calculation:
- The m cancels out:
xcm=6R3=2R
Conclusion:
The position of the center of mass from point A is 2R.
- We have three identical spheres, each with a radius R.
- The centers of the spheres are labeled as A, B, and C.
- The spheres are touching each other, so the distance between the centers of adjacent spheres is 2R.
- The distance from center A to center B is 2R.
- The distance from center A to center C is 4R (since A to B is 2R and B to C is another 2R).
- Let the mass of each sphere be m.
- Therefore, we have:
- Mass at A = m
- Mass at B = m
- Mass at C = m
- The formula for the center of mass xcm of a system of particles is given by:
xcm=m1x1+m2x2+m3x3m1+m2+m3
- Here, we take point A as the reference point (i.e., xA=0):
- x1=0 (for mass at A)
- x2=2R (for mass at B)
- x3=4R (for mass at C)
- Plugging in the values:
xcm=m⋅0+m⋅2R+m⋅4Rm+m+m
- This simplifies to:
xcm=0+2mR+4mR3m=6mR3m
- The m cancels out:
xcm=6R3=2R
The position of the center of mass from point A is 2R.
Similar Questions
Three identical spheres each of radius .R. are placed on a horizontal surface touching one another. If one of the spheres is removed, the shift in the centre of mass of the system is
View SolutionThree identical metal balls each of radius r are placed touching each other on a horizontal surface such that an equilateral triangle is formed, when the center of three balls are joined. The center of mass of system is located at the
View Solution
Knowledge Check
Two identical spheres each of mass M and Radius R are separated by a distance 10R. The gravitational force on mass m placed at the midpoint of the line joining the centres of the spheres is
AzeroB2GMm25R2CGMm25R2DGMm100R2
Four identical spheres each of radius 10 cm and mass 1 kg are placed on a horizontal surface touching one another so that their centres are located at the corners of square of side 20 cm. What is the distance of their centre of mass from centre of either sphere ?
View SolutionThree identicle particle each of mass 1kg are placed with their centres on a straight line. Their centres are marked P,QandR respectively. The distance of centre of mass of the system from P is.
View SolutionThree identical spheres of mass M each are placed at the corners of an equilateral triangle of side 2 m. Taking one of the corners as the origin, the position vector of the centre of mass is
View SolutionA solid sphere of radius R is placed on a cube of side 2R. Both are made of same material. Find the distance of their centre of mass fro the interface.
View SolutionA square lamina of side a and a circular lamina of diameter a are placed touching each other as shown in figure. Find distance of their centre of mass from point O, the centre of square.
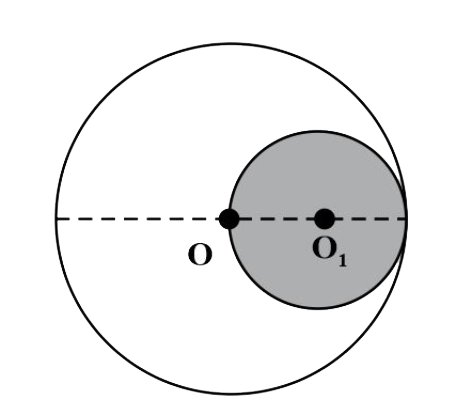
View SolutionA spherical cavity is made in a lead sphere of radius R such that its surface touches the outsides surface of lead sphere and passes through the centre. The shift in the centre of mass of the lead sphere as a result of this following, is
View SolutionThree identical particles each of mass m are placed at the vertices of an equilateral triangle of side a. Fing the force exerted by this system on a particle P of mass m placed at the

(a) the mid point of a side
(b) centre of the triangle
View Solution