Two wires of equal length are stretched by the same force. One wire is heavy while the other is light which one will have less frequency
heavier wire
lighter wire
both have same frequency
data insufficient
The correct Answer is:A
To solve the problem of determining which wire will have less frequency when two wires of equal length are stretched by the same force, we can follow these steps:
1. Understanding the Setup:
- We have two wires of equal length (L1 = L2).
- They are subjected to the same tensile force (F1 = F2).
- One wire is heavier (mass M1) and the other is lighter (mass M2), with M1 > M2.
2. Frequency of Vibrating Strings:
- The frequency of a vibrating wire is determined by its mass and tension. The formula for the fundamental frequency (f) of a wire is given by:
f∝1√m
where m is the mass per unit length (linear density) of the wire.
3. Relating Mass and Frequency:
- Since both wires are of equal length and are stretched by the same force, we can say:
f1∝1√M1(for the heavier wire)
f2∝1√M2(for the lighter wire)
4. Comparing Frequencies:
- Given that M1 > M2, we can analyze the relationship:
- Since M1 (mass of the heavier wire) is greater than M2 (mass of the lighter wire), it follows that:
√M1>√M2
- Therefore, we can conclude:
f1<f2
- This means that the frequency of the heavier wire (f1) is less than the frequency of the lighter wire (f2).
5. Final Conclusion:
- Since the heavier wire has a lower frequency compared to the lighter wire, the answer to the question is that the heavier wire will have less frequency.
Answer:
The heavier wire will have less frequency.
---
- We have two wires of equal length (L1 = L2).
- They are subjected to the same tensile force (F1 = F2).
- One wire is heavier (mass M1) and the other is lighter (mass M2), with M1 > M2.
- The frequency of a vibrating wire is determined by its mass and tension. The formula for the fundamental frequency (f) of a wire is given by:
f∝1√m
where m is the mass per unit length (linear density) of the wire.
- Since both wires are of equal length and are stretched by the same force, we can say:
f1∝1√M1(for the heavier wire)
f2∝1√M2(for the lighter wire)
- Given that M1 > M2, we can analyze the relationship:
- Since M1 (mass of the heavier wire) is greater than M2 (mass of the lighter wire), it follows that:
√M1>√M2
- Therefore, we can conclude:
f1<f2
- This means that the frequency of the heavier wire (f1) is less than the frequency of the lighter wire (f2).
- Since the heavier wire has a lower frequency compared to the lighter wire, the answer to the question is that the heavier wire will have less frequency.
The heavier wire will have less frequency.
Topper's Solved these Questions
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-IA (Statement type questions:)
18 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-IA (Matching )
4 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Doppler effect :)
15 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PROBLEMS (LEVEL - II)
33 Videos
- WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -III (POLARISITION)
10 Videos
Similar Questions
Two wires of the same material and length are stretched by the same force. Their masses are in the ratio 3:2. Their elongations are in the ratio
View SolutionTwo wires of the same material and same mass are stretched by the same force. Their lengths are in the ratio 2:3. Their elongation are in the ratio
View Solution
Knowledge Check
A wire is stretched by one mm by a force of 1 kN. The work done is stretching the wire is
A5 ergsB5 JoulesC0.5 ergsD0.5 Joules
Two wires having same length and material are stretched by same force. Their diameters are in the ratio 1:3. The ratio of strain energy per unit volume for these two wires (smaller to larger diameter) when stretched is
View SolutionTwo wires of equal length one of copper and other of manganin have the same resistance. Which wire is thicker?
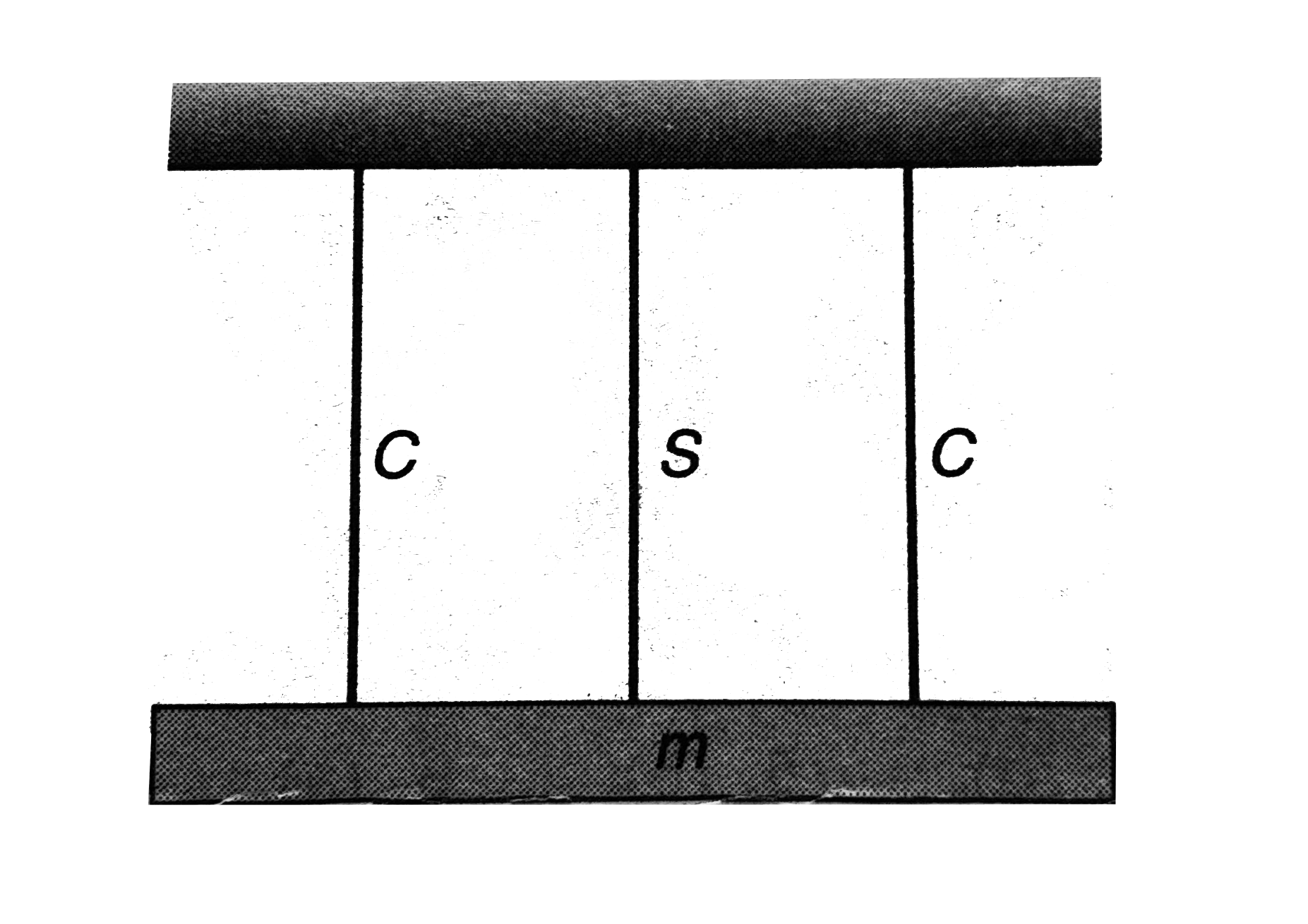
View SolutionA homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
View Solution(a) Name two factors on which the specific resistance of a wire depends ?
(b) Two wires A and B are made of copper. The wire A is long and thin, while the wire B is short and thick. Which will have more specific resistance ?
View SolutionTwo steel wires of the same radius have their lengths in the ratio of 1:2. If they are stretched by the same force, then the strains produced in the two wires will be in the ratio of
View SolutionTwo wires P and Q are equally thick and made of the same material but the length of Pis twice that of Q
(a) Which wire will have a greater extension for a given load ?
(b) Which one will undergo a greater strain ?
View SolutionTwo wires A and B of same dimensions are stretched by same amount of force .Young's modulus of A is twice that of B. Which wire will get more elongation?
View Solution
