Doubtnut Promotions Sticky

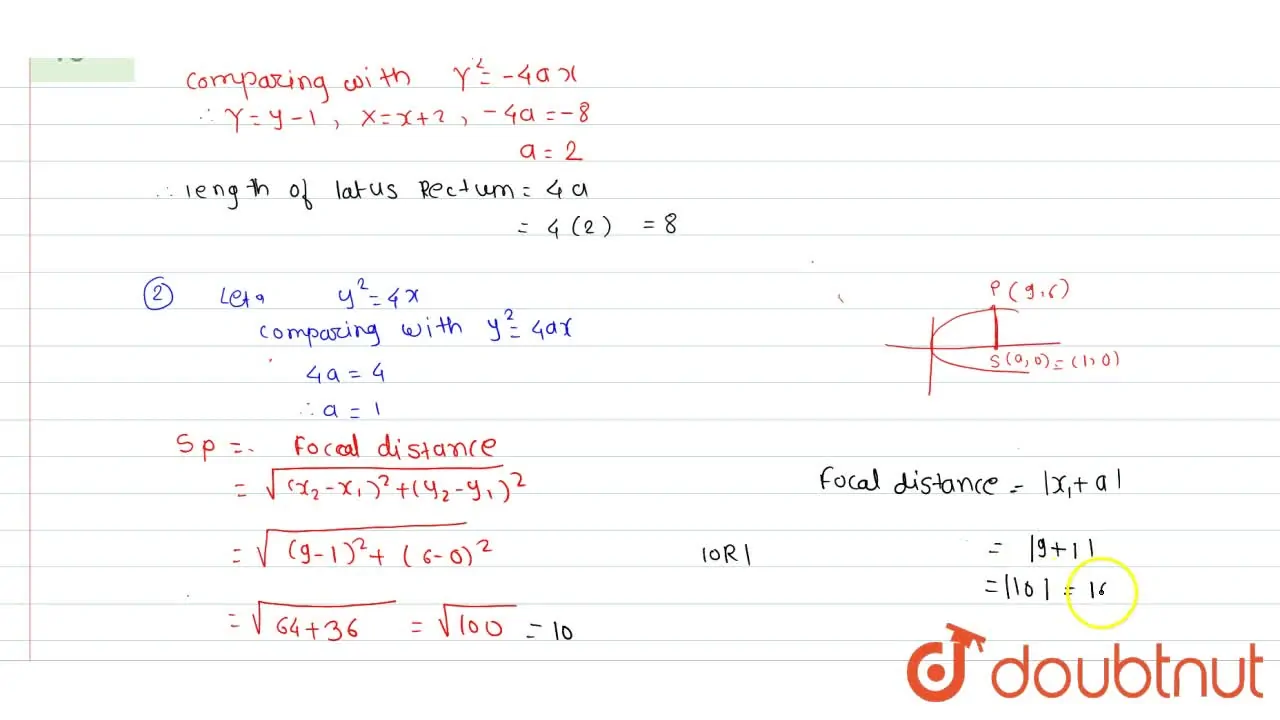
I : The length of the latus rectum of the parabola y2+8x−2y+17=0 is 8 .
II: The focal distance of the point (9,6) on the parabola y2=4x is 10

A

2

B

4

C

8 , 10

D

16

Video Solution
Doubtnut Promotions Banner
Text Solution
Generated By DoubtnutGPT

The correct Answer is:C

To solve the given problem, we will break it down into two parts as indicated in the question.

Part I: Length of the Latus Rectum of the Parabola

1. Given Equation: The equation of the parabola is y2+8x−2y+17=0.

2. Rearranging the Equation: We need to rearrange this equation into the standard form of a parabola. Start by isolating the y terms:
y2−2y+8x+17=0

3. Completing the Square: We complete the square for the y terms:
y2−2y=(y−1)2−1
Substitute this back into the equation:
(y−1)2−1+8x+17=0
Simplifying gives:
(y−1)2+8x+16=0
Rearranging this, we get:
(y−1)2=−8(x+2)

4. Identifying Parameters: Now, we can compare this with the standard form (y−k)2=−4a(x−h). Here, we have:
- k=1
- h=−2
- 4a=8 which implies a=2.

5. Finding the Length of the Latus Rectum: The length of the latus rectum L is given by the formula:
L=4a
Substituting a=2:
L=4×2=8

Part II: Focal Distance of the Point (9, 6) on the Parabola y2=4x

1. Given Equation: The equation of the parabola is y2=4x.

2. Identifying Parameters: This is already in the standard form y2=4ax. Here, we have:
- 4a=4 which implies a=1.
- The focus of the parabola is at (a,0)=(1,0).

3. Finding the Focal Distance: The focal distance SP from the point P(9,6) to the focus S(1,0) can be calculated using the distance formula:
SP=√(x2−x1)2+(y2−y1)2
Here, (x1,y1)=(1,0) and (x2,y2)=(9,6):
SP=√(9−1)2+(6−0)2
Calculating gives:
SP=√82+62=√64+36=√100=10

Summary of Results
- The length of the latus rectum of the parabola y2+8x−2y+17=0 is 8.
- The focal distance of the point (9,6) on the parabola y2=4x is 10.

---

|