A heavy uniform chain lies on horizontal table top. If the co-efficient of friction between the chain and the table surface is 0.5, the maximum percentage of the length of the chain that can hang over one edge of the table is
20%
33.3%
75%
50%
The correct Answer is:B
To solve the problem, we will analyze the forces acting on the chain and apply the principles of equilibrium and friction.
1. Understanding the Setup:
- We have a heavy uniform chain lying on a horizontal table, with a portion hanging over the edge.
- Let the total length of the chain be l.
- Let x be the length of the chain hanging over the edge of the table. Therefore, the length of the chain on the table is l−x.
2. Forces Acting on the Chain:
- The weight of the hanging part of the chain is given by Wh=m⋅g⋅x, where m is the mass per unit length of the chain, and g is the acceleration due to gravity.
- The normal force N acting on the part of the chain on the table is equal to the weight of the chain lying on the table: N=m⋅g⋅(l−x).
3. Frictional Force:
- The maximum static frictional force Ff that can act on the chain is given by:
Ff=μ⋅N=μ⋅m⋅g⋅(l−x)
- Here, μ is the coefficient of friction, which is given as 0.5.
4. Equilibrium Condition:
- For the chain to remain in equilibrium, the weight of the hanging part must be balanced by the frictional force:
Wh=Ff
- Substituting the expressions for Wh and Ff:
m⋅g⋅x=μ⋅m⋅g⋅(l−x)
- We can cancel m⋅g from both sides (assuming m≠0):
x=μ⋅(l−x)
5. Solving for x:
- Rearranging the equation:
x+μx=μl
x(1+μ)=μl
x=μl1+μ
6. Substituting the Value of μ:
- Substituting μ=0.5:
x=0.5l1+0.5=0.5l1.5=13l
7. Calculating the Percentage:
- The percentage of the chain that can hang over the edge is:
Percentage=(xl)×100=(1/3ll)×100=1003≈33.33%
Final Answer:
The maximum percentage of the length of the chain that can hang over one edge of the table is approximately 33.33%.
- We have a heavy uniform chain lying on a horizontal table, with a portion hanging over the edge.
- Let the total length of the chain be l.
- Let x be the length of the chain hanging over the edge of the table. Therefore, the length of the chain on the table is l−x.
- The weight of the hanging part of the chain is given by Wh=m⋅g⋅x, where m is the mass per unit length of the chain, and g is the acceleration due to gravity.
- The normal force N acting on the part of the chain on the table is equal to the weight of the chain lying on the table: N=m⋅g⋅(l−x).
- The maximum static frictional force Ff that can act on the chain is given by:
Ff=μ⋅N=μ⋅m⋅g⋅(l−x)
- Here, μ is the coefficient of friction, which is given as 0.5.
- For the chain to remain in equilibrium, the weight of the hanging part must be balanced by the frictional force:
Wh=Ff
- Substituting the expressions for Wh and Ff:
m⋅g⋅x=μ⋅m⋅g⋅(l−x)
- We can cancel m⋅g from both sides (assuming m≠0):
x=μ⋅(l−x)
- Rearranging the equation:
x+μx=μl
x(1+μ)=μl
x=μl1+μ
- Substituting μ=0.5:
x=0.5l1+0.5=0.5l1.5=13l
- The percentage of the chain that can hang over the edge is:
Percentage=(xl)×100=(1/3ll)×100=1003≈33.33%
The maximum percentage of the length of the chain that can hang over one edge of the table is approximately 33.33%.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - II
136 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
10 Videos
- LOGIC GATESBOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)
10 Videos
Similar Questions
A heavy uniform metal chain is kept on the surface of a horizontal table. The coefficient of friction between the chain and the table surface is 0.22. Find the maximum fraction of length of the chain that could hung over on one edge of the table ?
View SolutionA heavy uniform chain lies on horizontal table top. If the coefficient of friction 0.25, then the maximum fraction of length of the chain that can hang over on one edge of the table is
View Solution
Knowledge Check
A heavy uniform chain lies on horizontal table top. If the coefficient of friction between the chain and the table surface is 0.25, then the maximum fraction of the length of the chain that can hang over one edge of the table is
A0.2B0.25C0.35D0.15A 20 N block is being pushed across a horizontal table by an 18 N force. If the coefficient of kinetic friction between the block and the table is 0.4, find the acceleration of the block.
A0.5m/s2B1m/s2C5m/s2D7.5m/s2
A homogoneous chain of length L lies on a table. The coefficient of friction between the chain and the table is μ. The maximum length which can hang over the table in equilibrium is
View SolutionA chain is placed on a rough table, partially hanging, as shown in the figure. The coefficient of static friction between the chain and the table is μ = 0.4. If the maximum possible length of the hanging part is x and the length of the chain is L, then what is the value of Lx?
View SolutionA block is kept on a horizontal table. The table is undergoing simple farmonic motion of frequency 3Hz in a horizontal plane. The coefficient of static friction between the block and the table surface is 0.72. find the maximum amplitude of the table at which the block does not slip on the surface g=10ms−1
View SolutionA homogeneous chain lies in limiting equilibrium on a horizontal table of coefficient of friction 0.5 with part of it hanging over the edge of the table. The fractional length of the chain hanging down the edge of the table is
View SolutionA block is kept on a horizontal table. The stable is undergoing simple harmonic motion of frequency 3Hz in a horizontal plane. The coefficient of static friciton between block and the table surface is 0.72. find the maximum amplitude of the table at which the block does not slip on the surface.
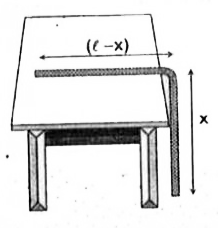
View SolutionA uniform chain of mass m and length I is kept on the table with a part of it overhanging (see figure). If the coefficient of friction between the table and the chain is 1/3 then find the maximum length of the chain that can overhang such that the chain remain in equilibrium.
View Solution
