Six forces lying in a plane and forming angles of 60∘ relative to one another are applied to the center of a homogenous sphere with a mass m = 6 kg. These forces are radially outward and consecutively IN, 2N, 3N, 4N, 5N and 6N. The acceleration of the sphere is
Zero
1/2 m/s2
1m/s2
2m/s2
The correct Answer is:C
To solve the problem, we will follow these steps:
Step 1: Identify the Forces
We have six forces acting on the sphere:
- F1 = 1 N
- F2 = 2 N
- F3 = 3 N
- F4 = 4 N
- F5 = 5 N
- F6 = 6 N
These forces are applied at angles of 60 degrees relative to each other.
Step 2: Calculate the Resultant of Opposite Forces
Since the forces are radially outward and arranged in a circular pattern, we can group them into pairs that are opposite to each other.
1. Pair F1 and F4:
- Resultant (R1) = F4 - F1 = 4 N - 1 N = 3 N
2. Pair F2 and F5:
- Resultant (R2) = F5 - F2 = 5 N - 2 N = 3 N
3. Pair F3 and F6:
- Resultant (R3) = F6 - F3 = 6 N - 3 N = 3 N
Step 3: Combine the Resultant Forces
Now we have three resultant forces (R1, R2, R3), each equal to 3 N. Since these resultant forces are also at angles of 60 degrees to each other, we can find the net resultant force (R_net) using vector addition.
Step 4: Calculate the Net Resultant Force
Since the angle between each resultant force is 60 degrees, we can use the formula for the resultant of two forces at an angle θ:
R=√R21+R22+2R1R2cos(θ)
However, since we have three equal forces (R1, R2, R3) of 3 N each, we can simplify the calculation. The resultant of three equal forces at 120 degrees can be computed as:
Rnet=3+3+3=9 N (in the direction of the resultant)
Step 5: Calculate the Acceleration of the Sphere
Using Newton's second law, we can find the acceleration (a) of the sphere:
F=ma⟹a=Fm
Where:
- F = net resultant force = 9 N
- m = mass of the sphere = 6 kg
Substituting the values:
a=9 N6 kg=1.5 m/s2
Final Answer
The acceleration of the sphere is 1.5 m/s².
---
We have six forces acting on the sphere:
- F1 = 1 N
- F2 = 2 N
- F3 = 3 N
- F4 = 4 N
- F5 = 5 N
- F6 = 6 N
Since the forces are radially outward and arranged in a circular pattern, we can group them into pairs that are opposite to each other.
- Resultant (R1) = F4 - F1 = 4 N - 1 N = 3 N
- Resultant (R2) = F5 - F2 = 5 N - 2 N = 3 N
- Resultant (R3) = F6 - F3 = 6 N - 3 N = 3 N
Now we have three resultant forces (R1, R2, R3), each equal to 3 N. Since these resultant forces are also at angles of 60 degrees to each other, we can find the net resultant force (R_net) using vector addition.
Since the angle between each resultant force is 60 degrees, we can use the formula for the resultant of two forces at an angle θ:
Using Newton's second law, we can find the acceleration (a) of the sphere:
- F = net resultant force = 9 N
- m = mass of the sphere = 6 kg
The acceleration of the sphere is 1.5 m/s².
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - II
136 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
10 Videos
- LOGIC GATESBOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)
10 Videos
Similar Questions
Knowledge Check
A body of mass 10kg is acted upon by two per pendicular forces 6N and 8N. The resultant ac-celeration of the body is .
A1ms−2 at angle of tan−1(34)w.r.t.8NforceB0.2ms−2 at angle of tan−1(34)w.r.t.8NforceC1ms−2 at angle of tan−1(43)w.r.t.8NforceD0.2ms−2 at angle of tan−1(43)w.r.t.8Nforce
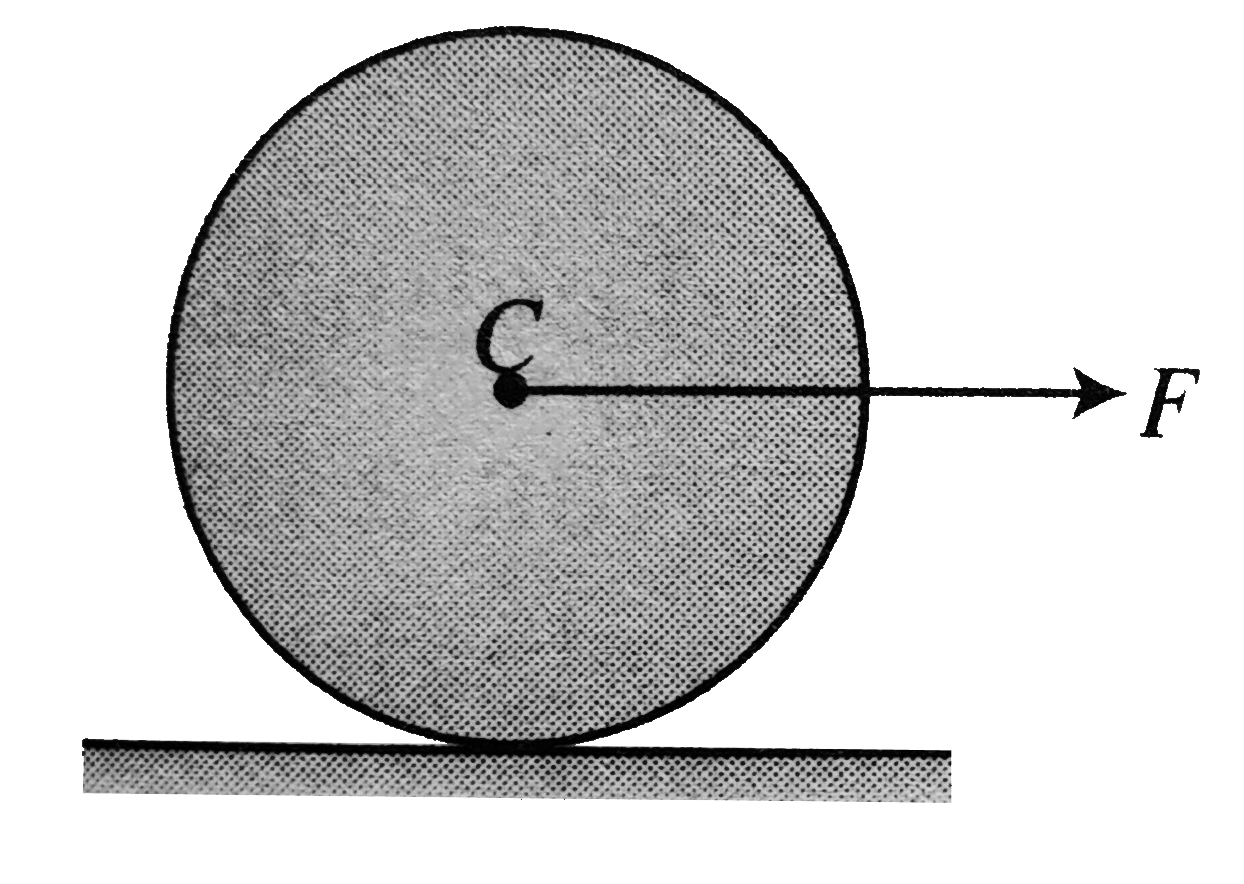
A horizontal force F=14N acts at the centre of mass of a sphere of mass m=1kg. If the sphere rolls without sliding, find the frictional force (in N)
View SolutionA force is inclined at an angle of 60∘ from the horizontal. If the horizontal component of the force is 4N,calculate the vertical component.
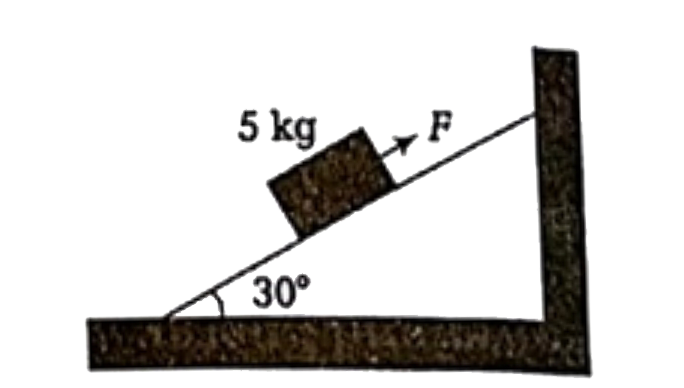
View SolutionA force F=75 N is applied on a block of mass 5 kg along the fixed smooth incline as as shown in the figure. Here gravitational acceleration g = 10 m s−2 . The acceleration of the block is
View SolutionThe greater and least resultant of two forces are 9 N and 5 N respectively. If they are applied at 60°. The magnitude of the resultant is
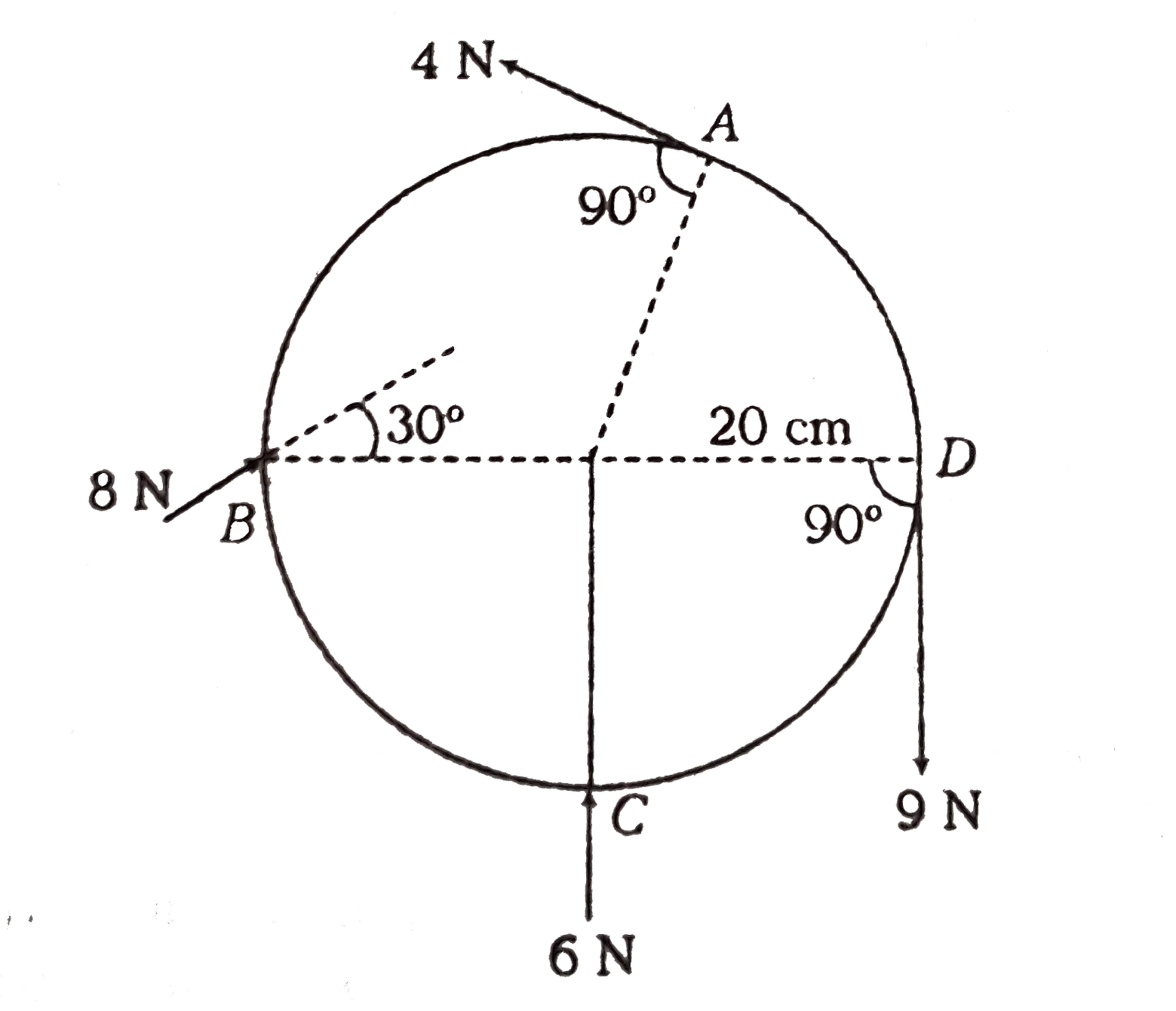
View SolutionForces are applied on a wheel of radius 20 cm as shown in the figure. The torque produced by the forces 4 N at A, 8N at B, 6 N at C and 9N at D at angles indicated is
View SolutionThe length of a spring is α when a force of 4N is applied on it and the length is β when 5N is applied. Then the length of spring when 9N force is applied is-
View SolutionTwo forces of magnitudes 3N and 4 N act together on an object making an angle 60∘ with each other. Find the resultant force acting on the object.
View Solution
