The minimum deviation produced by a hollow prism filled with a certain liquid to be . The light ray is also found to be refracted at angle . The refractive index of the liquid is
The correct Answer is:A
To find the refractive index of the liquid in the hollow prism, we can follow these steps:
Step 1: Understand the Given Information
- Minimum deviation (Δm) = 30°
- Angle of refraction (r) = 30°
Step 2: Relate the Angle of Prism (A) to the Angle of Refraction
In a prism, at minimum deviation, the angle of the prism (A) is related to the angle of refraction (r) by the formula:
A=2r
Substituting the given value of r:
A=2×30°=60°
Step 3: Use the Formula for Refractive Index (μ)
The formula for the refractive index (μ) of the prism filled with a liquid is given by:
μ=sin(A+Δm2)sin(A2)
Step 4: Substitute the Values into the Formula
Now, substituting A = 60° and Δm = 30° into the formula:
μ=sin(60°+30°2)sin(60°2)
Calculating the angles:
- 60°+30°2=90°2=45°
- 60°2=30°
Thus, we have:
μ=sin(45°)sin(30°)
Step 5: Use Known Values of Sine
Now, we can use the known values:
- sin(45°)=1√2
- sin(30°)=12
Substituting these values:
μ=1√212
Step 6: Simplify the Expression
To simplify:
μ=1√2×2=2√2=√2
Final Answer
The refractive index of the liquid in the prism is:
μ=√2
---
- Minimum deviation (Δm) = 30°
- Angle of refraction (r) = 30°
In a prism, at minimum deviation, the angle of the prism (A) is related to the angle of refraction (r) by the formula:
A=2r
Substituting the given value of r:
A=2×30°=60°
The formula for the refractive index (μ) of the prism filled with a liquid is given by:
μ=sin(A+Δm2)sin(A2)
Now, substituting A = 60° and Δm = 30° into the formula:
μ=sin(60°+30°2)sin(60°2)
Calculating the angles:
- 60°+30°2=90°2=45°
- 60°2=30°
μ=sin(45°)sin(30°)
Now, we can use the known values:
- sin(45°)=1√2
- sin(30°)=12
μ=1√212
To simplify:
μ=1√2×2=2√2=√2
The refractive index of the liquid in the prism is:
μ=√2
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (EXERCISE II LEVEL -II (ADVANCED) STRAIGHT OBJECTIVE TYPE QUESTIONS)
2 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (EXERCISE II LEVEL -II (ADVANCED) LINKED COMPREHENSION TYPE QUESTIONS)
6 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (EXERCISE I LEVEL -II (ADVANCED) INTEGER TYPE QUESTIONS)
2 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
10 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
106 Videos
Similar Questions
Knowledge Check
A ray of light is incident normally on the prism (μ=32) immersed in a liquid as shown in the figure. The largest value for the angle α so that ray is totally reflected at the face AC is 30∘. The refractive index of the given liquid is
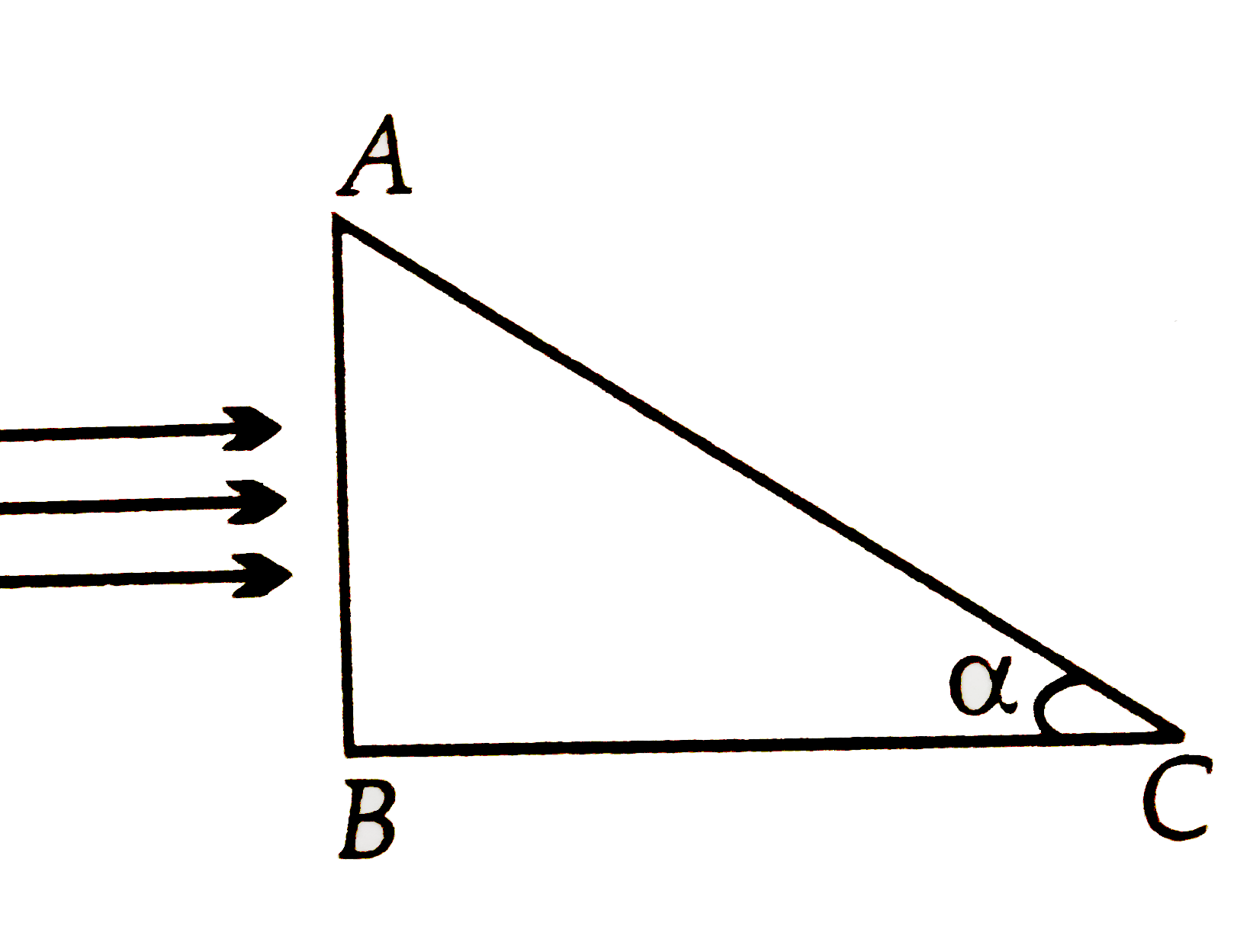 A√32B34C43D3√34
A√32B34C43D3√34
The angle of minimum deviation produced by an equilateral prism is 46∘ The refractive index of material of the prism.
View SolutionThe angle of minimum deviation for a 75∘ prism of dense glass is found to be 45∘ when in air and 15∘ when immersed in certain liquid. The refractive index of the liquid is
View SolutionA ray of monochromatic light suffers minimum deviation of 38∘ while passing through a prism of refracting angle 60∘ . Refracting index of the prism material is
View SolutionA ray of light incidents on a refracting surface at 30∘ with the surface . If the angle of refraction is 45∘ refractive index of the medium is
View SolutionA ray of light is incident at an angle of 60∘ on one face of a prism which has refracting angle of 30∘ . The ray emerging out of the prism makes an angle of 30∘ with the incident ray. If the refractive index of the material of the prism is μ=√a , find the value of a .
View SolutionA narrow beam of monochromatic light, PQ, is incident normally on one face of an equiangular glass prism of refractive index 1.45. When the prism is immersed in a certain liquid, the ray makes a grazing emergence along the other face (figure). Find the refractive index of this liquid .
View SolutionA light ray going through a prism with the angle of prism 60∘, is founded to deviate at least by 30∘. what Is the range of the refractive index of the prism?
View Solution
