A ray of monochromatic light suffers minimum deviation of 38∘ while passing through a prism of refracting angle 60∘ . Refracting index of the prism material is
The correct Answer is:3
To find the refractive index of the prism material given the minimum deviation and the refracting angle of the prism, we can follow these steps:
1. Understand the relationship between angles:
The formula for minimum deviation (D) in a prism is given by:
D=i+e−A
where i is the angle of incidence, e is the angle of emergence, and A is the refracting angle of the prism. At minimum deviation, i=e.
2. Set up the equation for minimum deviation:
Since i=e at minimum deviation, we can write:
D=2i−A
Rearranging gives:
2i=D+A⇒i=D+A2
3. Substitute the known values:
Given D=38∘ and A=60∘:
i=38∘+60∘2=98∘2=49∘
4. Calculate the angle of refraction:
The angle of refraction r can be calculated using the relationship:
r=A2=60∘2=30∘
5. Use Snell's Law to find the refractive index:
According to Snell's Law:
μ=sinisinr
Substituting the values we found:
μ=sin49∘sin30∘
6. Calculate the sine values:
We know that sin30∘=0.5. Now we need to calculate sin49∘. Using a calculator or sine table:
sin49∘≈0.7547
7. Substitute the sine values into the equation:
μ=0.75470.5=1.5094
8. Final answer:
The refractive index of the prism material is approximately:
μ≈1.51
The formula for minimum deviation (D) in a prism is given by:
D=i+e−A
where i is the angle of incidence, e is the angle of emergence, and A is the refracting angle of the prism. At minimum deviation, i=e.
Since i=e at minimum deviation, we can write:
D=2i−A
Rearranging gives:
2i=D+A⇒i=D+A2
Given D=38∘ and A=60∘:
i=38∘+60∘2=98∘2=49∘
The angle of refraction r can be calculated using the relationship:
r=A2=60∘2=30∘
According to Snell's Law:
μ=sinisinr
Substituting the values we found:
μ=sin49∘sin30∘
We know that sin30∘=0.5. Now we need to calculate sin49∘. Using a calculator or sine table:
sin49∘≈0.7547
μ=0.75470.5=1.5094
The refractive index of the prism material is approximately:
μ≈1.51
Similar Questions
The angle of minimum deviation measured with a prism is 30∘ and the angle of prism is 60∘. The refractive index of prism material is
View SolutionA ray of light suffers a minimum deviation when incident on equilateral prism of refractive index √2 The angle of deviation is
View SolutionThe angle of minimum deviation for an incident ligt ray on an equillateral prism is equal to its refracting angle. The refractive index of its material is
View SolutionA ray of light undergoes deviation of 30∘ when incident on an equilateral prism of refractive index √2. The angle made by the ray inside the prism with the base of the prism is
View SolutionIn the diagram the ray passing through prism is parallel to the base. Refractive index of material of prism is
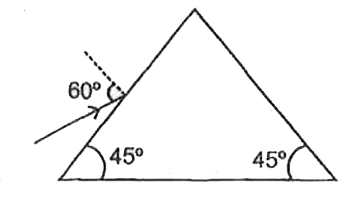
View SolutionA ray of monochromatic light is incident on one refracting face of a prism of angle 75∘. It passes thorugh the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is √2, the angle of incidence on the first face of the prism is
View SolutionA ray OP of monochromatic light is incident on the face AB of prism ABCD mear vertex B at an incident angle of 60degree (see figure). If the refractive index of the material of the prism is √3, which of the following is (are) are correct?

View SolutionA ray of light falling at an angle of 50∘ is refracted through a prism and suffers minimum deviation. The angle of the prism is 60∘. Find the angle of minimum deviation and refraction index of the material of the prism.
View SolutionA ray of monochromatic light is incident on the refracting face of a prism (angle 75∘0 . It passes through the prism and is incident on the other face at the critical angle. If the refractive index of the prism is √2 , then the angle of incidence on the first of the prism is
View SolutionThe angle of minimum deviation from a prism is 37∘. If the angle of prism is 53∘, find the refractive index of the material of the prism.
View Solution