A ray of light falling at an angle of 50∘ is refracted through a prism and suffers minimum deviation. The angle of the prism is 60∘. Find the angle of minimum deviation and refraction index of the material of the prism.
To solve the problem, we will follow these steps:
Step 1: Understand the relationship for minimum deviation
In the case of a prism, when light passes through it at minimum deviation, the relationship between the angle of incidence (i), the angle of the prism (A), and the angle of minimum deviation (D) is given by the formula:
D=2i−A
Step 2: Substitute the known values
We know:
- Angle of incidence, i=50∘
- Angle of prism, A=60∘
Substituting these values into the formula:
D=2(50∘)−60∘
D=100∘−60∘
D=40∘
Step 3: Calculate the refractive index of the prism
The refractive index (μ) of the prism material can be calculated using the formula:
μ=sin(D+A2)sin(A2)
Step 4: Substitute the values into the refractive index formula
We already found D=40∘ and A=60∘. Now we can calculate:
- D+A2=40∘+60∘2=100∘2=50∘
- A2=60∘2=30∘
Now substituting these into the refractive index formula:
μ=sin(50∘)sin(30∘)
Step 5: Calculate the sine values
We know:
- sin(30∘)=12
- sin(50∘) can be calculated using a calculator or trigonometric tables, which is approximately 0.766.
Step 6: Substitute the sine values into the equation
μ=0.7660.5
μ=1.532
Final Results
- Angle of minimum deviation D=40∘
- Refractive index of the prism material μ≈1.53
In the case of a prism, when light passes through it at minimum deviation, the relationship between the angle of incidence (i), the angle of the prism (A), and the angle of minimum deviation (D) is given by the formula:
D=2i−A
We know:
- Angle of incidence, i=50∘
- Angle of prism, A=60∘
D=2(50∘)−60∘
D=100∘−60∘
D=40∘
The refractive index (μ) of the prism material can be calculated using the formula:
μ=sin(D+A2)sin(A2)
We already found D=40∘ and A=60∘. Now we can calculate:
- D+A2=40∘+60∘2=100∘2=50∘
- A2=60∘2=30∘
μ=sin(50∘)sin(30∘)
We know:
- sin(30∘)=12
- sin(50∘) can be calculated using a calculator or trigonometric tables, which is approximately 0.766.
μ=0.7660.5
μ=1.532
- Angle of minimum deviation D=40∘
- Refractive index of the prism material μ≈1.53
Similar Questions
The angle of minimum deviation from a prism is 37∘. If the angle of prism is 53∘, find the refractive index of the material of the prism.
View SolutionThe angle of incidence for a ray of light at a refracting surface of a prism is 45∘. The angle of prism is 60∘. If the ray suffers minimum deviation through the prism, the angle of minimum deviation and refractive index of the material of the prism respectively, are :
View SolutionIf the angle of prism is 60∘ and the angle of minimum deviation is 40∘ , the angle of refraction will be
View SolutionThe angle of minimum deviation measured with a prism is 30∘ and the angle of prism is 60∘. The refractive index of prism material is
View SolutionFor refraction through a small angled prism, the angle of minimum deviation
View SolutionA thin prism of angle A=6∘ produces a deviation d=3∘. Find the refractive index of the material of prism.
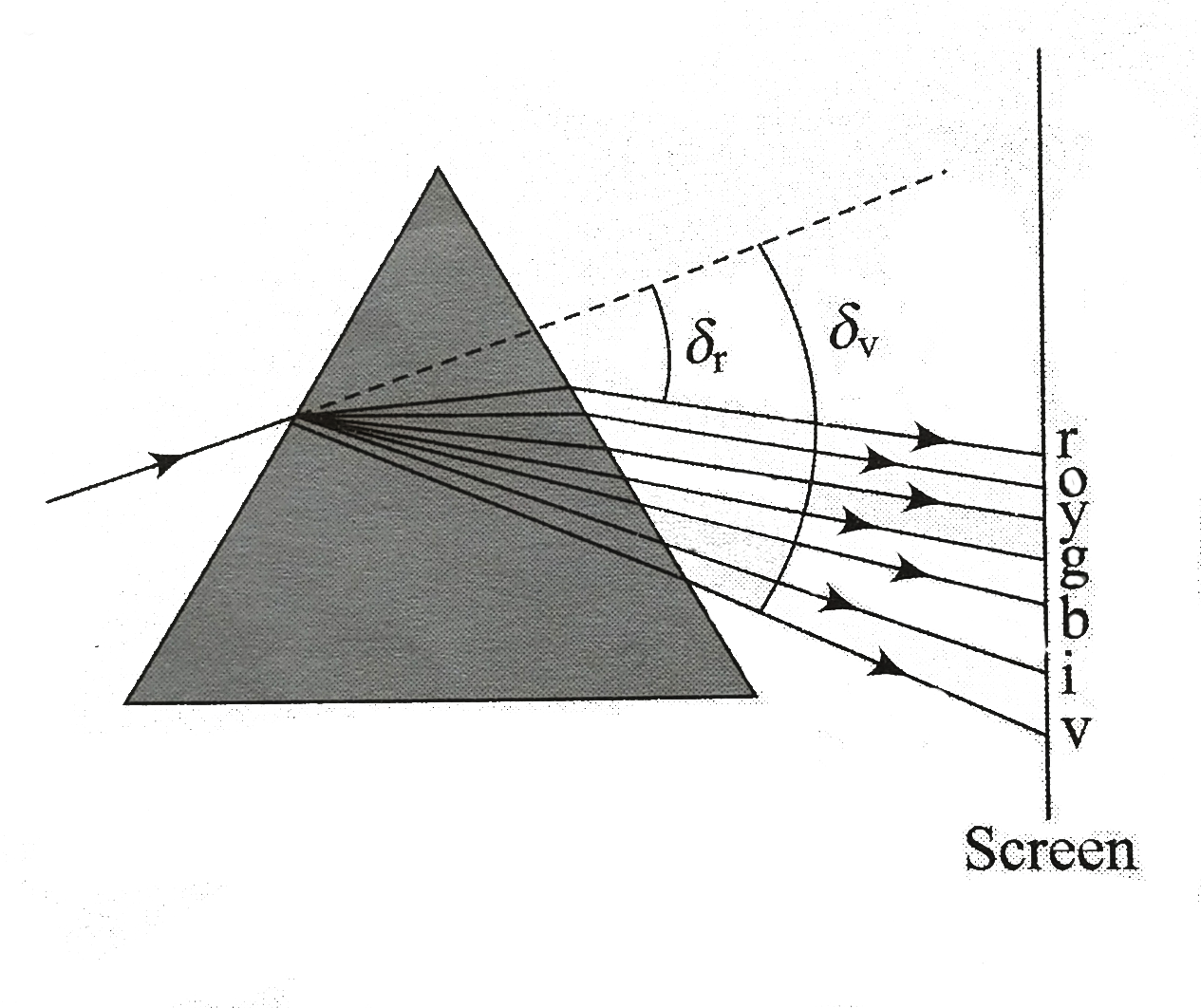
View SolutionThe refracting angle of a prism is A, and refractive index of the material of the prism is cot(A2). The angle of minimum deviation is
View SolutionFor a ray of light refracted through a prism of angle 60∘, the angle of incidence is equal to the angle of emergence, each equal to 45∘. Find the refractive index of the material of the prism.
View SolutionIf the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionA ray of light incident at an angle of incidence 48∘ on a prism of refracting angle 60∘ suffers minimum deviation. Calculate the angle of minimum deviation.
View Solution
