A 2kg block is placed over a 5kg block and both are placed on a smooth horizontal surface. The coefficient of friction between the blocks is 0.10. Find the acceleration of the two blocks if a horizontal force of 14N is applied to the upper block (g = 10ms−2).
To solve the problem, we will follow these steps:
Step 1: Identify the forces acting on the upper block (2 kg)
The applied force on the upper block is 14 N. The frictional force acting between the two blocks will oppose the motion. The maximum static friction force can be calculated using the formula:
Fmaxs=μ⋅m1⋅g
where:
- μ=0.10 (coefficient of friction)
- m1=2kg (mass of the upper block)
- g=10m/s2 (acceleration due to gravity)
Step 2: Calculate the maximum static friction force
Substituting the values:
Fmaxs=0.10⋅2kg⋅10m/s2=2N
Step 3: Determine if the applied force exceeds the maximum static friction
The applied force (14 N) is greater than the maximum static friction (2 N). Therefore, the upper block will slide over the lower block.
Step 4: Write the equation of motion for the upper block (2 kg)
For the upper block, the net force acting on it is:
Fnet=Fapplied−Fs
where Fs is the frictional force (which will be equal to the maximum static friction force since it is the only friction acting). Thus, we can write:
2kg⋅a1=14N−2N
This simplifies to:
2a1=12
a1=6m/s2
Step 5: Write the equation of motion for the lower block (5 kg)
The only force acting on the lower block is the frictional force from the upper block:
Fnet=Fs
Thus, we can write:
5kg⋅a2=2N
This simplifies to:
a2=25=0.4m/s2
Final Results
- The acceleration of the upper block (2 kg) is 6m/s2.
- The acceleration of the lower block (5 kg) is 0.4m/s2.
The applied force on the upper block is 14 N. The frictional force acting between the two blocks will oppose the motion. The maximum static friction force can be calculated using the formula:
Fmaxs=μ⋅m1⋅g
where:
- μ=0.10 (coefficient of friction)
- m1=2kg (mass of the upper block)
- g=10m/s2 (acceleration due to gravity)
Substituting the values:
Fmaxs=0.10⋅2kg⋅10m/s2=2N
The applied force (14 N) is greater than the maximum static friction (2 N). Therefore, the upper block will slide over the lower block.
For the upper block, the net force acting on it is:
Fnet=Fapplied−Fs
where Fs is the frictional force (which will be equal to the maximum static friction force since it is the only friction acting). Thus, we can write:
2kg⋅a1=14N−2N
This simplifies to:
2a1=12
a1=6m/s2
The only force acting on the lower block is the frictional force from the upper block:
Fnet=Fs
Thus, we can write:
5kg⋅a2=2N
This simplifies to:
a2=25=0.4m/s2
- The acceleration of the upper block (2 kg) is 6m/s2.
- The acceleration of the lower block (5 kg) is 0.4m/s2.
Similar Questions
A 2 kg block iks placed over a 4 kg block and both are plced on a smooth horizontal surfae. The coefficient of friction between the blocks is 0.20. Find theacceleration of the two blocks if a horizontal force os 12 N is applied to a. the upper block, b. the lower block. Take g=10ms2.
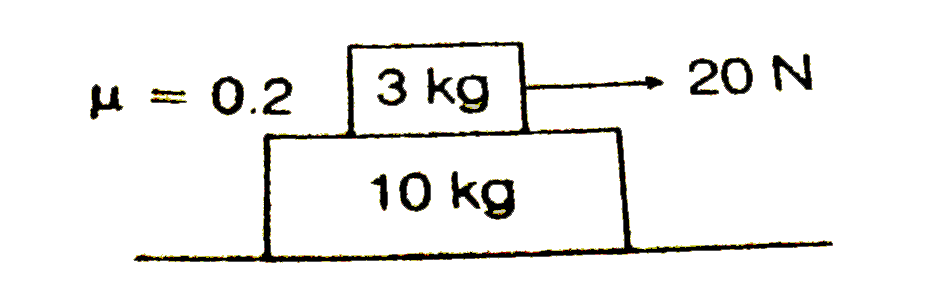
View SolutionA 3 kg block is placed over a 10 kg block and both are palced on a smooth horizontal surface. The coefficient of friction between the block is 0.2 . If a horizontal force of 20 N is applied to 3 kg block, accelerations of the two block in ms−2 are (take, g=ms−2)
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
A10NB5NC2.5ND20N
A block of mass m is kept over another block of mass 2 m and the system rests on a smooth horizontal surface. The coefficient of friction between the blocks is 0.50. Find the work done by the force of friction on the smaller block by the bigger block during a displacement d of the system, when a force mg is applied to the lower block.
View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionA block of mass m is placed on another block of mass M lying on a smooth horizontal surface as shown in the figure. The co-efficient of friction between the blocks is μ. Find the maximum horizontal force F (in Newton) that can be applied to the block M so that the blocks together with same acceleration? (M=3kg,m=2kg,μ=0.1,g=10m/s2)
 View Solution
View SolutionA block of mass 1kg lies on a horizontal surface in a truck. The coefficeint of friction between the block and surface is 0.5. If the acceleration of the truck is 6ms−2 the acceleration of the block relative to ground is
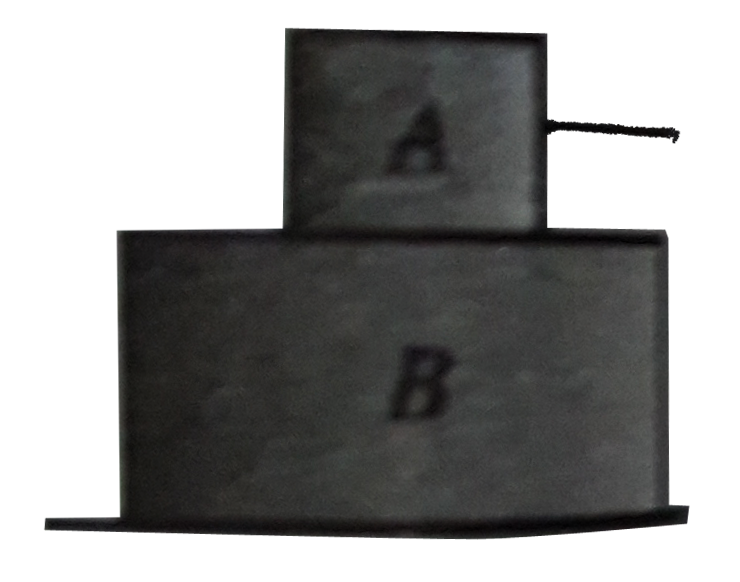
View SolutionA block A of mass 2kg is placed over smooth block B of mass 4kg which is placed over a smooth horizontal floor The coefficient of friction between A and B is 0.4 when a horizontal forces of magnitude 10N is applied on A the acceleration of block of A and B are
 View Solution
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View Solution
