A shell is fired from a long - range gun with an intial velocity u=1000ms−1 at an angle 30∘ to the horizontal. How long with the shell be in the air ? At what distance from the gun will it fall to ground ? The gun and the point where the shell lands are on the same horizontal line .
To solve the problem step by step, we will break it down into two parts: finding the time of flight and then finding the horizontal range.
Step 1: Finding the Time of Flight
The formula for the time of flight T of a projectile is given by:
T=2usinθg
Where:
- u=1000m/s (initial velocity)
- θ=30∘ (angle of projection)
- g=10m/s2 (acceleration due to gravity)
First, we need to calculate sin30∘:
sin30∘=12
Now substituting the values into the time of flight formula:
T=2×1000×1210
Simplifying this gives:
T=100010=100seconds
Step 2: Finding the Horizontal Range
The formula for the horizontal range R of a projectile is given by:
R=u2sin2θg
We need to calculate sin2θ:
sin2θ=sin60∘=√32
Now substituting the values into the range formula:
R=10002⋅√3210
Calculating 10002:
10002=106
Now substituting this back into the equation:
R=106⋅√3210
This simplifies to:
R=105⋅√31
Now calculating R:
R≈105⋅1.732=173200m
Final Answers
- Time of flight T=100seconds
- Horizontal range R≈173200meters
- u=1000m/s (initial velocity)
- θ=30∘ (angle of projection)
- g=10m/s2 (acceleration due to gravity)
- Horizontal range R≈173200meters
Topper's Solved these Questions
- MOTION IN A PLANEBook:AAKASH SERIESChapter:MOTION IN A PLANEExercise:NUMERICAL EXERCISE (LEVEL -2)
Explore 19 Videos
- MOTION IN A PLANEBook:AAKASH SERIESChapter:MOTION IN A PLANEExercise:QUESTION FOR DESCRIPTIVE ANSWER
Explore 7 Videos
- MOTION IN A PLANEBook:AAKASH SERIESChapter:MOTION IN A PLANEExercise:VERY SHORT ANSWER TYPE QUESTIONS
Explore 10 Videos
- MOTION IN A PLANEBook:AAKASH SERIESChapter:MOTION IN A PLANEExercise:EXERCISE-3 (Circular Motion)
Explore 7 Videos
- MOTION IN A STRAIGHT LINE Book:AAKASH SERIESChapter:MOTION IN A STRAIGHT LINE Exercise:very Short answer type question
Explore 15 Videos
Similar Questions
Knowledge Check
The bullet weighing 100g is released from the barrel of an air gun with a velocity of 4ms−1. Calculate the potential energy of the spring.
A0.8 JB800 JC8 JD0.08J
A bomb is fired from a cannon with a velocity of 1000ms−1 making an angle of 30∘ with the horizontal (g=9.8m/s2).
At what distance from the cannon the bomb will hit the ground?
View SolutionA shell is fired from a cannon, it explodes in mid air, its total
View SolutionA bomb is fired from a cannon with a velocity of 1000ms−1 making an angle of 30∘ with the horizontal (g=9.8m/s2).
With what speed the bomb will hit the ground and what will be it direction of motion while hitting?
View SolutionA body is thrown with the velocity u=12.0ms−1 at an angle of α=45∘ to the horizon. If it touches the ground at the distance s from the point where it was thrown. From what height h should stone be thrown in a horizontal direction with the same initial velocity u so that it falls at the same spot ?
View SolutionA bullet of mass 50 g is fired from a gun with initial velocity of 35 m/s. If mass of the gun is 4 kg, then calculate the recoil velocity of the gun.
View SolutionA shell is fired from a gun from the bottom of a hill along its slope. The slope of the hill is ∝=30∘ and the angle of the barrel to the horizontal β=60∘. The initial velocity v of the shell is 21ms−1. Then find the distance of point from the gun at which the shell will fall.
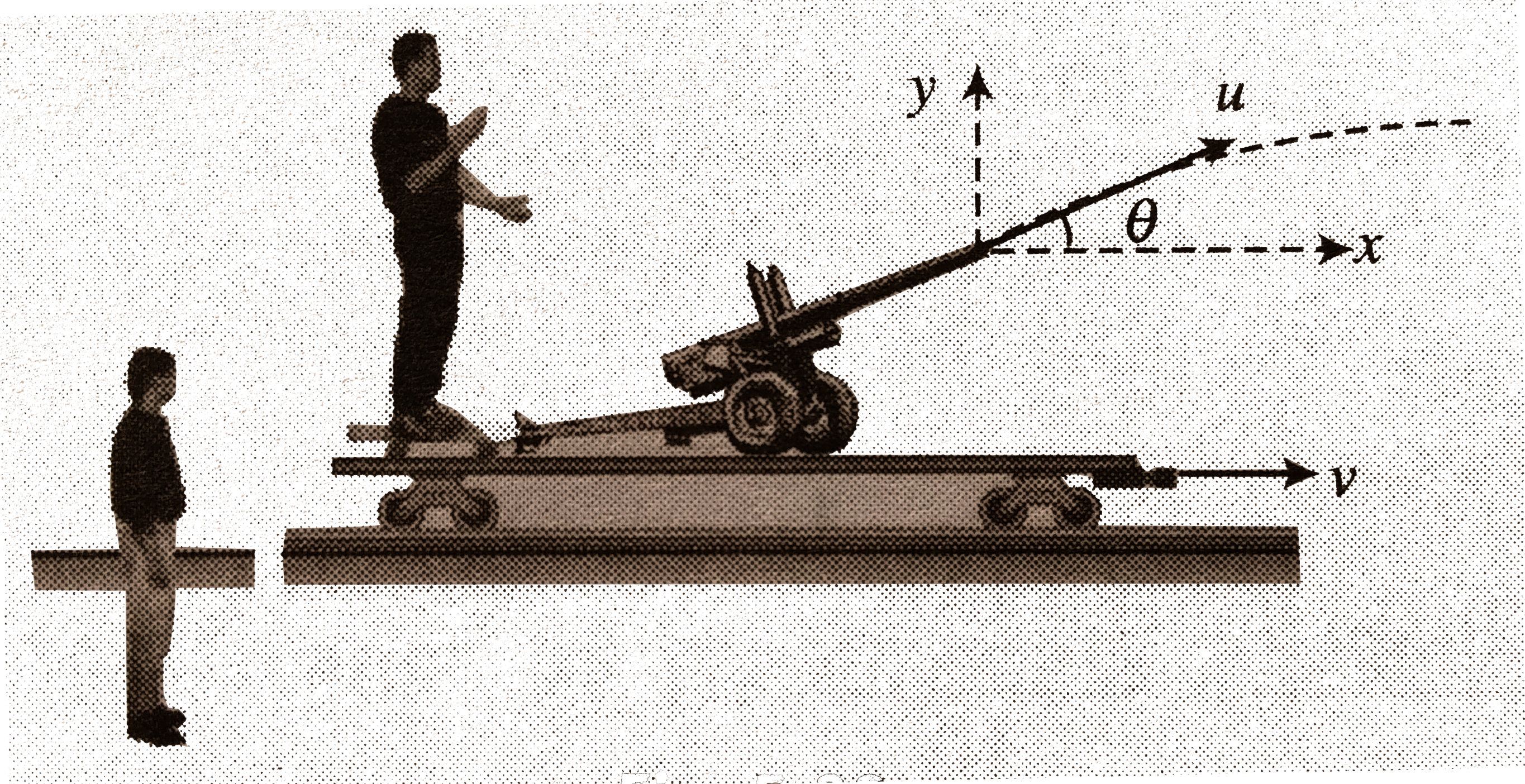
View SolutionA shell is projected from a gun with a muzzle velocity, u. The gun is fitted with a trolly car at an angle θ as shown in (Fig. 5.33). If the trolley car is made to move with constant velocity v towards right, find the
(a) horizontal range of the shell relative to ground.
(b) horizontal range of the shell relative to a person travelling with trolley.
 .
.
View Solution