A particle projected with velcoity v0 strikes at right α plane through the point of projection .
To solve the problem of a particle projected with an initial velocity v0 striking a plane inclined at an angle α, we can follow these steps:
Step 1: Understand the Problem
We have a particle projected at an angle α to the horizontal. We need to analyze its motion and determine the conditions under which it strikes the inclined plane.
Step 2: Break Down the Initial Velocity
The initial velocity v0 can be broken down into two components:
- Horizontal component: v0x=v0cos(α)
- Vertical component: v0y=v0sin(α)
Step 3: Determine the Equation of Motion
The particle is subject to gravity, which affects its vertical motion. The equations of motion for the vertical and horizontal components can be expressed as:
- Horizontal motion: x=v0xt=v0cos(α)t
- Vertical motion: y=v0yt−12gt2=v0sin(α)t−12gt2
Step 4: Find the Equation of the Inclined Plane
The equation of the inclined plane can be expressed as:
y=xtan(α)
This represents the line along which the particle will strike the plane.
Step 5: Set the Two Equations Equal
To find the time t when the particle strikes the plane, we set the vertical motion equation equal to the equation of the inclined plane:
v0sin(α)t−12gt2=v0cos(α)ttan(α)
Step 6: Simplify and Solve for t
Rearranging gives:
v0sin(α)t−v0sin(α)t=12gt2
This simplifies to:
12gt2=0
This indicates that the time of flight can be calculated using the quadratic formula if we set the equation properly.
Step 7: Calculate the Time of Flight
From the equation derived, we can solve for t:
t=2v0sin(α)g
Step 8: Find the Distance Traveled
Now, substituting t back into the horizontal motion equation:
x=v0cos(α)(2v0sin(α)g)
This gives us the horizontal distance traveled before striking the plane.
Step 9: Conclusion
The particle will strike the inclined plane at a distance x from the point of projection, which can be calculated using the above equations.
We have a particle projected at an angle α to the horizontal. We need to analyze its motion and determine the conditions under which it strikes the inclined plane.
The initial velocity v0 can be broken down into two components:
- Horizontal component: v0x=v0cos(α)
- Vertical component: v0y=v0sin(α)
The particle is subject to gravity, which affects its vertical motion. The equations of motion for the vertical and horizontal components can be expressed as:
- Horizontal motion: x=v0xt=v0cos(α)t
- Vertical motion: y=v0yt−12gt2=v0sin(α)t−12gt2
The equation of the inclined plane can be expressed as:
y=xtan(α)
This represents the line along which the particle will strike the plane.
To find the time t when the particle strikes the plane, we set the vertical motion equation equal to the equation of the inclined plane:
v0sin(α)t−12gt2=v0cos(α)ttan(α)
Rearranging gives:
v0sin(α)t−v0sin(α)t=12gt2
This simplifies to:
12gt2=0
This indicates that the time of flight can be calculated using the quadratic formula if we set the equation properly.
From the equation derived, we can solve for t:
t=2v0sin(α)g
Now, substituting t back into the horizontal motion equation:
x=v0cos(α)(2v0sin(α)g)
This gives us the horizontal distance traveled before striking the plane.
The particle will strike the inclined plane at a distance x from the point of projection, which can be calculated using the above equations.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - SHORT ANSWER TYPE QUESTIONS
6 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
Similar Questions
A particle projected with velcoity v0 strikes at right α plane through the point of projection .
View SolutionA particle is projected vertically upward with velocity u from a point A, when it returns to the point of projection .
View SolutionA ball is projected obliquely with a velocity 49 ms−1 strikes the ground at a distance of 245 m from the point of projection. It remained in air for
View SolutionA particle of mass'm' is projected with velocity v0 at an angle 'α' with the horizontal. The coefficient of restitution for any of its impact with the smooth ground is e.
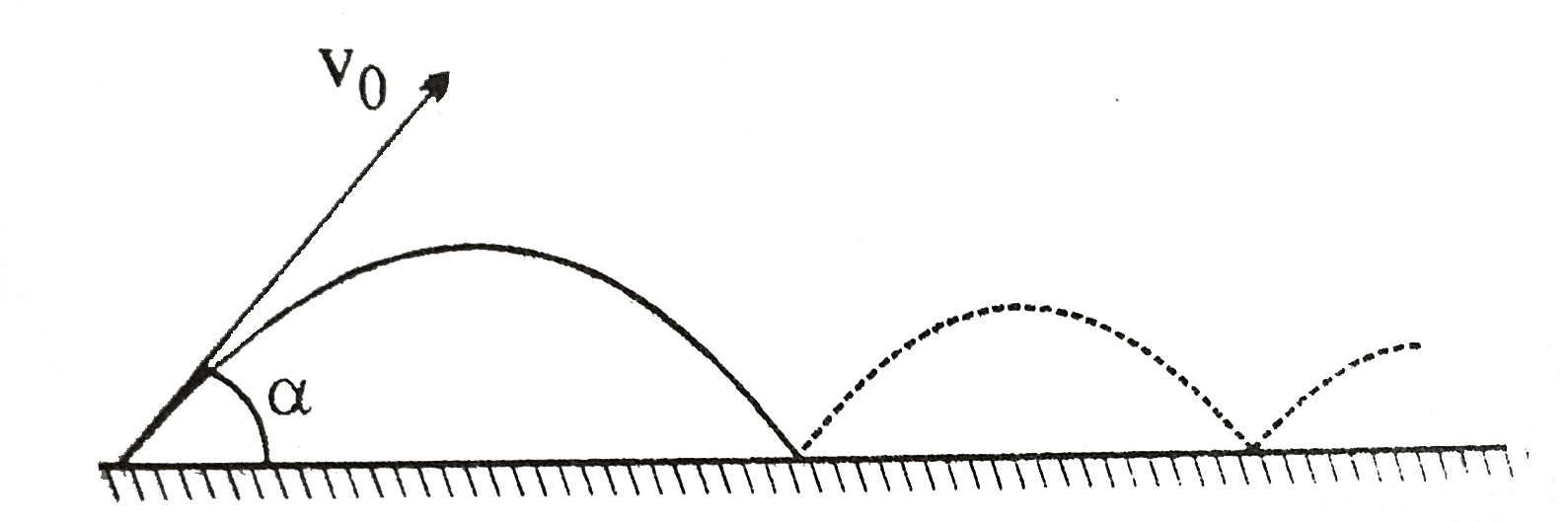
Find total time taken by the particle before it stops moving vertically?
View SolutionA particle is projected with a certain velocity at an angle α above the horizontal from the foot of an inclined plane of inclination 30∘. If the particle strikes the plane normally then α is equal to
View SolutionA particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View SolutionA particle is projected with a velocity ¯v=aˆi+bˆj . Find the radius of curvature of the trajectory of the particle at (i) point of projection (ii) highest point .
View SolutionA particle is projected with velocity v at an angle θ aith horizontal. The average angle velocity of the particle from the point of projection to impact equals
View SolutionA ball is projected from a point in a horizontal plane so as to strike a vertical wall at right angle A.after rebounding from the wall it strikes the horizontal plane once and returns to the point of projection just before second collision at horizontal surface. Find co-efficient of restitution for the two collisions, assuming it to be same for both the collision.
View SolutionAn object is projected horizontally from a top of the tower of height h. The line joining the point of projection and point of striking on the ground makes an angle 45∘ with ground, then with what velocity the object strikes the ground
View Solution
