A thin prism of 5∘ angle gives a deviation of 3.2∘. What is the value of refraction index of the material of the prism?
μ = 2.12
μ = 1.71
μ = 1.64
μ = 3.11
The correct Answer is:C
To find the refractive index (μ) of the material of the prism, we can use the formula that relates the angle of the prism (A), the angle of deviation (D), and the refractive index:
μ−1=DA
Where:
- μ is the refractive index,
- D is the angle of deviation,
- A is the angle of the prism.
Given:
- Angle of prism, A=5∘
- Angle of deviation, D=3.2∘
Step 1: Substitute the values into the formula
We can substitute the given values into the formula:
μ−1=3.25
Step 2: Calculate the right-hand side
Now, we calculate the right-hand side:
μ−1=0.64
Step 3: Solve for μ
Now, we can solve for μ:
μ=1+0.64
μ=1.64
Conclusion
The value of the refractive index of the material of the prism is μ=1.64.
---
- μ is the refractive index,
- D is the angle of deviation,
- A is the angle of the prism.
- Angle of prism, A=5∘
- Angle of deviation, D=3.2∘
We can substitute the given values into the formula:
Now, we calculate the right-hand side:
Now, we can solve for μ:
The value of the refractive index of the material of the prism is μ=1.64.
Similar Questions
Knowledge Check
A thin prism of 6∘ angle gives a deviation of 3∘. The refractive index of the material of the prism is.
A1B4/3C3/2D2A prism of angle4∘ gives a deviation of 2.4∘. The refractive index of the material of the prism is
A1.5B1.55C1.6D1.8If the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
A1.41B1.5C1.6D1.73
A thin prism of angle A=6∘ produces a deviation d=3∘. Find the refractive index of the material of prism.
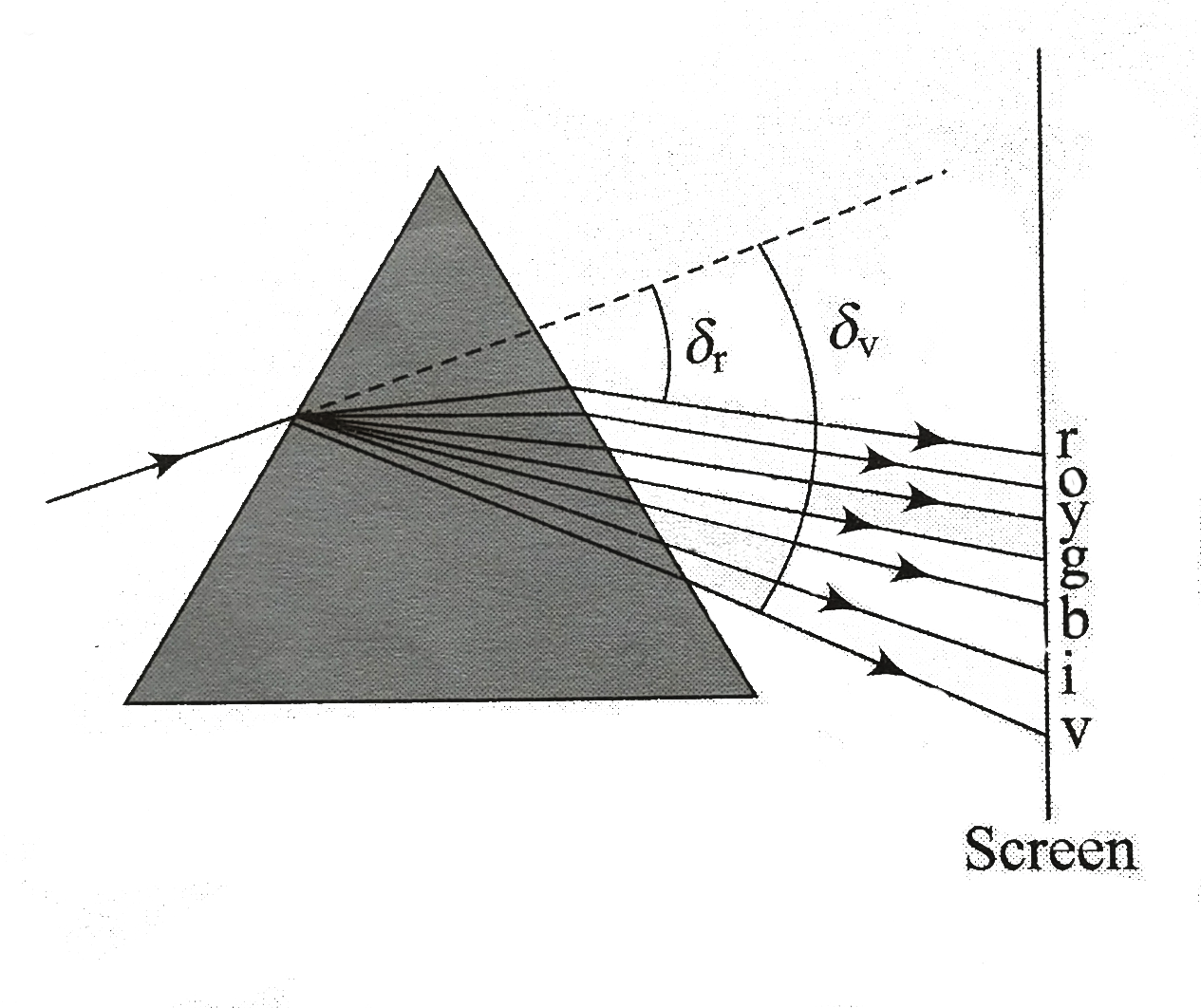
View SolutionA ray of light suffers a deviation of 3.2∘ on passing through a thin prism of angle 5∘. What is the refractive index of the material of the prism ?
View SolutionThe angle of minimum deviation caused by a prism is equal to the angle of the prism. What are the possible values of refractive index of the material of the prism?
View SolutionA ray of light on passing through an equilateral glass prism, suffers a minimum deviation equal to the angle of the prism. The value of refractive index of the material of the prism is.......
View SolutionThe angle of the prism is A and B if the angle of minimum deviation is (180∘−2A) then the refractive index of the material of the prism is
View Solution
