Question

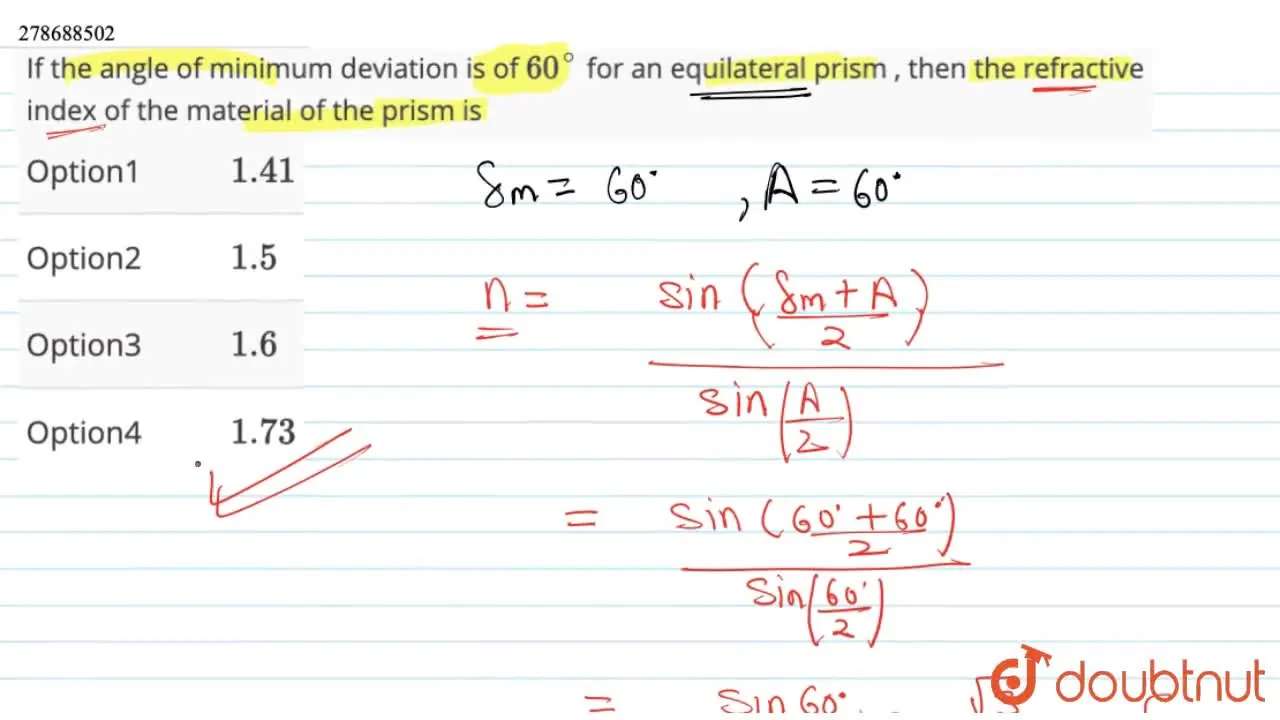
If the angle of minimum deviation is of ${60}^{\circ }$ for an equilateral prism , then the refractive index of the material of the prism is

Options

A. `1.41`
B. `1.5`
C. `1.6`
D. `1.73`

Answer

D

Explanation

To find the refractive index of an equilateral prism given the angle of minimum deviation, we can follow these steps:

Step 1: Understand the given information
- The angle of minimum deviation (D) is given as \(60^\circ\).
- The angle of the equilateral prism (A) is \(60^\circ\).

Step 2: Write the formula for the refractive index (n)
The formula for the refractive index of a prism in terms of the angle of minimum deviation and the prism angle is given by:

\( n = \frac{\sin\left(\frac{D + A}{2}\right)}{\sin\left(\frac{A}{2}\right)} \)

Step 3: Substitute the values into the formula
Substituting \(D = 60^\circ\) and \(A = 60^\circ\):

\( n = \frac{\sin\left(\frac{60^\circ + 60^\circ}{2}\right)}{\sin\left(\frac{60^\circ}{2}\right)} \)

Step 4: Simplify the expression
Calculating the angles:

\( n = \frac{\sin\left(\frac{120^\circ}{2}\right)}{\sin\left(\frac{60^\circ}{2}\right)} = \frac{\sin(60^\circ)}{\sin(30^\circ)} \)

Step 5: Use known values of sine
We know:

- \(\sin(60^\circ) = \frac{\sqrt{3}}{2}\)
- \(\sin(30^\circ) = \frac{1}{2}\)

Substituting these values into the equation:

\( n = \frac{\frac{\sqrt{3}}{2}}{\frac{1}{2}} = \frac{\sqrt{3}}{2} \times \frac{2}{1} = \sqrt{3} \)

Step 6: Calculate the numerical value
The numerical value of \(\sqrt{3}\) is approximately \(1.732\).

Step 7: Conclusion
Thus, the refractive index of the material of the prism is:

\( n \approx 1.732 \)

Final Answer
The refractive index of the prism is \(1.732\).

---