The radius of spherical capacitor when capacitance is 1μF is:
1⋅11m
9 km
10 m
1⋅11cm
The correct Answer is:B
To find the radius of a spherical capacitor with a capacitance of 1μF, we can use the formula for the capacitance of a spherical capacitor. The capacitance C of a spherical capacitor is given by the formula:
C=4πϵ0r1
where:
- C is the capacitance,
- r is the radius of the spherical capacitor,
- ϵ0 is the permittivity of free space, approximately 8.85×10−12F/m.
Step 1: Rearranging the formula for r
From the capacitance formula, we can rearrange it to solve for the radius r:
r=C4πϵ0
Step 2: Substituting the values
Now we can substitute the given value of capacitance C=1μF=1×10−6F and the value of ϵ0:
r=1×10−64π(8.85×10−12)
Step 3: Calculating the denominator
Calculating the denominator:
4πϵ0≈4×3.14×8.85×10−12≈1.112×10−10F
Step 4: Completing the calculation
Now substituting this back into our equation for r:
r=1×10−61.112×10−10≈8.99×103m
Step 5: Converting to kilometers
To convert meters to kilometers, we divide by 1000:
r≈8.99km
Final Result
Thus, the radius of the spherical capacitor is approximately 9km.
- C is the capacitance,
- r is the radius of the spherical capacitor,
- ϵ0 is the permittivity of free space, approximately 8.85×10−12F/m.
Topper's Solved these Questions
- SAMPLE PAPER 3Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 3Exercise:SECNTION -B
Explore 24 Videos
- SAMPLE PAPER 3Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 3Exercise:SECNTION -C
Explore 6 Videos
- SAMPLE PAPER 2Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 2Exercise:SECTION-C
Explore 6 Videos
- SAMPLE PAPER 5Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 5Exercise:SECTION - C
Explore 6 Videos
Similar Questions
A circuit has section AB as shown in The emf of the cell is 10V, and the capacitors have capacitances C1=1μF and C2=2μF.
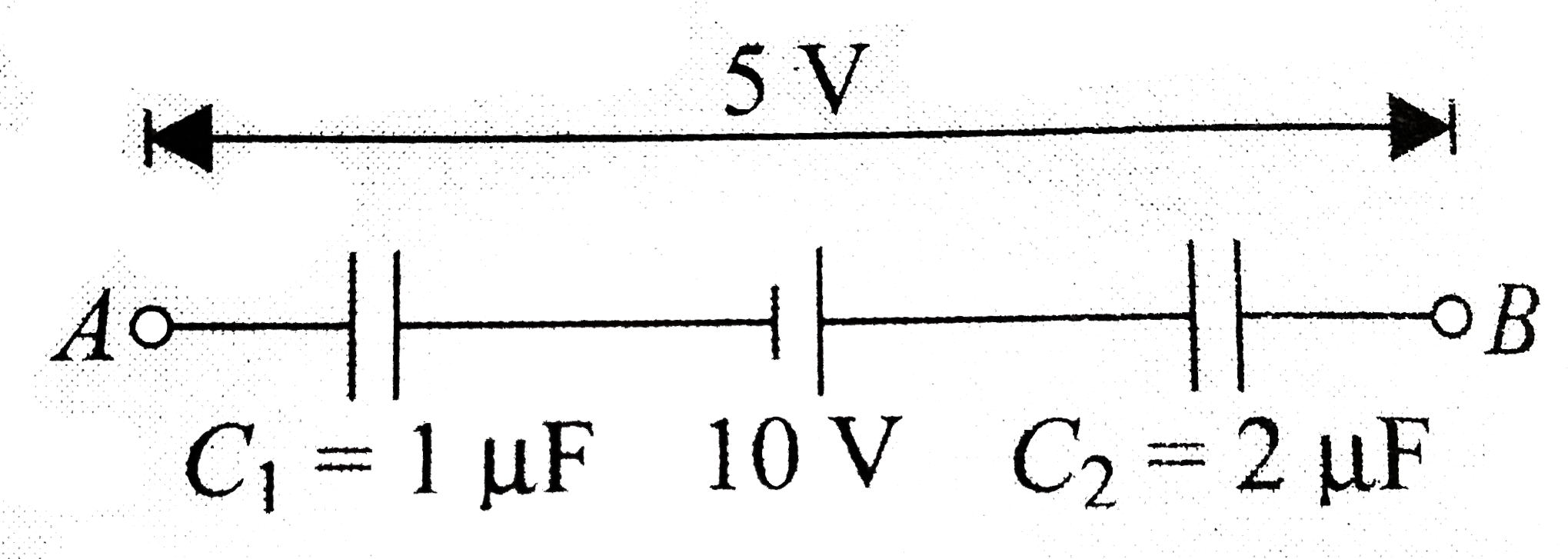 .
.
View SolutionHow many different values of capacitances can be obtained with the three given capacitors of capacitances 1μF,3μFand5μF? Use all the capacitors while forming any combination.
View Solution
Knowledge Check
An infinite number of identical capacitors each of capacitance 1μF are connected 1μF are connected as shown in Fig. Then the equivalent capacitance between A and B is
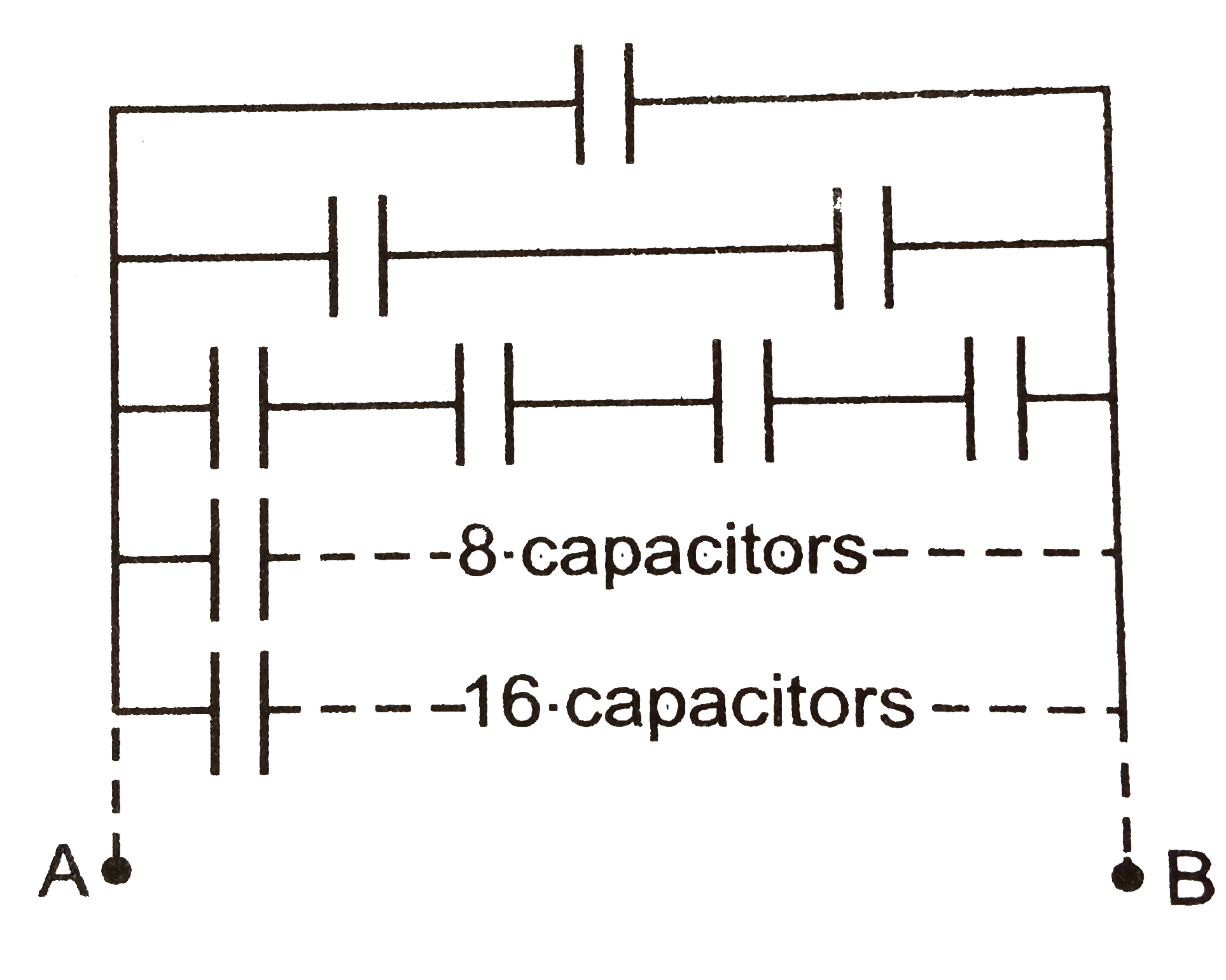 A1μFB2μFC12μFD∞
A1μFB2μFC12μFD∞In the circuit shown in figure, the charge stored on a capacitor of capacitance 3μF is
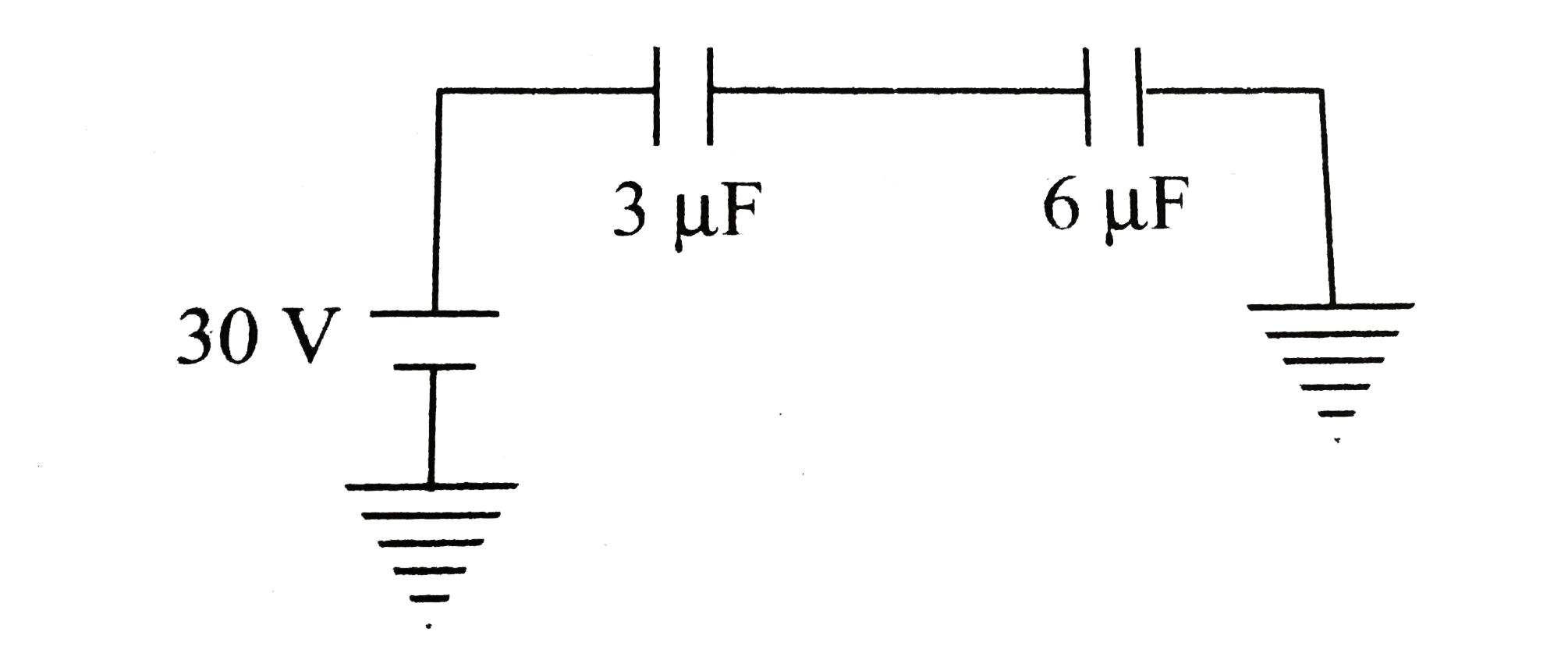 AzeroB40μCC60μCD90μC
AzeroB40μCC60μCD90μCShows a part of a circuit. If all the capacitors have a capacitance of 2μF, then the
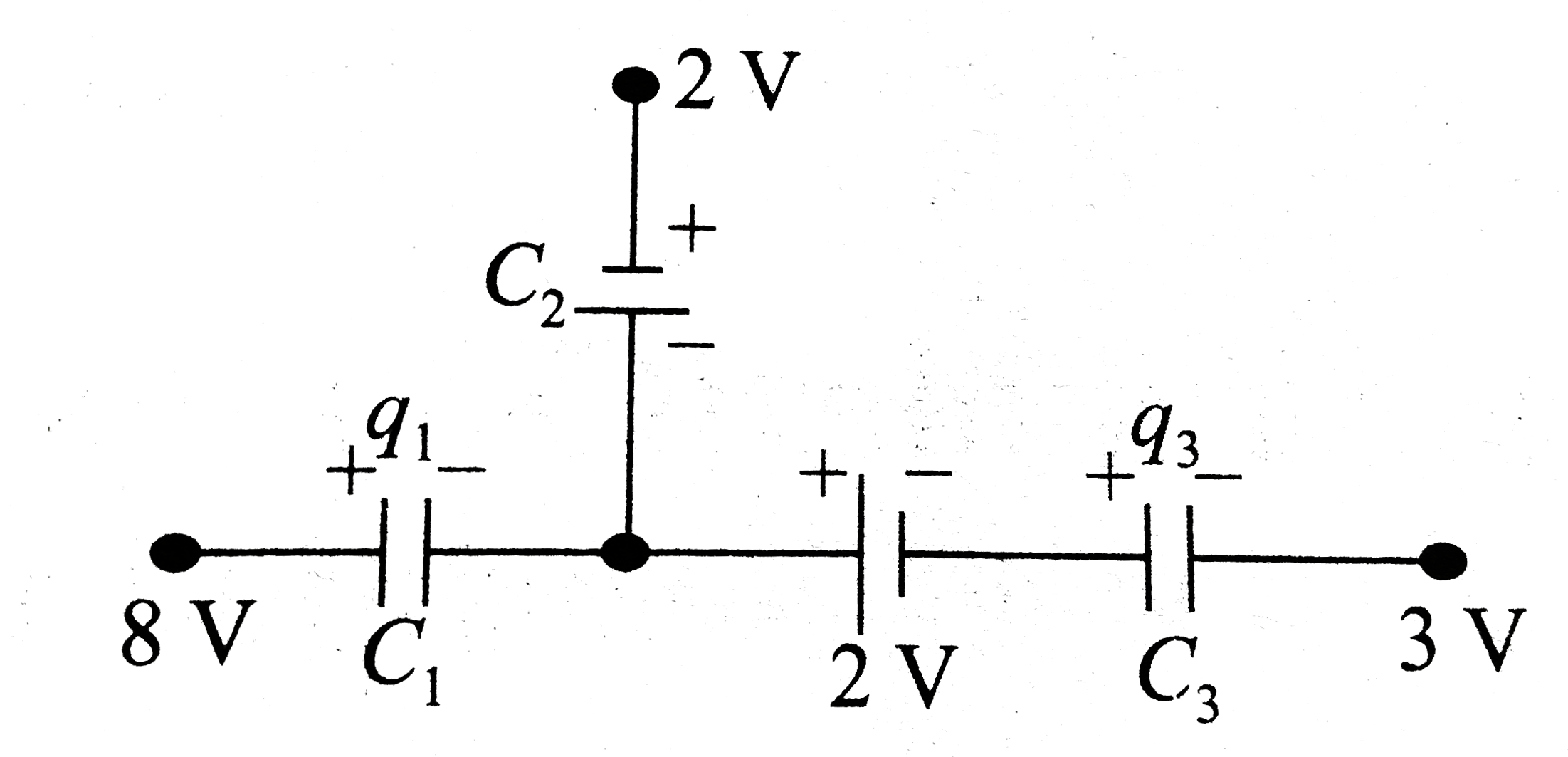 . Acharge on C3 is zeroBcharge on C3 is 12μCCcharge on C1 is 6μCDcharge on C1 in 6μC
. Acharge on C3 is zeroBcharge on C3 is 12μCCcharge on C1 is 6μCDcharge on C1 in 6μC
In the shown network, find the charges on capacitors of capacitances 5μF and 3μF, in steady state.
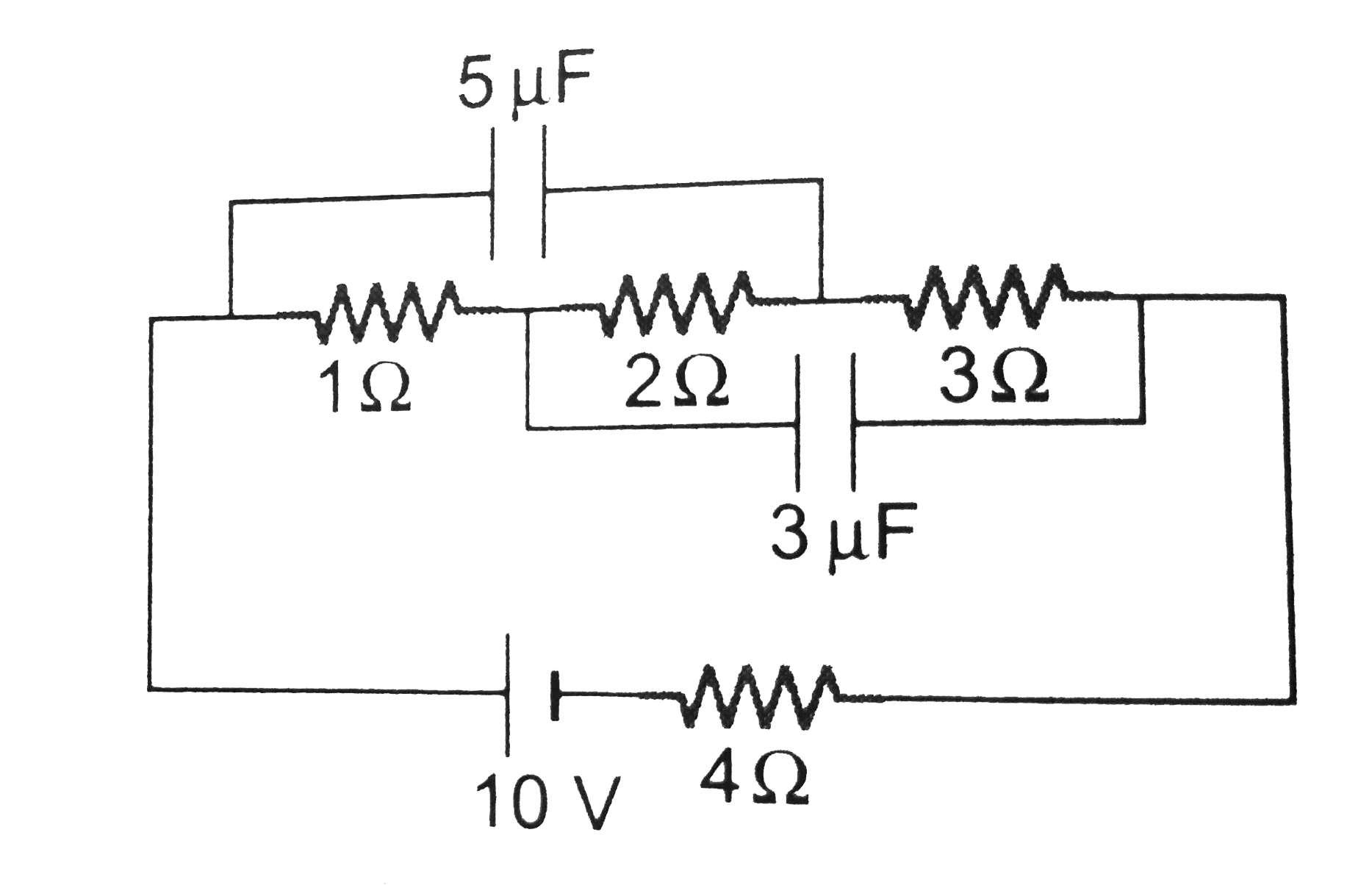
View SolutionAn infinite number of capacitors, having capacitances 1μF,2μF,4μFand8μF….. are connected in series. The equivalent capacitance of the system is
View SolutionFind the energy stored in a capacitor of capacitance 100μF when it is charged to a potential difference of 20 V.
View SolutionIn the above question, if the initial capacitance of the capacitor was 2μF, the amount of heat produced when the dielectric is inserted.
View SolutionA capacitor of capacitance C1=1μF can withstand maximum voltage V1=6kV and another capacitor of capacitance C2=3μF can withstand maximum voltage V24kV. When the two capacitors are connected in series, the combined system can withstand a maximum voltage of
View Solution