In figure , if AB||DC and AC, PQ intersect each other at the point O. Prove that OA.CQ=OC.AP.
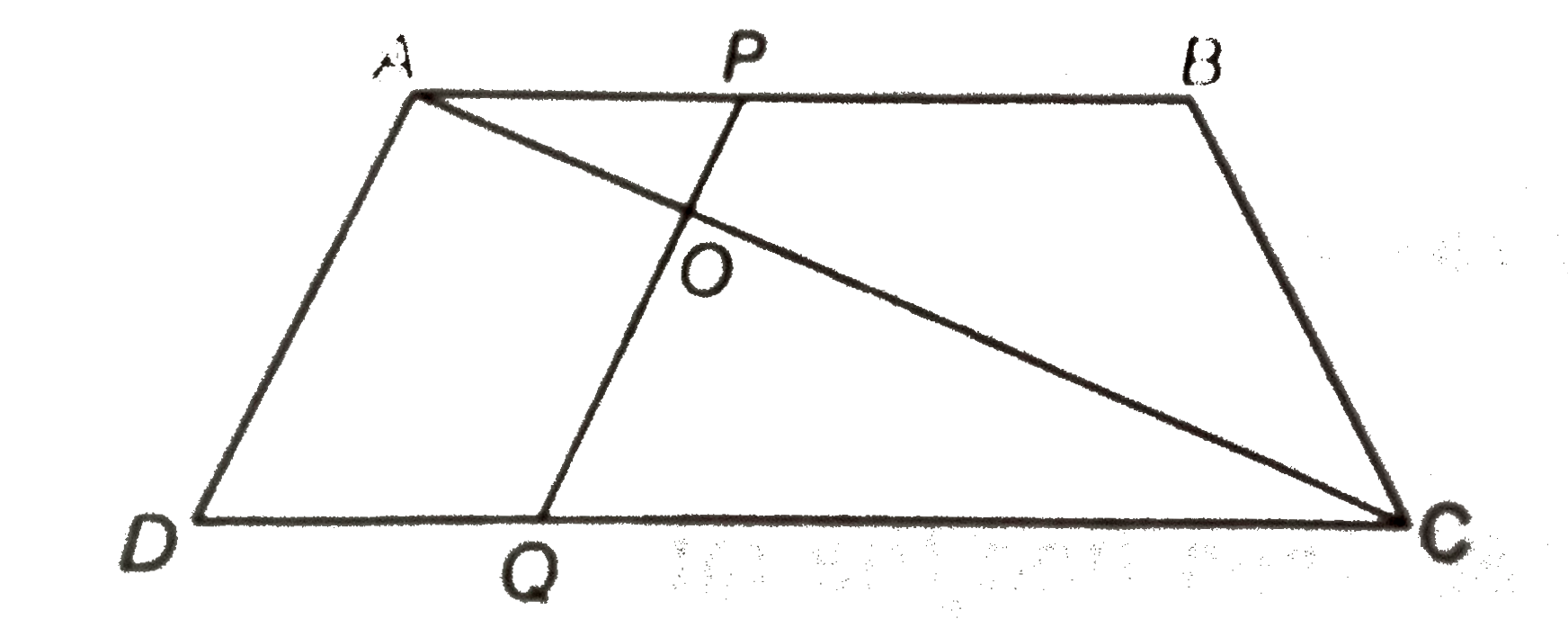
The correct Answer is:OA×CQ=OC×AP
Step by step video, text & image solution for In figure , if ABabs()DC and AC, PQ intersect each other at the point O. Prove that OA.CQ=OC.AP. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER -6 BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER -6 EXERCISE - Part - B (Section - V)
6 Videos
- SAMPLE PAPER -6 BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER -6 EXERCISE - Part - B (Section - III)
9 Videos
- SAMPLE PAPER -5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER -5EXERCISE - SECTION-C
11 Videos
- SAMPLE PAPER 01BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 01EXERCISE - PART-B (SECTION-V)
4 Videos
Similar Questions
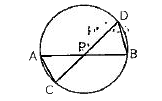
In the figure, two chords AB and CD intersect each other at the point P.
Prove that : AP. PB = CP. DP
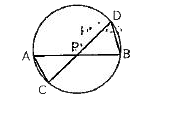
View SolutionIn the figure, two chords AB and CD intersect each other at the point P.
Prove that : ΔAPC~ΔDPB
View SolutionIn the figure the bisectors of ∠P and ∠Q intersect to each other at a point R. Prove that ∠PRQ=90∘.
View SolutionABCD is a trapezium in which AB||DC and its diagonals intersect each other as the point O. Show that AOBO=CODO.
View SolutionPQandRS are two parallel chords of a circle and lines RPandSQ intersect each other at O as shown in Figure. Prove that OP=OQ.

View SolutionPQ and RS are two parallel chords of a circle and lines RP and SQ intersect each other at O as shown in Figure. Prove that OP=OQ
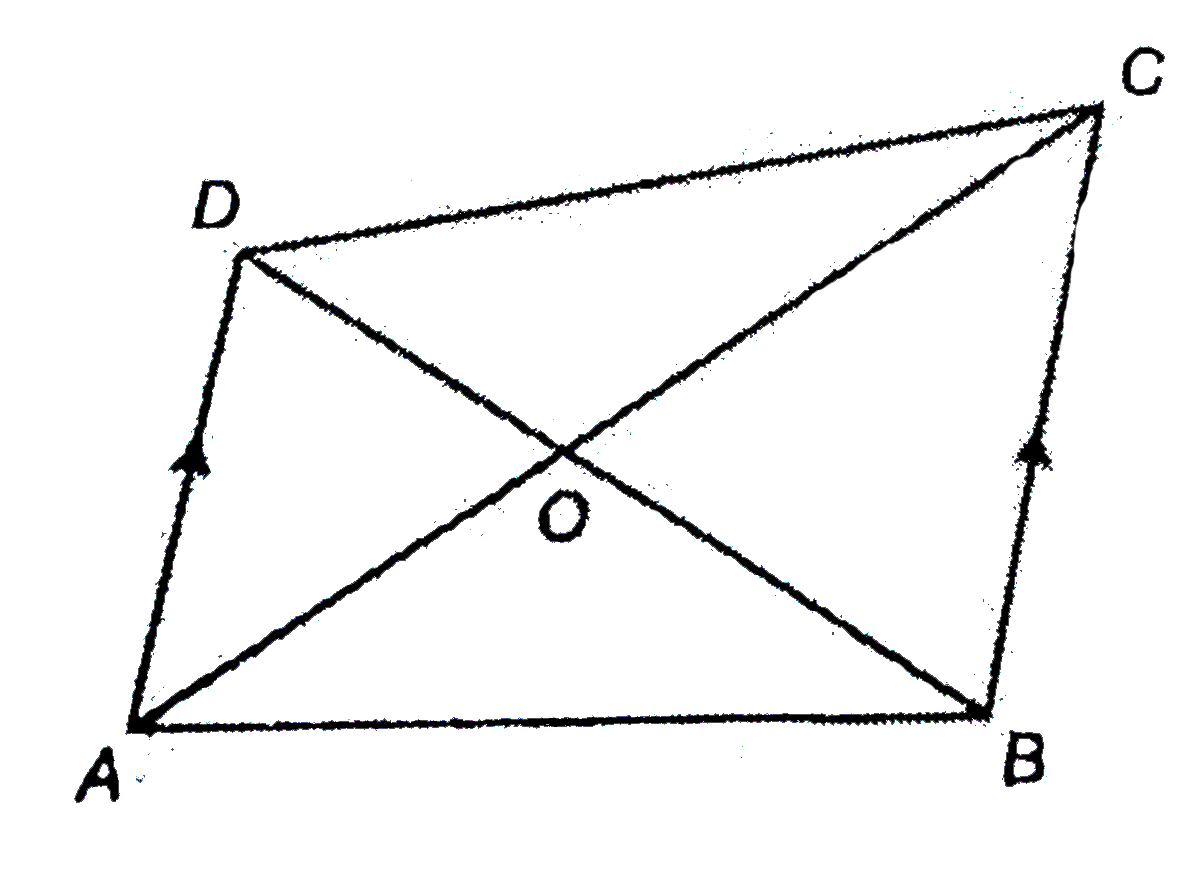
View SolutionIn the adjoining figure, ABCD is a quadrilateral in which AD∣∣BC. AC and BD intersect each other at point 'O'. Prove that:
area of ΔCOD= area of ΔABO
View SolutionIn the given figure AB and CD bisect each other at O. Prove that AC = BD.
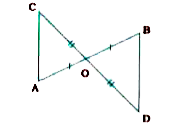
View SolutionTriangle ABC is an isosceles triangle with AB=AC. Bisectors of ∠Band∠C intersect each other at O. join A−O and prove that
OB=OC
View SolutionIn the given figure, chords PQ and RS intersect each other at point L. Find the lenth of RL.
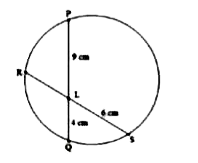
View Solution
