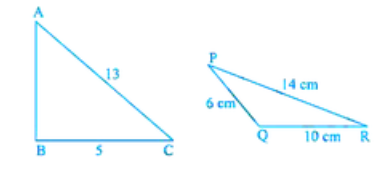
If then evaluate the length of AC. If perimeter of perimeter of cm and PR = 8 cm.
4 cm
6 cm
10 cm
3 cm
The correct Answer is:A
To find the length of AC in triangle ABC, given that triangle ABC is similar to triangle PQR, we can use the properties of similar triangles and the relationship between their perimeters.
1. Understand the Relationship of Similar Triangles:
Since triangle ABC is similar to triangle PQR, the ratios of corresponding sides are equal. This means:
ABPQ=ACPR=BCQR
2. Set Up the Ratio of Perimeters:
The ratio of the perimeters of the two triangles is equal to the ratio of their corresponding sides. Given:
- Perimeter of triangle ABC = 20 cm
- Perimeter of triangle PQR = 40 cm
Therefore, the ratio of the perimeters is:
Perimeter of ΔABCPerimeter of ΔPQR=2040=12
3. Use the Ratio to Find AC:
We know that PR corresponds to AC in the similar triangles. Given that PR = 8 cm, we can set up the equation:
ACPR=12
Substituting the value of PR:
AC8=12
4. Solve for AC:
To find AC, we can cross-multiply:
AC=8×12=4 cm
Final Answer:
The length of AC is 4 cm.
---
Since triangle ABC is similar to triangle PQR, the ratios of corresponding sides are equal. This means:
ABPQ=ACPR=BCQR
The ratio of the perimeters of the two triangles is equal to the ratio of their corresponding sides. Given:
- Perimeter of triangle ABC = 20 cm
- Perimeter of triangle PQR = 40 cm
Perimeter of ΔABCPerimeter of ΔPQR=2040=12
We know that PR corresponds to AC in the similar triangles. Given that PR = 8 cm, we can set up the equation:
ACPR=12
Substituting the value of PR:
AC8=12
To find AC, we can cross-multiply:
AC=8×12=4 cm
The length of AC is 4 cm.
Topper's Solved these Questions
- SAMPLE PAPER 02BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 02EXERCISE - SECTION-C
10 Videos
- SAMPLE PAPER 02BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 02EXERCISE - SECTION - A
11 Videos
- SAMPLE PAPER 02BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 02EXERCISE - SECTION - D
9 Videos
- SAMPLE PAPER 01BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 01EXERCISE - PART-B (SECTION-V)
4 Videos
- SAMPLE PAPER 03BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 03EXERCISE - Section - C
10 Videos
Similar Questions
Knowledge Check
If ΔABC ~ ΔPQR, then evaluate the length of AC, if perimeter of ΔABC = 20 cm, perimeter of ΔPQR = 40 cm and PR= 8 cm.
A4 cmB6 cmC10 cmD3 cmIf ΔABC≅ΔPQR then which of the following is not true?
ABC = PQBAC = PRCBC = QRDAB = PQΔXYZ is similar to ΔPQR.If ratio of perimeter of ΔXYZ and perimeter of ΔPQR is 16 : 9 and PQ = 3.6 cm, then what is the length (in cm) of XY?
A4.8B3.2C6.4D8.6
In the given triangles of Fig. 9.39, perimeter of ΔABC = perimeter of ΔPQR. Find the area of ΔABC.
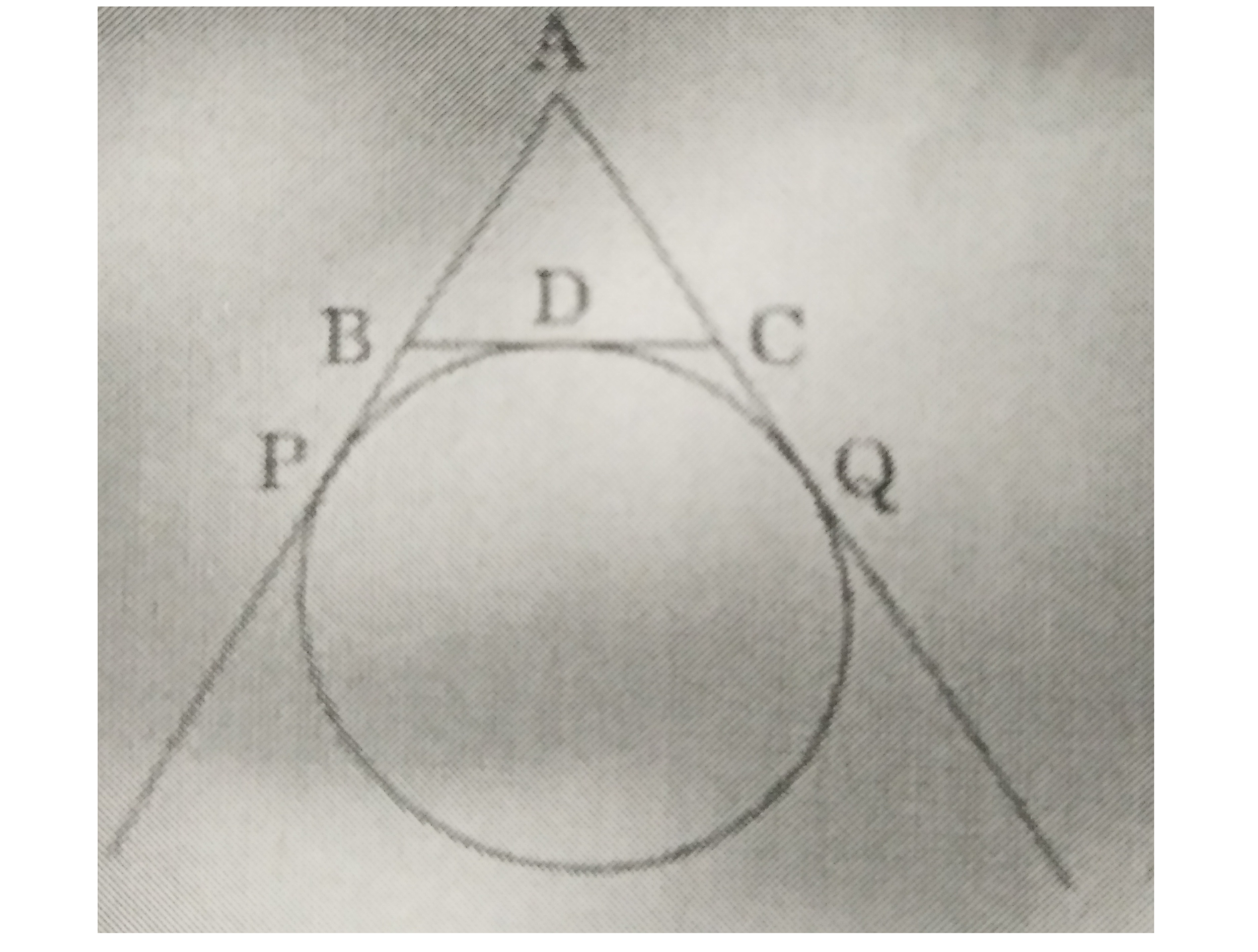
View SolutionIn Figure , find the perimeter of ΔABC. If AP = 12 cm.
View SolutionIf triangle ABC is similar to triangle PQR , Perimeter of triangle ABC = 30 cm , Perimeter of triangle = 45 cm , PR = 9 cm then find AC.
View SolutionΔABC~ΔDEF such that AB = 9.1 cm and DE =6.5 cm. If the perimeter of ΔDEF is 25 cm, what is the perimeter of ΔABC?
View SolutionΔXYZis similar to ΔPQR. If ratio of perimeter of ΔXYZand perimeter of ΔPQRis 16:9 and PQ=3.6cm, then what is the length (in cm) of XY?
View Solution