In space electric field is given by . A cube of side 0.5m is placed with one corner at origin along + y axis. Find charge enclosed inside cube:
The correct Answer is:D
To solve the problem, we need to find the charge enclosed inside the cube placed in an electric field defined by →E=150y2N/C. We will use Gauss's Law, which states that the electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space (ϵ0).
1. Understand the Geometry of the Cube:
The cube has a side length of 0.5m and is positioned such that one corner is at the origin (0, 0, 0) and extends along the positive y-axis. Therefore, the coordinates of the cube's corners will be:
- (0, 0, 0)
- (0.5, 0, 0)
- (0, 0.5, 0)
- (0, 0, 0.5)
- (0.5, 0.5, 0)
- (0.5, 0, 0.5)
- (0, 0.5, 0.5)
- (0.5, 0.5, 0.5)
2. Calculate the Electric Field at the Cube:
The electric field is given by →E=150y2N/C. Since the cube extends from y=0 to y=0.5, we need to evaluate the electric field at the average position of the cube along the y-axis. The average y value is:
yavg=0+0.52=0.25m
Now, substituting yavg into the electric field equation:
E=150(0.25)2=150×0.0625=9.375N/C
3. Calculate the Area of the Cube Face:
The area A of one face of the cube is given by:
A=side2=(0.5)2=0.25m2
4. Calculate the Electric Flux:
The electric flux ΦE through the face of the cube in the direction of the electric field is given by:
ΦE=E⋅A=9.375N/C×0.25m2=2.34375N m2/C
5. Use Gauss's Law to Find Charge Enclosed:
According to Gauss's Law:
ΦE=Qencϵ0
Rearranging gives:
Qenc=ΦE⋅ϵ0
Where ϵ0=8.85×10−12C2/N m2. Thus:
Qenc=2.34375N m2/C×8.85×10−12C2/N m2
Qenc≈2.08×10−11C
Final Answer:
The charge enclosed inside the cube is approximately 2.08×10−11C.
The cube has a side length of 0.5m and is positioned such that one corner is at the origin (0, 0, 0) and extends along the positive y-axis. Therefore, the coordinates of the cube's corners will be:
- (0, 0, 0)
- (0.5, 0, 0)
- (0, 0.5, 0)
- (0, 0, 0.5)
- (0.5, 0.5, 0)
- (0.5, 0, 0.5)
- (0, 0.5, 0.5)
- (0.5, 0.5, 0.5)
The electric field is given by →E=150y2N/C. Since the cube extends from y=0 to y=0.5, we need to evaluate the electric field at the average position of the cube along the y-axis. The average y value is:
yavg=0+0.52=0.25m
Now, substituting yavg into the electric field equation:
E=150(0.25)2=150×0.0625=9.375N/C
The area A of one face of the cube is given by:
A=side2=(0.5)2=0.25m2
The electric flux ΦE through the face of the cube in the direction of the electric field is given by:
ΦE=E⋅A=9.375N/C×0.25m2=2.34375N m2/C
According to Gauss's Law:
ΦE=Qencϵ0
Rearranging gives:
Qenc=ΦE⋅ϵ0
Where ϵ0=8.85×10−12C2/N m2. Thus:
Qenc=2.34375N m2/C×8.85×10−12C2/N m2
Qenc≈2.08×10−11C
The charge enclosed inside the cube is approximately 2.08×10−11C.
Similar Questions
A cube is placed inside an electric field, →E=150y2ˆj. The side of the cube is 0.5 m and is placed in the field as shown in the given figure. The charge inside the cube is :
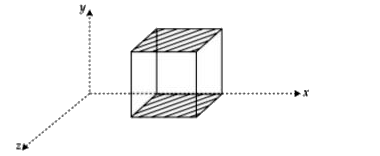
View SolutionA cube is placed inside an electric field, →E=150y2ˆj. The side of the cube is 0.5 m and is placed in the field as shown in the given figure. The charge inside the cube is :
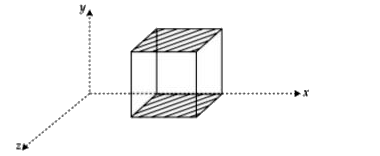
View Solution
Knowledge Check
Figure above shows a closed Gaussian surface in the space of a cube of edge length 3.0m. There exists an electric field given by →E=[(2.0x+4.0)i+8.0j+3.0k]N/C, where x is in metres, in the region in which it lies. The net charge in coulumbs enclosed by the cube is equal to
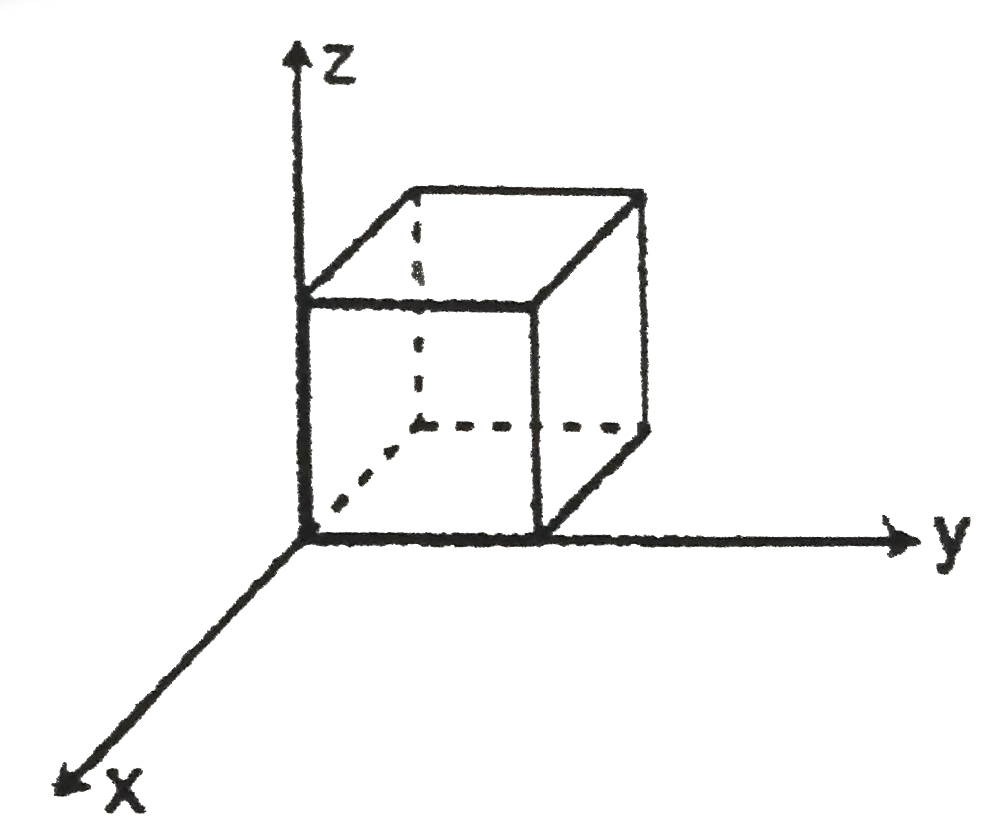 A−54∈0B6∈0C−6∈0D54∈0
A−54∈0B6∈0C−6∈0D54∈0An electric field given by →E=4ˆi+3(y2+2)ˆj passes through Gaussian cube of side 1m placed at origin such that its three sides represents x,y and z axis. This net charge enclosed within the cube is
A4ε0B3ε0C4ε0DzeroA uniform magnetic field existsin region given by →B=3ˆi+4ˆj+5ˆk. A rod of length 5m is placed along y-axis is moved along x- axis with constant speed 1m/sec. Then the magnitude of induced e.m.f in the rod is :
AzeroB25voltC20voltD15volt
An electric field given by →E=4ˆi+3(y2+2)ˆj pierces a gaussian cube of side 1m placed at origin such that one of its corners is at origin and rest of sides are along positive side of coordinate axis. Find the magnitude of net charge enclosed within the cube
[3∈0]
View SolutionAn electric field is given by →E=4ˆi+3(y2+2)ˆj pierces gaussian cube of side 1 m placed at origin such that one of its corners is at origin & rest of sides are along positive side of coordinate axis. If the magnitude of net charge enclosed is n ɛ0 then n (in SI units) will be equal to
View SolutionAn electric field given by →E=4ˆi−(20y2+2)ˆj pierces a Gussian cube of side 1 m placed at origin such that its three sides represents x,y and z axes. The net charge enclosed within the cube is x×10−10C, where is _____. (take ∈0=8.85×10−12N−1m−2C2)
View SolutionAn electric field given by ¯¯¯E=4ˆi−(3y2+2)ˆj pierces Gaussian's cube of side 1 m placed at origin such that its three sides represents x1y and z axis. The net charge enclosed within the cube is given by −neo. Find the value of n.
'(##CEN_KSR_PHY_JEE_C18_E01_024_Q11##)'
View SolutionElectric field in a region of space is radially outward from origin and varies with distance 'r' from origin as E = kr. Find the charge enclosed in a sphere of radius 'a' centred at origin.
View Solution