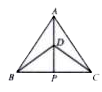
ΔABCandΔDBC have a common base and drawn towards one sides. ∠BAC=∠BDC=90∘. If AC and DB intersect at P, then:
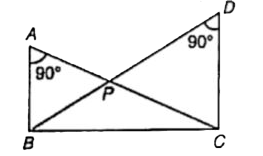
The correct Answer is:c2>a2+b2
Step by step video, text & image solution for DeltaABC and DeltaDBC have a common base and drawn towards one sides. angleBAC = angleBDC = 90^(@). If AC and DB intersect at P, then: by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
- BOOK - LUCENT PUBLICATIONCHAPTER - CONGRUENCE AND SIMILAR TRIANGLES EXERCISE - EXERCISE-5A47videos
CONGRUENCE AND SIMILAR TRIANGLES
- BOOK - LUCENT PUBLICATIONCHAPTER - CONGRUENCE AND SIMILAR TRIANGLES EXERCISE - EXERCISE-5B8videos
CONGRUENCE AND SIMILAR TRIANGLES
- BOOK - LUCENT PUBLICATIONCHAPTER - CIRCLE AND ITS TANGENT LINESEXERCISE - EXERCISE 8B27videos
CIRCLE AND ITS TANGENT LINES
- BOOK - LUCENT PUBLICATIONCHAPTER - ELEMENTARY TRIGONOMETRIC IDENTITIES EXERCISE - EXERCISE 11B37videos
ELEMENTARY TRIGONOMETRIC IDENTITIES
Similar Questions
Two triangles BACandBDC , right angled at AandD respectively, are drawn on the same base BC and on the same side of BC . If AC and DB intersect at P, prove that AP⋅PC=DP⋅PB.
View SolutionΔABCandΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to BP intersect BC at P, show that
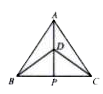
ΔABD≅ΔACDView Solution
Knowledge Check
- Question 1 - Select One
Two right triangles ABC and DBC are drawn on the same hypotenues BC on the same side of BC. If AC and DB intersects at P, then :
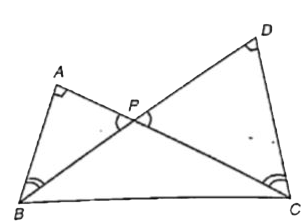 AAPPC=BPDPBAP×DP=PC×BPCAP×PC=BP×DPDAP×BP=PC×PD
AAPPC=BPDPBAP×DP=PC×BPCAP×PC=BP×DPDAP×BP=PC×PD - Question 2 - Select One
ΔABC and ΔDBC are on the same base BC but on opposite sides of it. AD and BC intersect each other at O. If AO=a cm, DO=b cm and the area of ΔABC=xcm2, then what is the area (in cm2) of ΔDBC?
AbxaBabxCab2xD(a+b)2x - Question 3 - Select One
In ΔABC,∠B=90∘. If P is the mid-point of side AC, then
PA=PB=AACB3ACC12ACD2AC
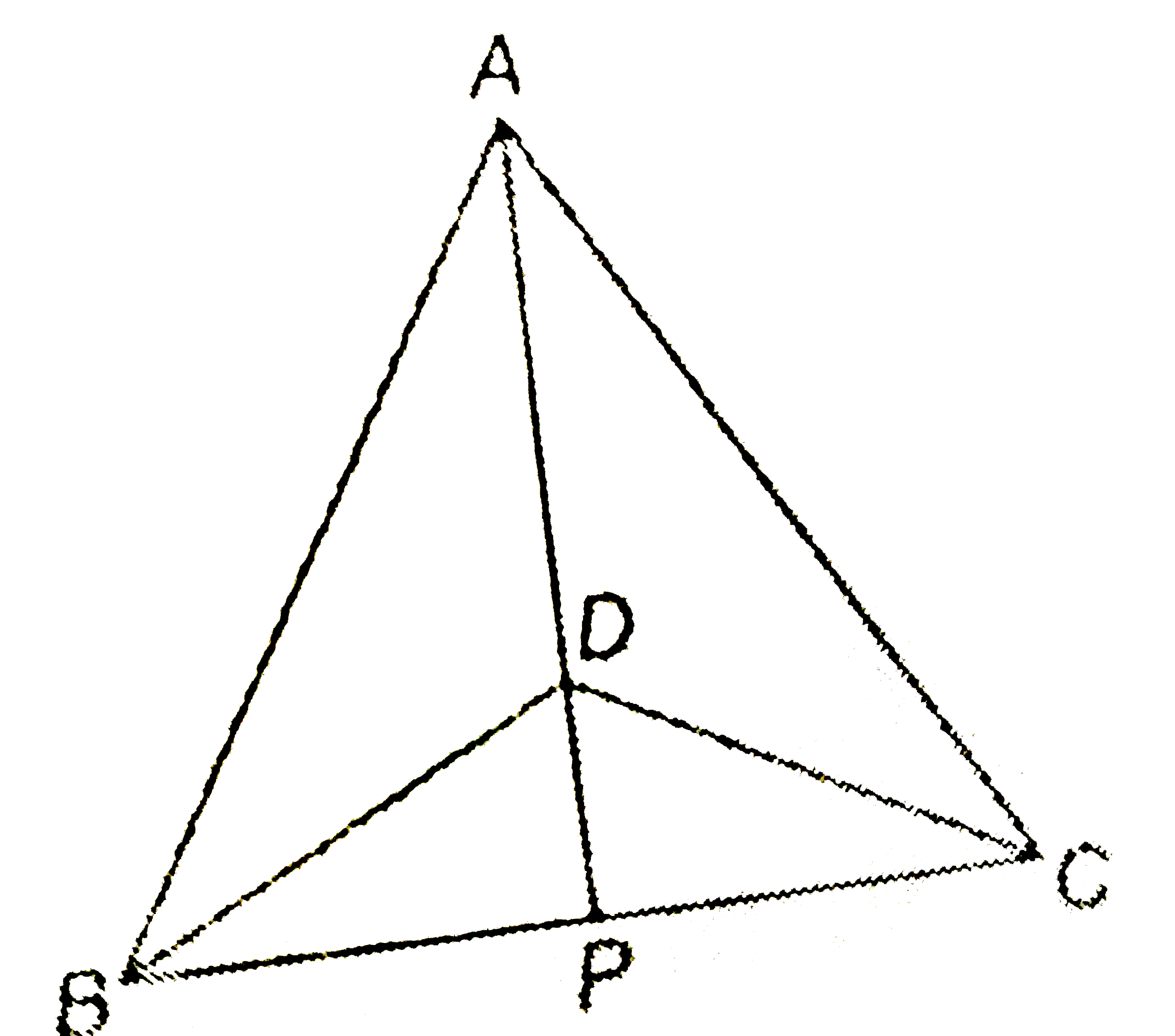
ΔABCandΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to BP intersect BC at P, show that
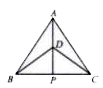
ΔABP≅ΔACPView SolutionΔABCandΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to BP intersect BC at P, show that
AP bisects ∠A as well as ∠D.View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to intersect BC at P, show that :
(i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
 View Solution
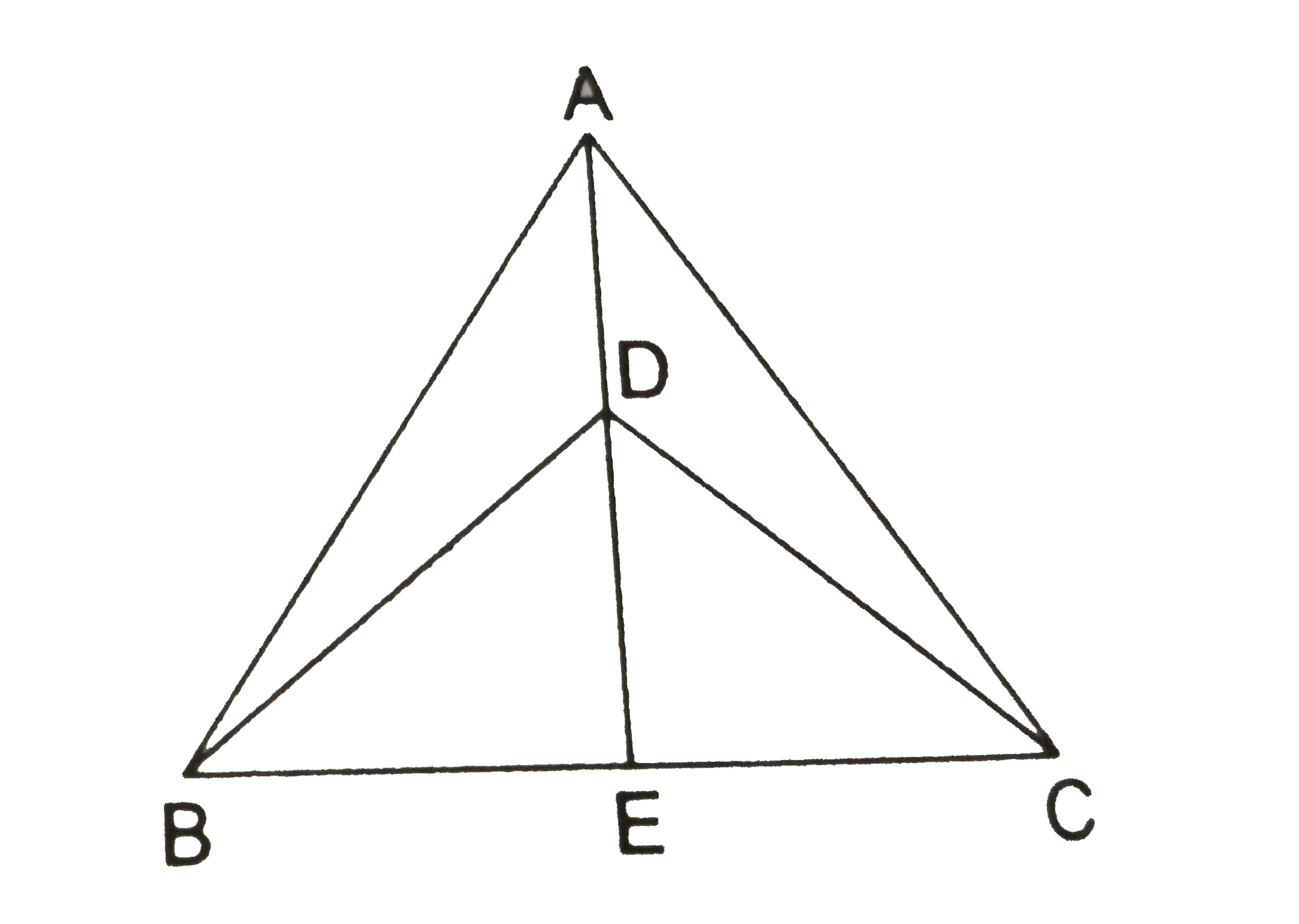
View SolutionΔABCandΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at E, show that
(i) ΔABD≅ΔACD
(ii) ΔABE≅ΔACE
(iii) AE bisects ∠A as well as ∠D
(iv) AE is the perpendicualr bisector of BC.
 View Solution
View SolutionSlove the following questions.
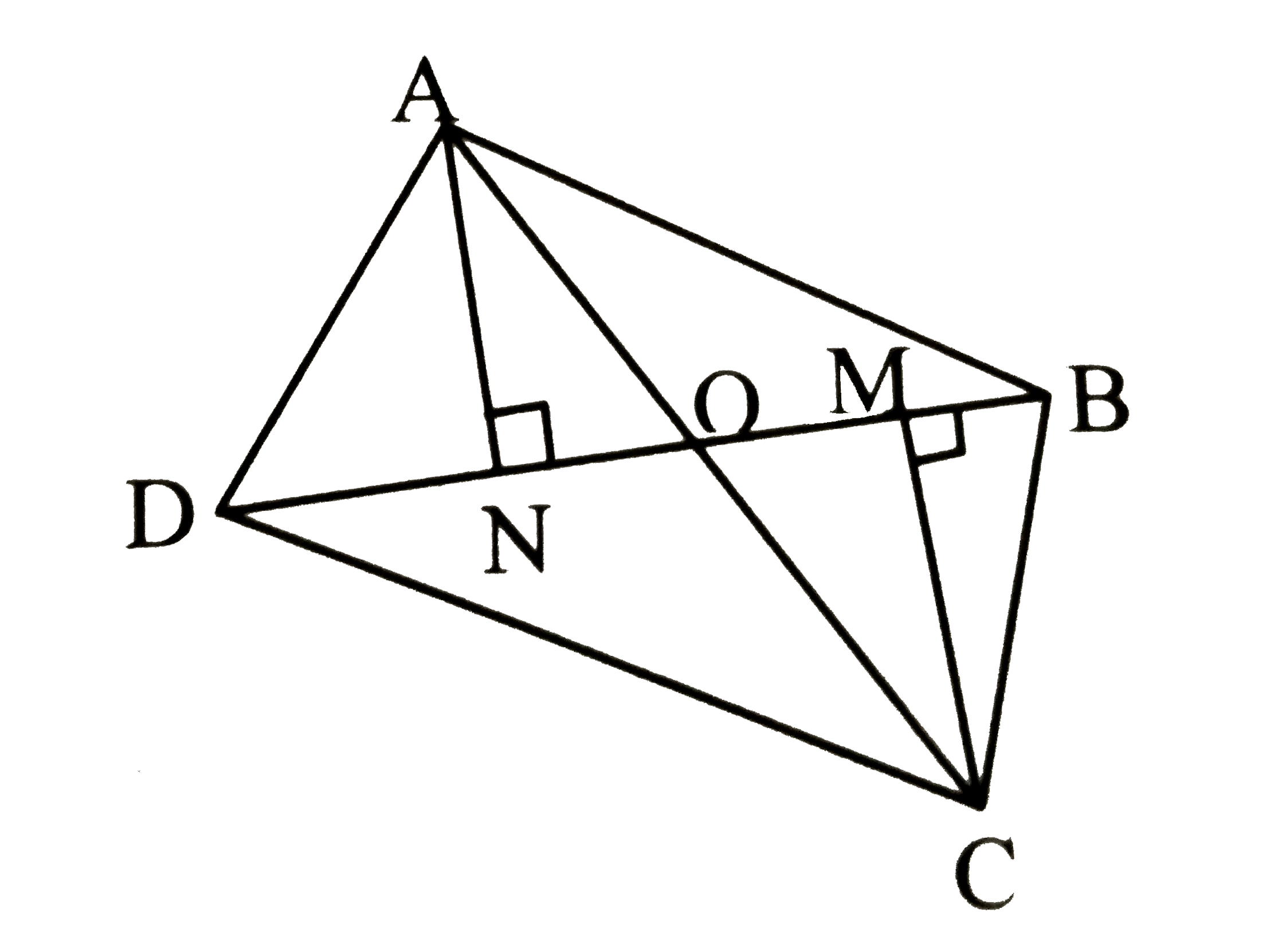
In the figure, ΔADBandΔCDB are drawn on the same base BD.if AC and BD intersect at O, then prove that A(ΔADB)A(ΔCDB)=AOCO.
 View Solution
View Solution
