A steel wire of cross-sectional area 4 cm2 has elastic limit of 2.2×108N/m2. The maximum upward acceleration that can be given to a 1000 kg elevator supported by this steel wire if the stress is to exceed one-fourth of the elastic limit is [Take, g = 10 m/s2 ]
10 m/s2
9m/s2
11m/s2
12m/s2
The correct Answer is:D
To solve the problem step by step, let's break it down:
Step 1: Understand the Given Data
- Cross-sectional area of the steel wire, A=4cm2=4×10−4m2
- Elastic limit of the steel wire, σmax=2.2×108N/m2
- Mass of the elevator, m=1000kg
- Acceleration due to gravity, g=10m/s2
Step 2: Calculate One-Fourth of the Elastic Limit
We need to find the maximum stress that can be applied without exceeding one-fourth of the elastic limit:
σallowed=14σmax=14×2.2×108=0.55×108N/m2
Step 3: Calculate the Maximum Tension in the Wire
Using the formula for stress, we can relate stress to tension and area:
σ=TA⟹T=σ×A
Substituting the values:
T=0.55×108×4×10−4=0.55×4×104=2.2×104N
Step 4: Apply Newton's Second Law
The tension in the wire must overcome the weight of the elevator and provide the necessary upward acceleration:
T−mg=ma
Where T is the tension, mg is the weight of the elevator, and a is the upward acceleration. Rearranging gives:
T=mg+ma
Factoring out m:
T=m(g+a)
Step 5: Substitute Known Values
Substituting T from Step 3:
2.2×104=1000(10+a)
Step 6: Solve for a
Now, we can solve for a:
2.2×104=10000+1000a
2.2×104−10000=1000a
1.2×104=1000a
a=1.2×1041000=12m/s2
Conclusion
The maximum upward acceleration that can be given to the elevator is:
12m/s2
- Cross-sectional area of the steel wire, A=4cm2=4×10−4m2
- Elastic limit of the steel wire, σmax=2.2×108N/m2
- Mass of the elevator, m=1000kg
- Acceleration due to gravity, g=10m/s2
We need to find the maximum stress that can be applied without exceeding one-fourth of the elastic limit:
σallowed=14σmax=14×2.2×108=0.55×108N/m2
Using the formula for stress, we can relate stress to tension and area:
σ=TA⟹T=σ×A
Substituting the values:
T=0.55×108×4×10−4=0.55×4×104=2.2×104N
The tension in the wire must overcome the weight of the elevator and provide the necessary upward acceleration:
T−mg=ma
Where T is the tension, mg is the weight of the elevator, and a is the upward acceleration. Rearranging gives:
T=mg+ma
Factoring out m:
T=m(g+a)
Substituting T from Step 3:
2.2×104=1000(10+a)
Now, we can solve for a:
2.2×104=10000+1000a
2.2×104−10000=1000a
1.2×104=1000a
a=1.2×1041000=12m/s2
The maximum upward acceleration that can be given to the elevator is:
12m/s2
Topper's Solved these Questions
Similar Questions
A steel wire with cross-section 3 cm2 has elastic limit 2.4 × 108 Nm−2. The maximum upward acceleration that can be given to a 1200 kg elevator supported by this cable if the stress is not to exceed one-third of the elastic limit (take g =10m/ s2) is
View SolutionThe elastic limit of a steel cable is 3.0×108N/m2 and the cross-section area is 4cm2. Find the maximum upward acceleration that can be given to a 900kg elevator supported by the cable if the stress is not to exceed one - third of the elastic limit.
View Solution
Knowledge Check
The elastic limit of an elevator cable is 2×109N/m2. The maximum upward acceleration that an elevator of amss 2×103kg can have been supported by a cable would not exceed half of the elastic limit would be
A10m/s2B50m/s2C40m/s2DNot possible to move upThe elastic limit of an elavator cable is 2×109N/m2. The maximum upward acceleration that an elavator of mass 2×103kg can have when supported by a cable whose cross sectional area is 10−4m2, provided the stres in cable would not exceed half to the elastic limit would be
A10ms−2B50ms−2C40ms−2DNot possible to move upIn the above problem if the the yield strength of steel is 2.5×108N/m2, then the maximum mass that can be hung at the lower end of the wire is
A785kgB78.75kgC78.25kgD78.50kg
A steel wire having cross-sectional area 2 mm^(2) is stretched by 20 N. Find the lateral strain produced in the wire Y_("steel") = 2 xx 10^(11) Nm^(-2), sigma = 0.391
View SolutionIf the elastic limit of copper is 1.5×108N/m2,determine the minimum diameter a copper wire can have under a load of10.0kgfind , if its elastic limit is not to be exceeded.
View SolutionThe maximum load that may be applied to a mild steel wire is 7 kg wt. If the radius of the wire is 0.2 mm, find the maximum elastic strain permitted . [Y=2×1011N/m2]
View SolutionA mild steel wire of length 2L and cross-sectional area A is stretched, well within elastic limit, horizontally between two pillars,
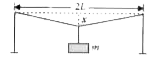
A mass m is suspended from the midpoint of the wire. Strain in the wire is
View SolutionA steal wire of cross-section area 3×10−6m2 can withstand a maximum strain of 10−3 .Young's modulus of steel is 2×1011Nm−2 .The maximum mass this wire can hold is
View Solution
