Question

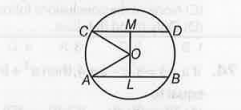
AB = 16 cm and CD = 12 cm are two parallel chords lie on same side of centre of a given circle. If distance between them is 2 cm then what is the radius of the circle?

Options

A. a)5 cm
B. b)10 cm
C. c)3 cm
D. d)12 cm

Answer

A

Explanation

To find the radius of the circle given the lengths of two parallel chords and the distance between them, we can follow these steps:

1. Identify the Given Information:
- Length of chord AB = 16 cm
- Length of chord CD = 12 cm
- Distance between the chords = 2 cm

2. Draw the Circle and Chords:
- Draw a circle with center O.
- Draw the two parallel chords AB and CD on the same side of the center O.

3. Determine the Midpoints of the Chords:
- Let P be the midpoint of chord AB. Since AB = 16 cm, then AP = PB = 8 cm.
- Let Q be the midpoint of chord CD. Since CD = 12 cm, then CQ = QD = 6 cm.

4. Set Up the Right Triangles:
- Draw perpendicular lines from the center O to the midpoints P and Q.
- The distance OP = x (the distance from the center to chord AB).
- The distance OQ = x + 2 (since the distance between the chords is 2 cm).

5. Apply the Pythagorean Theorem:
- For triangle OAP (right triangle):
\( OA^2 = OP^2 + AP^2 \implies r^2 = x^2 + 8^2 \implies r^2 = x^2 + 64 \)
- For triangle OCQ (right triangle):
\( OC^2 = OQ^2 + CQ^2 \implies r^2 = OQ^2 + 6^2 \implies r^2 = (x + 2)^2 + 36 \)

6. Set the Equations Equal:
- Since both expressions equal \( r^2 \):
\( x^2 + 64 = (x + 2)^2 + 36 \)

7. Expand and Simplify:
- Expand the right side:
\( (x + 2)^2 = x^2 + 4x + 4 \)
- Thus, the equation becomes:
\( x^2 + 64 = x^2 + 4x + 4 + 36 \)
- Simplifying gives:
\( x^2 + 64 = x^2 + 4x + 40 \)
- Cancel \( x^2 \) from both sides:
\( 64 = 4x + 40 \)

8. Solve for x:
- Rearranging gives:
\( 64 - 40 = 4x \implies 24 = 4x \implies x = 6 \)

9. Find the Radius:
- Substitute \( x = 6 \) back into the equation for \( r^2 \):
\( r^2 = x^2 + 64 = 6^2 + 64 = 36 + 64 = 100 \)
- Therefore, the radius \( r = \sqrt{100} = 10 \) cm.

Final Answer:
The radius of the circle is 10 cm.

---