Question

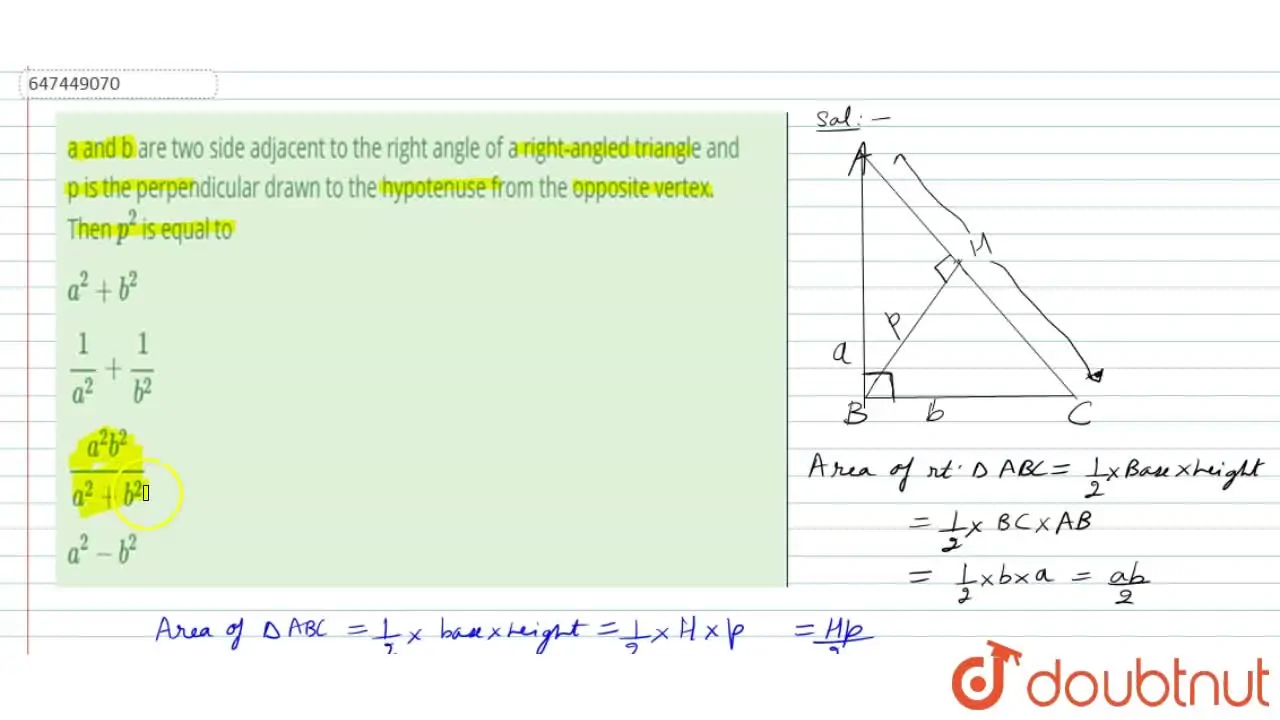
a and b are two side adjacent to the right angle of a right-angled triangle and p is the perpendicular drawn to the hypotenuse from the opposite vertex. Then ${\displaystyle p^2}$ is equal to

Options

A. `a^(2) + b^(2)`
B. `(1)/(a^(2)) + (1)/(b^(2))`
C. `(a^(2)b^(2))/(a^(2)+b^(2))`
D. `a^(2) - b^(2)`

Answer

C

Explanation

To solve the problem, we need to find the value of $$p^2$$ where $$p$$ is the perpendicular drawn from the opposite vertex to the hypotenuse of a right-angled triangle with sides $$a$$ and $$b$$.

1. Identify the Triangle and its Components:
- Let \( \triangle ABC \) be a right-angled triangle where \( \angle ABC = 90^\circ \).
- Let \( AB = c \) (the hypotenuse), \( BC = a \), and \( AC = b \).
- The point \( P \) is the foot of the perpendicular drawn from vertex \( A \) to the hypotenuse \( BC \).

2. Area of Triangle Using Base and Height:
- The area of triangle \( ABC \) can be calculated using the two sides adjacent to the right angle:
\( \text{Area} = \frac{1}{2} \times a \times b \)

3. Area of Triangle Using Hypotenuse and Height:
- The area can also be expressed using the hypotenuse \( c \) and the height \( p \):
\( \text{Area} = \frac{1}{2} \times c \times p \)

4. Equating the Two Area Expressions:
- Since both expressions represent the same area, we can set them equal to each other:
\( \frac{1}{2} \times a \times b = \frac{1}{2} \times c \times p \)
- Simplifying this gives:
\( ab = cp \quad \text{(Equation 1)} \)

5. Using Pythagorean Theorem:
- According to the Pythagorean theorem:
\( c^2 = a^2 + b^2 \)
- Therefore, we can express \( c \) as:
\( c = \sqrt{a^2 + b^2} \)

6. Substituting \( c \) into Equation 1:
- Substitute \( c \) into Equation 1:
\( ab = \sqrt{a^2 + b^2} \cdot p \)

7. Solving for \( p \):
- Rearranging gives:
\( p = \frac{ab}{\sqrt{a^2 + b^2}} \)

8. Finding \( p^2 \):
- Now, squaring both sides to find \( p^2 \):
\( p^2 = \left(\frac{ab}{\sqrt{a^2 + b^2}}\right)^2 \)
- This simplifies to:
\( p^2 = \frac{a^2b^2}{a^2 + b^2} \)

Conclusion:
Thus, the value of \( p^2 \) is:
\( p^2 = \frac{a^2b^2}{a^2 + b^2} \)