From two points on the ground lying on a straight line through the foot of a pillaer , the two angles of elevation of the top of the pillar are complementary to each other . If the distance of the two points from the foot of the pillar are 9 metres and 16 metres and the two points lie on the same side of the pillar , then the height of the pillar is
5 m
10 m
7 m
12 m
The correct Answer is:D
To find the height of the pillar given the distances from two points and the fact that the angles of elevation are complementary, we can follow these steps:
1. Understand the Problem:
We have a pillar with height h. There are two points, P and Q, on the ground from which the angles of elevation to the top of the pillar are complementary. The distances from the foot of the pillar to points P and Q are 9 meters and 16 meters, respectively.
2. Define Angles:
Let the angle of elevation from point P be θ. Therefore, the angle of elevation from point Q will be 90∘−θ since they are complementary.
3. Set Up the Tangent Ratios:
From point P:
tan(θ)=h9(1)
From point Q:
tan(90∘−θ)=cot(θ)=h16(2)
4. Relate Tangent and Cotangent:
Recall that cot(θ)=1tan(θ). Therefore, from equation (1):
cot(θ)=9h
Substituting this into equation (2):
h16=9h
5. Cross-Multiply:
Cross-multiplying gives:
h2=9×16
6. Calculate h2:
h2=144
7. Find h:
Taking the square root of both sides:
h=√144=12 meters
Conclusion:
The height of the pillar is 12 meters.
We have a pillar with height h. There are two points, P and Q, on the ground from which the angles of elevation to the top of the pillar are complementary. The distances from the foot of the pillar to points P and Q are 9 meters and 16 meters, respectively.
Let the angle of elevation from point P be θ. Therefore, the angle of elevation from point Q will be 90∘−θ since they are complementary.
From point P:
tan(θ)=h9(1)
From point Q:
tan(90∘−θ)=cot(θ)=h16(2)
Recall that cot(θ)=1tan(θ). Therefore, from equation (1):
cot(θ)=9h
Substituting this into equation (2):
h16=9h
Cross-multiplying gives:
h2=9×16
h2=144
Taking the square root of both sides:
h=√144=12 meters
The height of the pillar is 12 meters.
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE -IV22videos
TRIGONOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE -V45videos
TRIGONOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE - II420videos
TRIGONOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - TIME AND WORKEXERCISE - TEST YOURSELF25videos
TIME AND WORK
Similar Questions
From a point on the ground 40 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘. The angle of elevation of the top of a water tank(on the top of tower) is 45∘.find the height of the tower and depth of tank
View SolutionFrom a point on the ground which is at a distance of 50 m from the foot of the towe, the angle of elevation of the top of the tower is observed to be 30∘. Find the height of the tower.
View Solution
Knowledge Check
- Question 1 - Select One
From two points on the ground and lying on a straight line through the foot of a pillar, the two angles of elevation of the top of the pillar are complementary to each other. If the distances of the two points from the foot of the pillar are 12 metres and 27 metres and the two points lie on the same side of the pillar, then the height (in metres) of the pillar is:
A16B12C15D18 - Question 2 - Select One
A and B are two points in the horizontal plane through O,, the foot of a pillar OP of height h such that ∠AOB=θ. If the elevation of the top of the pillar from A and B are also equal to θ, then AB is equal to
AhcotθBhcosθsecθ2Chcotθ sinθ2Dhcosθcosecθ2 - Question 3 - Select One
From two points , lying on the same horizontal line , the angles of elevation of the top of the pilar are θandϕ(θ<ϕ) . If the height of the pillar is h m and the two points lie on the same sides of the pillar , then the the distance between the two points is
Ah(tanθ−tanϕ) metreBh(cotϕ−cotθ) metreCh(cotθ−cotϕ) metreDh(tanθtanϕtanϕ−tanθ) metre
From a point on the ground, 20m away from the foot of a vertical tower, the angle of elevation of the top of the tower is 60o , what is the length of the tower?
View SolutionIn figure , the angle of elevation of the top of a tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m, Find the distance of the point from the foot of the power .
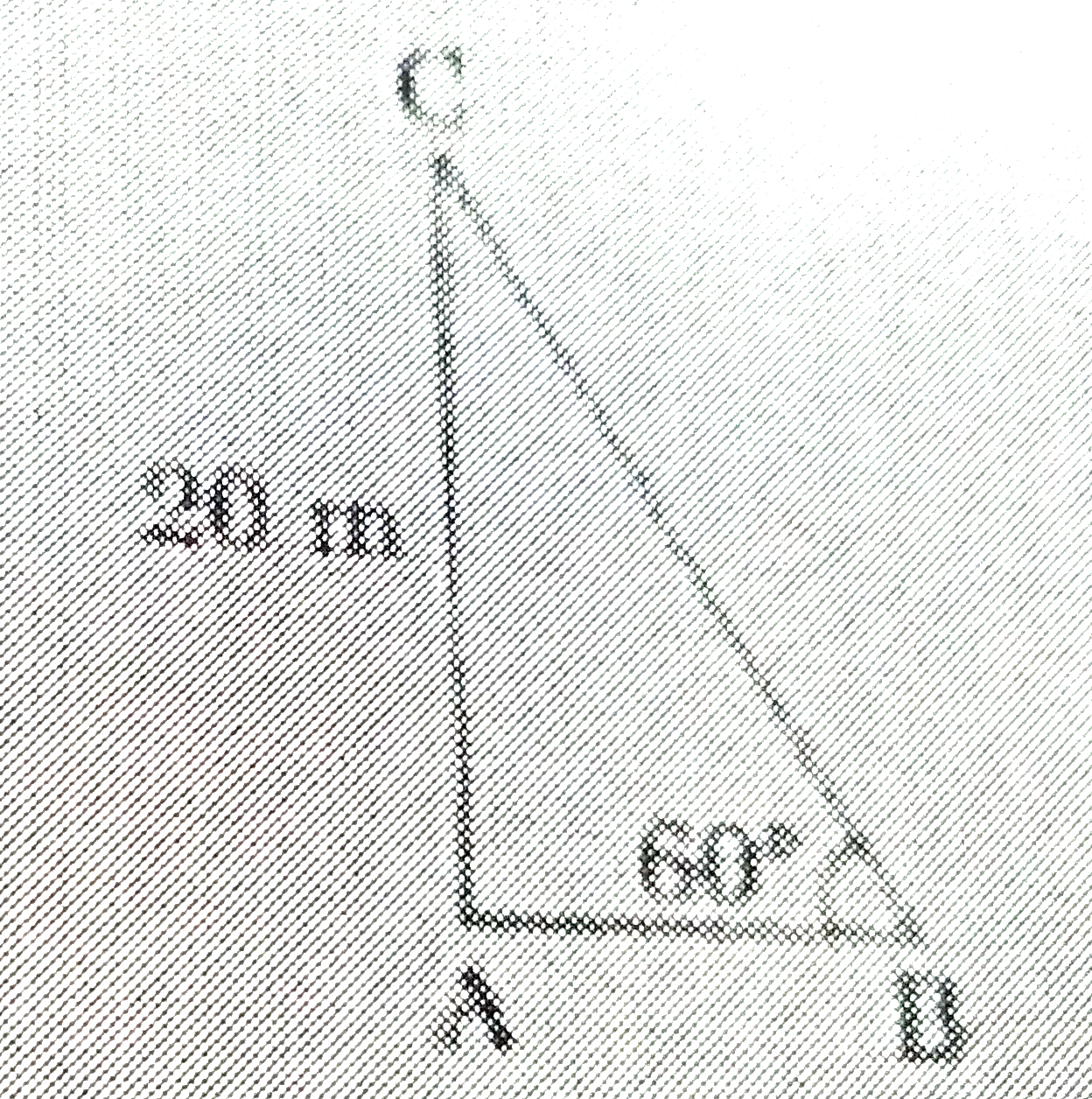 View Solution
View SolutionA and B are two points in the horizontal plane through O, the foot of pillar OP of height h such that ∠AOB=θ. If the elevation of the top of the pillar from A and B are also equal to θ, then AB is equal to
View SolutionA lower of height 15 m stands vertically on the ground. From a point on the ground the angle of elevation of the top of the tower is found to be 30∘. What is the distance of the point from the foot of the tower?
View SolutionA tower of height 15 m stands vertically on the ground. From a point on the ground the angle of elevation of the top of the tower is found to be 30∘. What is the distance of the point from the foot of the tower?
View Solution
