From a point P on a level ground the angle of elevation to the top of the tower is 30∘ .If the tower is 100 metre high , the distance of point P from the foot of the tower is (Take √3=1.73)
149 metre
156 metre
173 metre
188 metre
The correct Answer is:C
To solve the problem, we will use trigonometric ratios. We are given the height of the tower and the angle of elevation from point P to the top of the tower.
1. Identify the Given Information:
- Height of the tower (AB) = 100 meters
- Angle of elevation (∠APB) = 30 degrees
2. Draw a Right Triangle:
- Let P be the point on the ground.
- Let A be the foot of the tower (the base).
- Let B be the top of the tower.
- The triangle formed is triangle APB, where:
- AB is the height of the tower (perpendicular).
- AP is the distance from point P to the foot of the tower (base).
- PB is the line of sight from point P to the top of the tower.
3. Use the Tangent Function:
- In triangle APB, we can use the tangent of the angle of elevation:
tan(θ)=OppositeAdjacent
- Here, θ=30∘, the opposite side is AB (height of the tower = 100 m), and the adjacent side is AP (the distance we want to find).
- Therefore:
tan(30∘)=ABAP
- We know that tan(30∘)=1√3.
4. Set Up the Equation:
- Plugging in the values:
1√3=100AP
5. Cross-Multiply to Solve for AP:
- Cross-multiplying gives:
AP=100⋅√3
6. Substitute the Value of √3:
- Given that √3≈1.73:
AP=100⋅1.73=173 meters
7. Conclusion:
- The distance of point P from the foot of the tower is 173 meters.
- Height of the tower (AB) = 100 meters
- Angle of elevation (∠APB) = 30 degrees
- Let P be the point on the ground.
- Let A be the foot of the tower (the base).
- Let B be the top of the tower.
- The triangle formed is triangle APB, where:
- AB is the height of the tower (perpendicular).
- AP is the distance from point P to the foot of the tower (base).
- PB is the line of sight from point P to the top of the tower.
- In triangle APB, we can use the tangent of the angle of elevation:
tan(θ)=OppositeAdjacent
- Here, θ=30∘, the opposite side is AB (height of the tower = 100 m), and the adjacent side is AP (the distance we want to find).
- Therefore:
tan(30∘)=ABAP
- We know that tan(30∘)=1√3.
- Plugging in the values:
1√3=100AP
- Cross-multiplying gives:
AP=100⋅√3
- Given that √3≈1.73:
AP=100⋅1.73=173 meters
- The distance of point P from the foot of the tower is 173 meters.
Topper's Solved these Questions
- TRIGONOMETRY BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE -IV
22 Videos
- TRIGONOMETRY BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE -V
45 Videos
- TRIGONOMETRY BOOK - KIRAN PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - TYPE - II
420 Videos
- TIME AND WORKBOOK - KIRAN PUBLICATIONCHAPTER - TIME AND WORKEXERCISE - TEST YOURSELF
25 Videos
Similar Questions
From a point P on a level ground, the angle of elevation of the top of a tower is 30o . If the tower is 100 m high, the distance of point P from the foot of the tower is (a) 149 m (b) 156 m (c) 173 m (d) 200 m
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
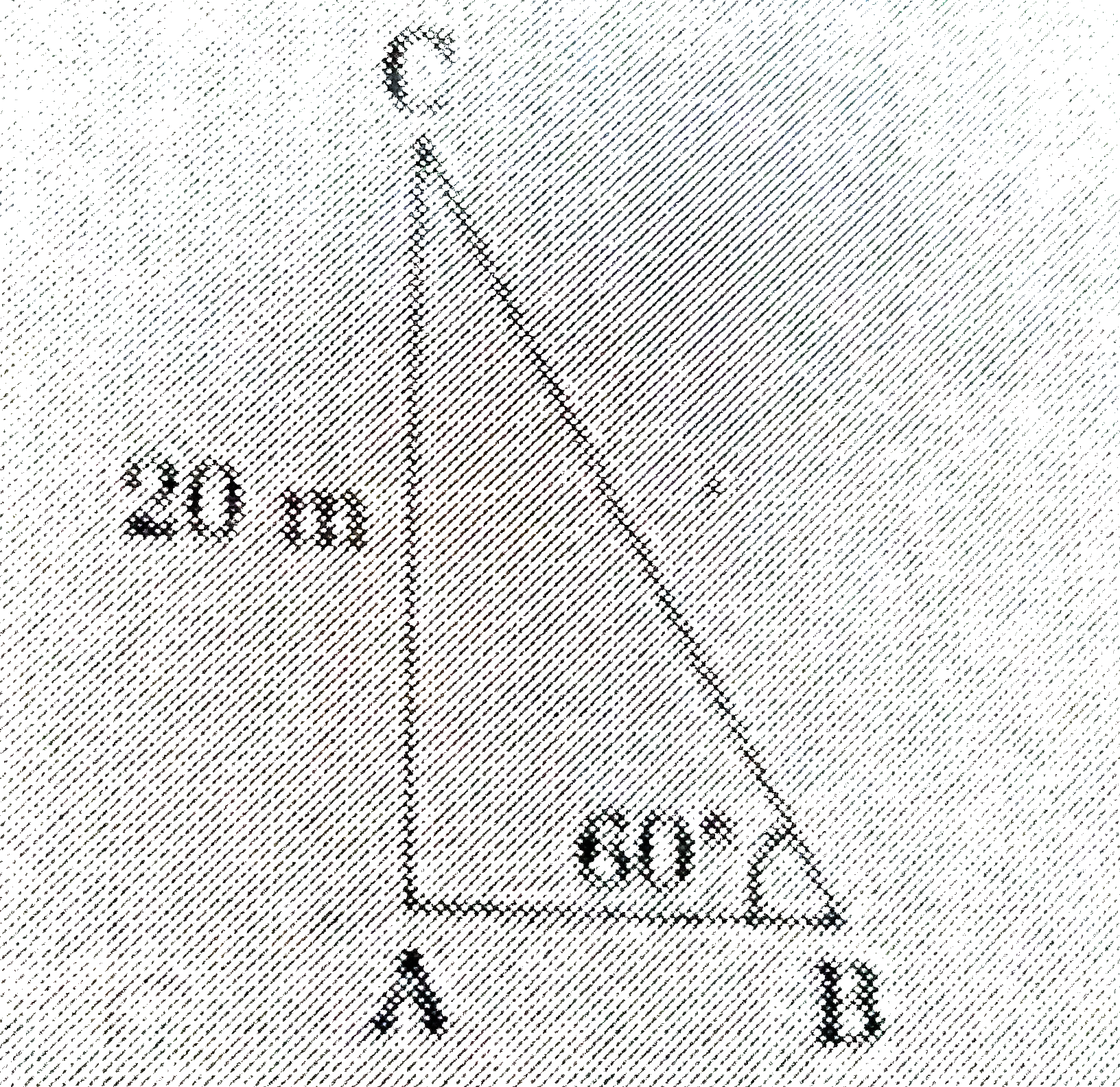
View Solution
Knowledge Check
From a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ . The height of the tower is
A10√3mB20√3 mC10√3 mD20√3mAt a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ The height of the tower is
A20√3mB20√3mC√320mDNone of theseAt a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ The height of the tower is
A20√3mB20√3mC√320mDNone of these
In figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionFrom a point on the ground 40 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘. The angle of elevation of the top of a water tank(on the top of tower) is 45∘.find the height of the tower and depth of tank
View SolutionTwo men are on opposite sides of a tower .They measure the angles of elevation of the top of the tower as 30∘and45∘ respectively .If the height of the tower is 50 metre , the distance between the two men is (Take √3=1.73)
View SolutionA tower of height 15 m stands vertically on the ground. From a point on the ground the angle of elevation of the top of the tower is found to be 30∘. What is the distance of the point from the foot of the tower?
View SolutionA lower of height 15 m stands vertically on the ground. From a point on the ground the angle of elevation of the top of the tower is found to be 30∘. What is the distance of the point from the foot of the tower?
View Solution
