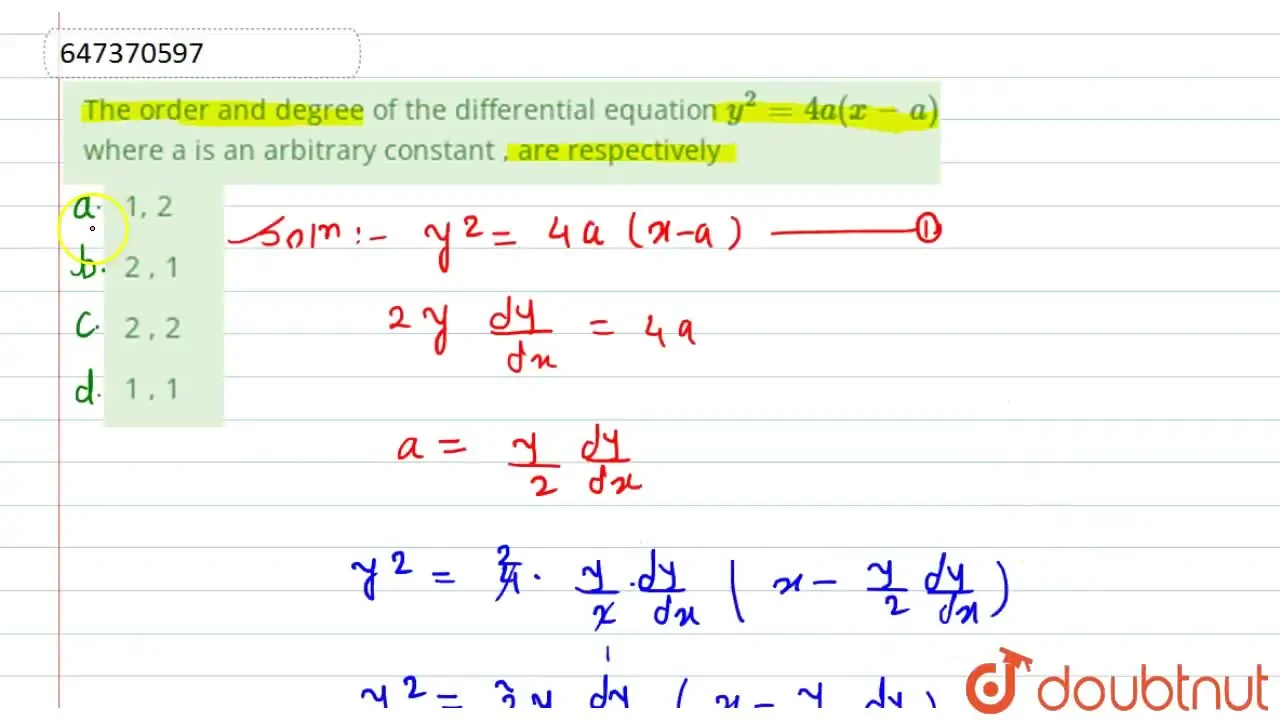
The order and degree of the differential equation y2=4a(x−a) , where a is an arbitrary constant , are respectively
1, 2
2 , 1
2 , 2
1 , 1
The correct Answer is:A
Step by step video, text & image solution for The order and degree of the differential equation y^(2) = 4a (x -a) , where a is an arbitrary constant , are respectively by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
The order and degrree of the differential equation y2=4a(x−a) , where 'a' is an arbitrary constant,are respectively .
View SolutionThe degree of the differential equaiton which has a solution y2=4a(x+a2) where a is arbitrary constant
View SolutionDifferential equation yy1+x=A, where A is an arbitrary constant, represents a family of
View SolutionThe solution of the differential equation dydx=2x−yx−6y is (where c is an arbitrary constant)
View SolutionThe solution of the differential equation dydx=x−yx−3y is (where, c is an arbitrary constant)
View SolutionThe solution of the differential equation dy−ydx2x=√xydy is (where , c is an arbitrary constant)
View SolutionFind the differential equation for the family of curves y2=4a(x+b)where c is an arbitrary constant.
View SolutionThe differential equation for the family of curves x2+y2−2ay=0, where a is an arbitrary constant is
View SolutionThe solution of differential equation x2(xdy+ydx)=(xy−1)2dx is (where c is an arbitrary constant)
View SolutionThe solution of the differential equation xdydx=yln(y2x2) is (where, c is an arbitrary constant)
View Solution