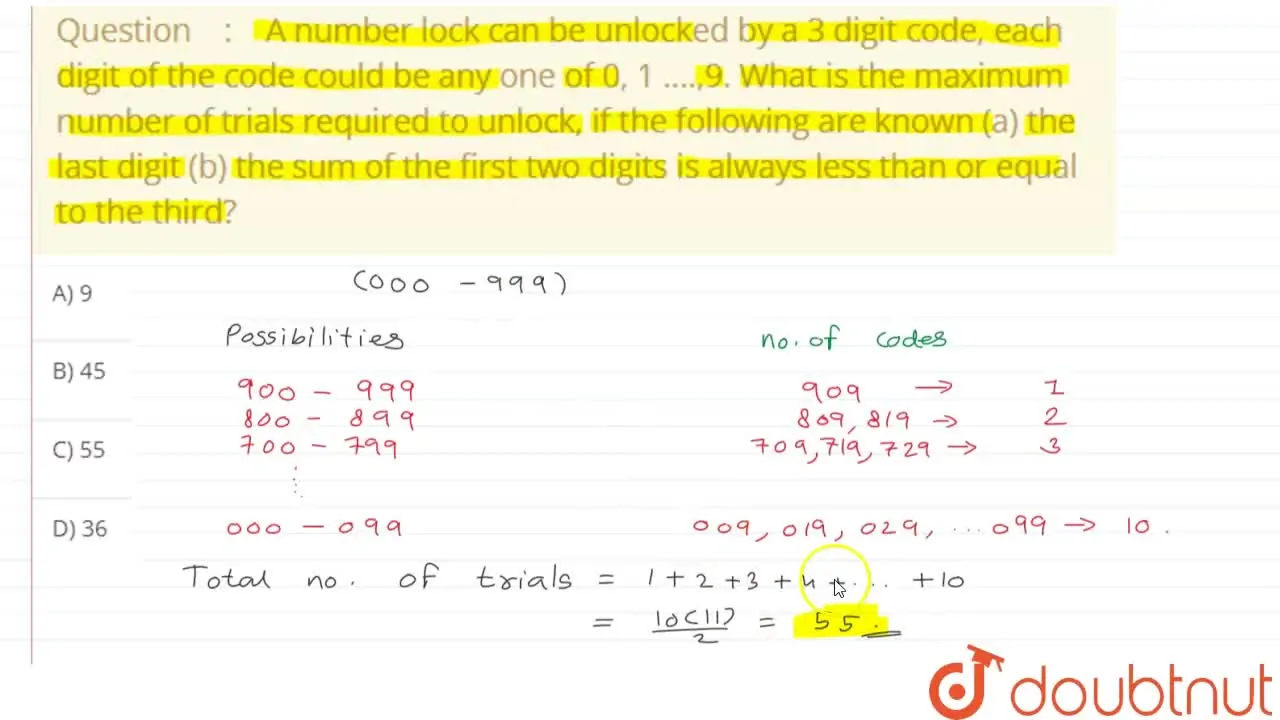
A number lock can be unlocked by a 3 digit code, each digit of the code could be any one of 0, 1 ....,9. What is the maximum number of trials required to unlock, if the following are known (a) the last digit (b) the sum of the first two digits is always less than or equal to the third?
A) 9
B) 45
C) 55
D) 36
The correct Answer is:C
Step by step video, text & image solution for A number lock can be unlocked by a 3 digit code, each digit of the code could be any one of 0, 1 ....,9. What is the maximum number of trials required to unlock, if the following are known (a) the last digit (b) the sum of the first two digits is always less than or equal to the third? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
What are the last two digits of the number 9200
View SolutionWhat are the last two digit of the number 9200 ?
View SolutionWhat are the last two digits of the number 9200 ?
View SolutionThe number of three digit numbers such that its first two digits are each divisible by its third digit is
View SolutionIn a two-digit number, the units digit is 3 more than the ten's digit. The sum of the digits is 18 less than the original number. Find the product of the digits
View SolutionIn a two-digit number, the units digit is 3 more than the ten's digit. The sum of the digits is 18 less than the original number. Find the product of the digits
View SolutionA three digit number is equal to 17 times the sum of its digits. If 198 is added to the number, the digits get reserved. The sum of the extreme digits is 1 less than the midle digit. Find the number.
View SolutionWhat is the two digit number ?
(i) The difference between the two digits is 9.
(ii) The sum of the digits is equal to the difference between the two digits.View SolutionA number is formed with two digits. The tens digit is more than the unit digit and the sum of digits is 17 of the numbers. Find the number.
View SolutionThe units digit of a two digit number is 2 more than the tens digit. If the number is subtracted from the sum of the squares of its digits the result is two thirds of the product of the digits. What is the number?
View Solution