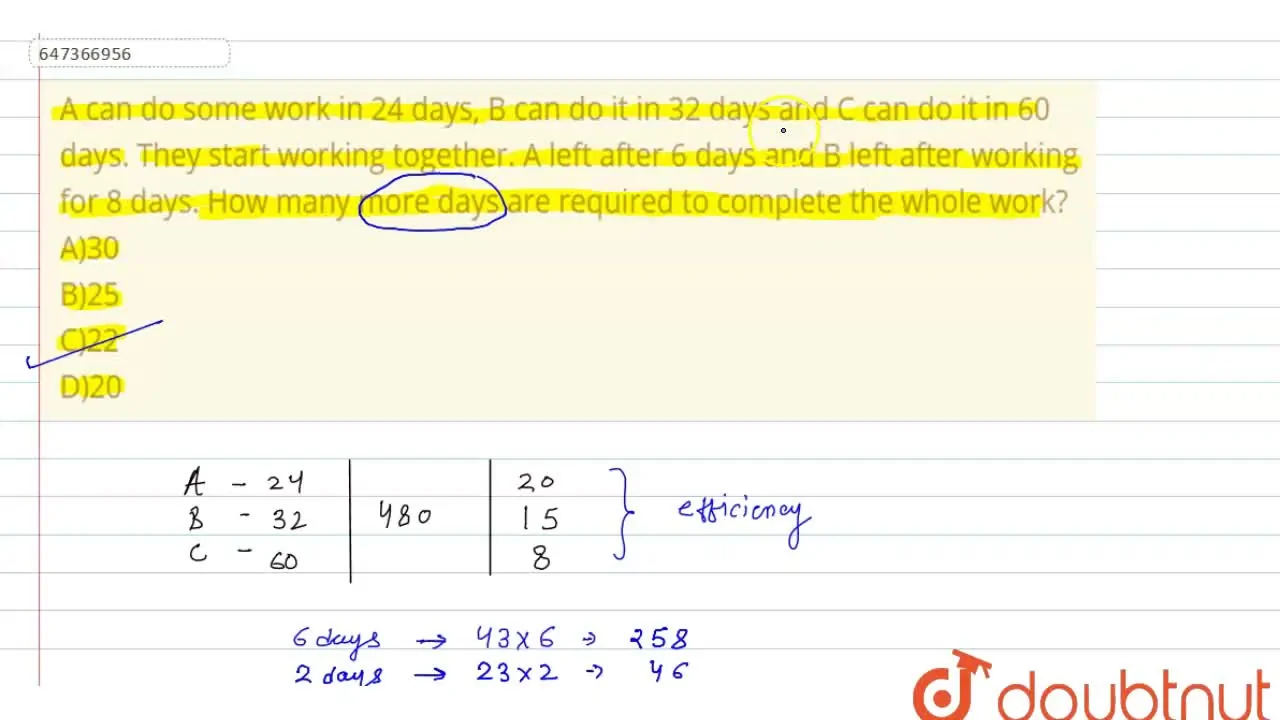
A can do some work in 24 days, B can do it in 32 days and C can do it in 60 days. They start working together. A left after 6 days and B left after working for 8 days. How many more days are required to complete the whole work?
A)30
B)25
C)22
D)20
30
25
22
20
The correct Answer is:C
Step by step video, text & image solution for A can do some work in 24 days, B can do it in 32 days and C can do it in 60 days. They start working together. A left after 6 days and B left after working for 8 days. How many more days are required to complete the whole work? A)30 B)25 C)22 D)20 by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - DISHA PUBLICATIONCHAPTER - TIME AND WORKEXERCISE - Practice Exercise (Standard Level)37videos
TIME AND WORK
- BOOK - DISHA PUBLICATIONCHAPTER - TIME AND WORKEXERCISE - Practice Exercise (Expert Level)27videos
TIME AND WORK
- BOOK - DISHA PUBLICATIONCHAPTER - TIME AND WORKEXERCISE - Test Yourself15videos
TIME AND WORK
- BOOK - DISHA PUBLICATIONCHAPTER - SET THEORYEXERCISE - Test Yourself15videos
SET THEORY
- BOOK - DISHA PUBLICATIONCHAPTER - TIME, SPEED AND DISTANCEEXERCISE - Test Yourself15videos
TIME, SPEED AND DISTANCE
Similar Questions
A can do a work in 20 days and B can do it in 14 days. If they work together for 7 days, then the fraction of the work left is:
View SolutionA can do a piece of work in 30 days while B can do it in 40 days. In how many days can A and B working together do it?
View SolutionA can do 40% of a work in 6 days and B can do 30% of the same work in 3 days. They started the work together but B left after 2 days and A continued to work. In how many days was the entire work completed ?
A किसी कार्य का 40% भाग 6 दिनों में तथा B इसी कार्य का 30% भाग 3 दिनों में पूरा कर सकता है | उन्होंने एक साथ कार्य शुरू किया लेकिन 2 दिनों के बाद B हट गया और A ने कार्य जारी रखा | पूरा कार्य कितने दिनों में समाप्त हुआ ?View SolutionA can do a work in 40 days and B can do the same work in 60 days. In how many days they can do it together?
View SolutionA can do a piece of work in 8 days, B can do it in 16 days, while C can do it in 80 days. In how many days they can complete the whole work, working together?
View SolutionA can do a piece of work in 10 days, B can do it in 12 days and C can do it in 15 days. In how many days they can complete the work, working together?
View SolutionA and B can do a piece of work in 72 days B and C can do it in 120 days. A and C can do it in 90 days. In how many days, all the three together can do the work?
View SolutionA can do a work in 45 days and B can do it in 40 days. Both starts the work together and after some days Aleaves the work. If the work is completed in 32 days, then for how many days A worked?
View SolutionA and B can do a work in 8 days. B alone can do it in 24 days. In how many days, A alone can do the same work?
View SolutionA can do a work in 20 days. B can do a work in 30 days and C can do a work in 60 days. If 'B' and 'C' helps A every third day, then how many days after work will complete?
View Solution