In the given figure, AB=AC, CH=CB and HK ||BC. If ∠CAX=137∘and∠CHK=K∘, then the value of K is…… 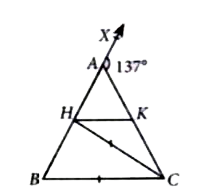
Video Solution
Text Solution
The correct Answer is:B
|
Answer
Step by step video, text & image solution for In the given figure, AB=AC, CH=CB and HK ||BC. If angleCAX=137^@ and angleCHK=K^@, then the value of K is…… by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - TRIANGLESEXERCISE - EXERCISE (Multiple Choice Questions) (MATCH THE FOLLOWING)2videos
TRIANGLES
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - TRIANGLESEXERCISE - EXERCISE (Multiple Choice Questions) (ASSERTION & REASON TYPE)5videos
TRIANGLES
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - TRIANGLESEXERCISE - EXERCISE (Multiple Choice Questions) (LEVEL-1 )35videos
TRIANGLES
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - STATISTICSEXERCISE - Olympiad/HOTS Corner20videos
STATISTICS
Similar Questions
Knowledge Check
- Question 1 - Select One
In the given figure AD=BC,AC=BD. Then ΔPAB is
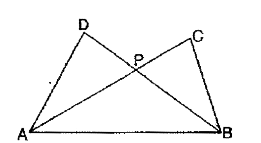 AequilateralBright angledCscaleneDisosceles
AequilateralBright angledCscaleneDisosceles - Question 2 - Select One
In the given figure, BC=AC=AD,∠EAD=81∘. Find the value of x :
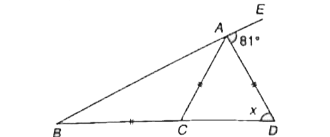 A45∘B54∘C63∘D36∘
A45∘B54∘C63∘D36∘
In the given figure, if DE || AC , then AB =
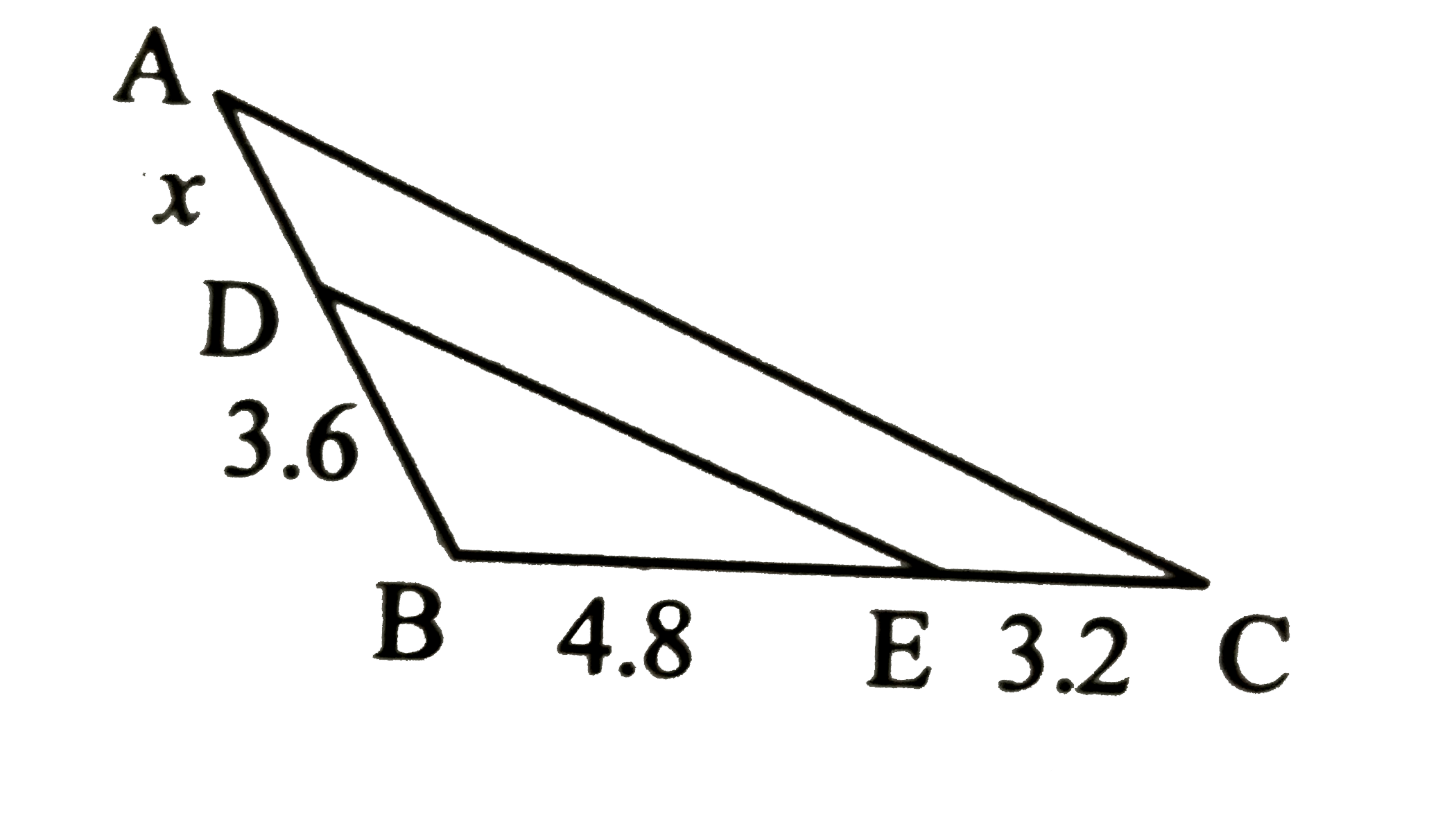 View Solution
View SolutionIn the given figure, AB∣∣CD and BC∣∣ED. Find the value of x.
 View Solution
View SolutionIn the given figure AB||CE and BC||FG, then value of x is.
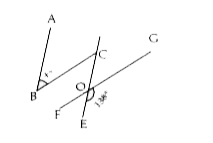 View Solution
View SolutionIn the given figure of AD = CB then prove that AC = BD
 View Solution
View SolutionIn the given figure , if AB=BC and ∠A=∠C then find the value of x
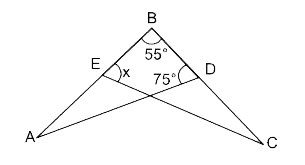 View Solution
View SolutionIn the given figure, AC=AE,AB=AD and ∠BAD=∠EAC. Prove that BC=DE.
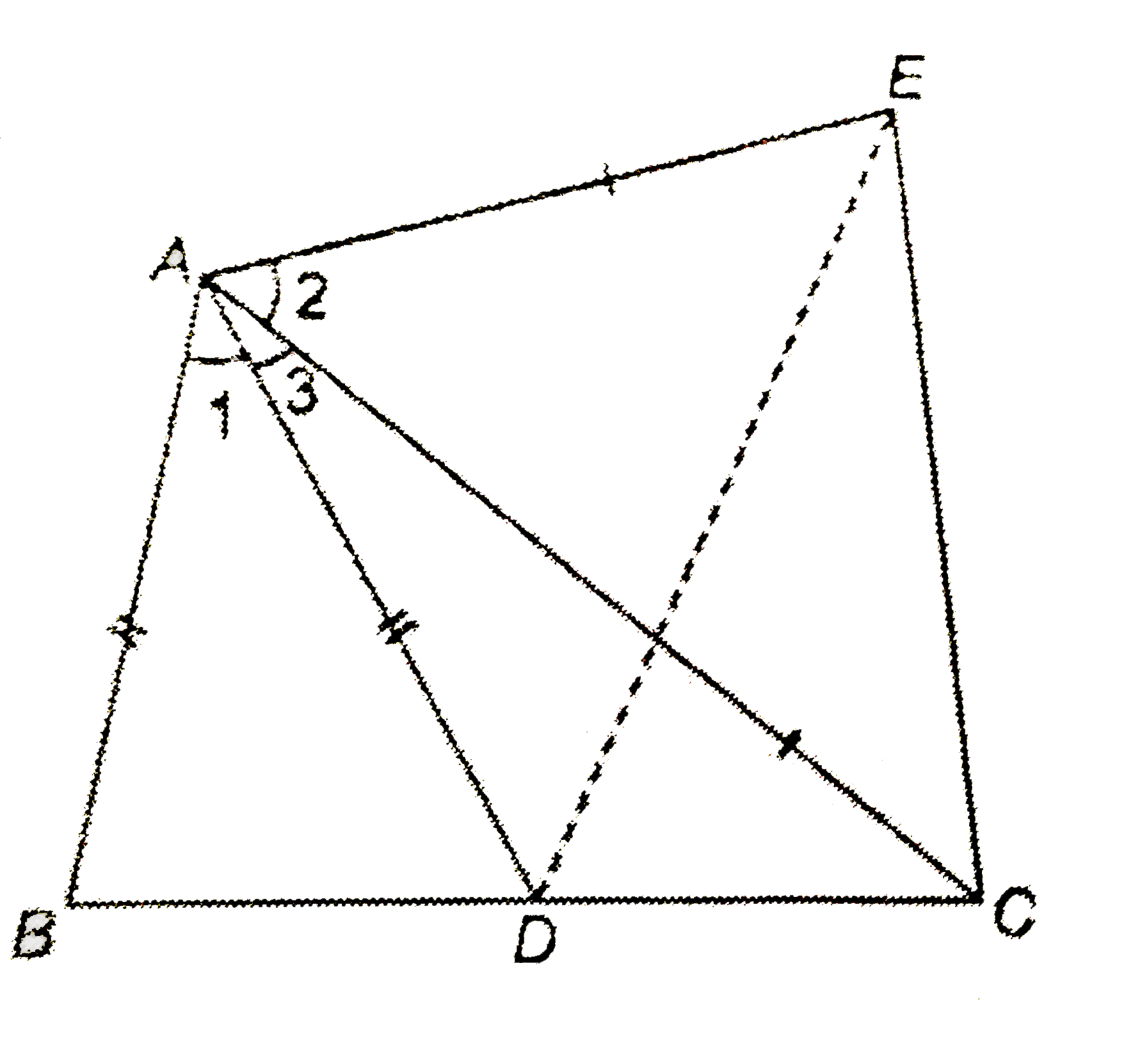 View Solution
View Solution