A continuous and differentiable function y = f(x) is such that its graph cuts line y = mx + c at n distinct points. Then the minimum number of points at which f''(x) = 0 is/are Solution in Malayalam
n - 1
n - 3
n - 2
cannot say
The correct Answer is:C
Step by step video, text & image solution for A continuous and differentiable function y = f(x) is such that its graph cuts line y = mx + c at n distinct points. Then the minimum number of points at which f''(x) = 0 is/are by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Which of the following is true about point of extremum x=a of function y=f(x)? At x=a, function y=f(x) may be discontinuous. At x=a, function y=f(x) may be continuous but non-differentiable. At x=a ,function y=f(x) may have point of inflection. none of these
View SolutionA function y = f(x) has a second-order derivative f''(x)=6(x−1). If its graph passed through the point (2,1) and at that point tangent to the graph is y=3x−5, then the value of f(0) is
View SolutionA continuous and differentiable function y=f(x) is such that its graph cuts line y=mx+c at n distinct points. Then the minimum number of points at which f''(x)=0 is/are
View Solutionएक सतत फलन f के अवकलज f' का ग्राफ चित्र में दर्शाया गया है तथा f(0)=0 है, तो फलन y=f(x) के लिए ज्ञात कीजिए -
नति परिवर्तन बिन्दु
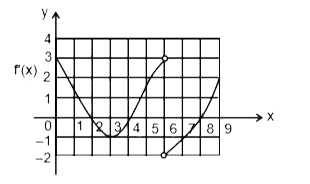
View Solutionएक सतत फलन f के अवकलज f' का ग्राफ चित्र में दर्शाया गया है तथा f(0)=0 है, तो फलन y=f(x) के लिए ज्ञात कीजिए -
क्रान्तिक बिन्दु
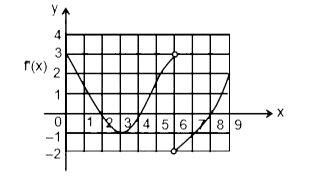
View SolutionThe graph of y=f''(x) for a function f is shown.
Number of points of inflection for y=f(x) is….. .

View SolutionA continuous and differentiable function y=f(x) is such that its graph cuts line y=mx+c at n distinct points. Then the minimum number of points at which f''(x)=0 is/are (a)n−1 (b) n−3 n−2 (d) cannot say
View SolutionA continuous and differentiable function y=f(x) is such that its graph cuts line y=mx+c at n distinct points. Then the minimum number of points at which f(x)=0 is/are
(a) n−1
(b) n−3
(c) n−2
(d) cannot say
View Solution
