Question

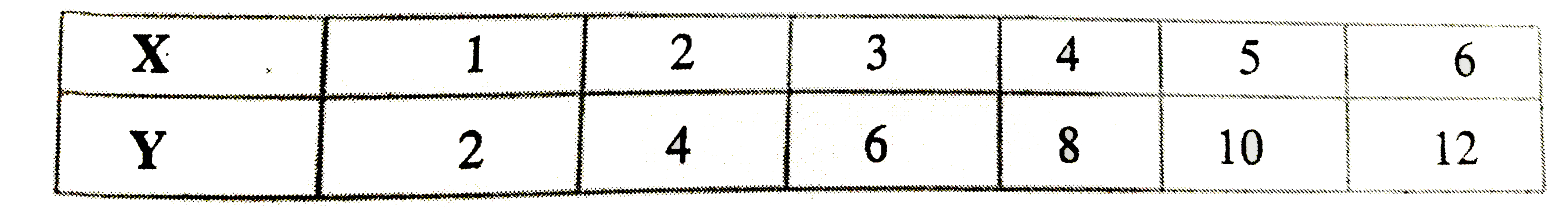
If two regression lines coincide with each other, there is no correlation between the variates

Answer

No

Explanation

To determine whether the statement "If two regression lines coincide with each other, there is no correlation between the variates" is true or false, we will analyze the properties of regression lines and correlation.

1. Understanding Regression Lines:
- There are two regression lines: one is the regression of Y on X (denoted as Y on X) and the other is the regression of X on Y (denoted as X on Y).
- The equations of these lines can be represented as:
- \( Y = B_{Y|X}X + C_1 \) (Regression of Y on X)
- \( X = B_{X|Y}Y + C_2 \) (Regression of X on Y)

2. Coinciding Regression Lines:
- If the two regression lines coincide, it means that they are identical. This implies that the slopes of the two regression lines are equal, i.e., \( B_{Y|X} = B_{X|Y} \).
- Coinciding lines suggest a perfect linear relationship between the two variables.

3. Correlation Coefficient:
- The correlation coefficient \( r \) measures the strength and direction of a linear relationship between two variables.
- The slopes of the regression lines are related to the correlation coefficient:
- \( B_{Y|X} = r \cdot \frac{s_Y}{s_X} \)
- \( B_{X|Y} = r \cdot \frac{s_X}{s_Y} \)
- Here, \( s_Y \) and \( s_X \) are the standard deviations of Y and X, respectively.

4. Analyzing the Statement:
- If the regression lines coincide, it indicates that there is a perfect correlation (either positive or negative) between the two variables.
- If there were no correlation (i.e., \( r = 0 \)), the regression lines would not coincide. Instead, they would represent different constants (horizontal or vertical lines).

5. Conclusion:
- Since coinciding regression lines indicate a perfect correlation, the statement "If two regression lines coincide with each other, there is no correlation between the variates" is false.

Final Answer:
The statement is false.