If U=3X+1,V=Y+2 and rXY=0.6 then rUV is
0.2
−0.2
0.6
none of these
The correct Answer is:C
To solve the problem, we need to find the correlation coefficient rUV given the transformations U=3X+1 and V=Y+2, along with the correlation coefficient rXY=0.6.
1. Identify the transformations: We have U=3X+1 and V=Y+2.
- Here, a=3 (the coefficient of X) and c=1 (the coefficient of Y).
Hint: Look for the coefficients of the variables in the transformations to identify a and c.
2. Check the positivity of coefficients: Both a and c are positive.
- a=3>0
- c=1>0
Hint: Ensure that both coefficients are greater than zero to apply the correlation property.
3. Apply the correlation property: There is a property of correlation coefficients that states:
raX+b,cY+d=rXY
if a>0 and c>0. In our case, since both a and c are positive, we can use this property.
Hint: Remember that the correlation coefficient remains unchanged under linear transformations when the coefficients are positive.
4. Calculate rUV: Since the property holds, we have:
rUV=rXY
Given rXY=0.6, we find:
rUV=0.6
Hint: Substitute the known correlation coefficient directly into the equation.
5. Conclusion: The value of rUV is 0.6.
Final Answer:
rUV=0.6
- Here, a=3 (the coefficient of X) and c=1 (the coefficient of Y).
Hint: Look for the coefficients of the variables in the transformations to identify a and c.
- a=3>0
- c=1>0
raX+b,cY+d=rXY
if a>0 and c>0. In our case, since both a and c are positive, we can use this property.
rUV=rXY
Given rXY=0.6, we find:
rUV=0.6
rUV=0.6
Topper's Solved these Questions
- CORRELATION AND REGRESSION BOOK - ML KHANNACHAPTER - CORRELATION AND REGRESSION EXERCISE - PROBLEM SET (1) TRUE/FALSE
6 Videos
- CORRELATION AND REGRESSION BOOK - ML KHANNACHAPTER - CORRELATION AND REGRESSION EXERCISE - PROBLEM SET (1) (FILL IN THE BLANKS)
10 Videos
- CORRELATION AND REGRESSION BOOK - ML KHANNACHAPTER - CORRELATION AND REGRESSION EXERCISE - QUESTION (STATE GIVING REASONS, WHETHER EACH OF THE FOLLOWING STATEMENTS IS TRUE OR FALSE)
10 Videos
- CONCEPTS OF SET THEORY BOOK - ML KHANNACHAPTER - CONCEPTS OF SET THEORY EXERCISE - Self Assessment Test
13 Videos
- DEFINITE INTEGRALBOOK - ML KHANNACHAPTER - DEFINITE INTEGRALEXERCISE - Miscellaneous Questions (Assertion/Reason)
1 Video
Similar Questions
Knowledge Check
If U=aX+b,andV=−cY+d where a and c positive. If r(U,V)=0.6, then r(X,Y) is equal to
A0.6B−0.6C0.6caD0.6acIt is given that X=10,y=90σx=3.σy=12 and rxy=0.8 Then regression equation of X on Y is
AY=3.2X+58BX=3.2Y+58CX=-8+0.2YDY=-8+0.2XIn the circuit shown below E1=4.0V,R1=2Ω,E2=6.0V,R2=4Ω and R3=2Ω. The current I1 is
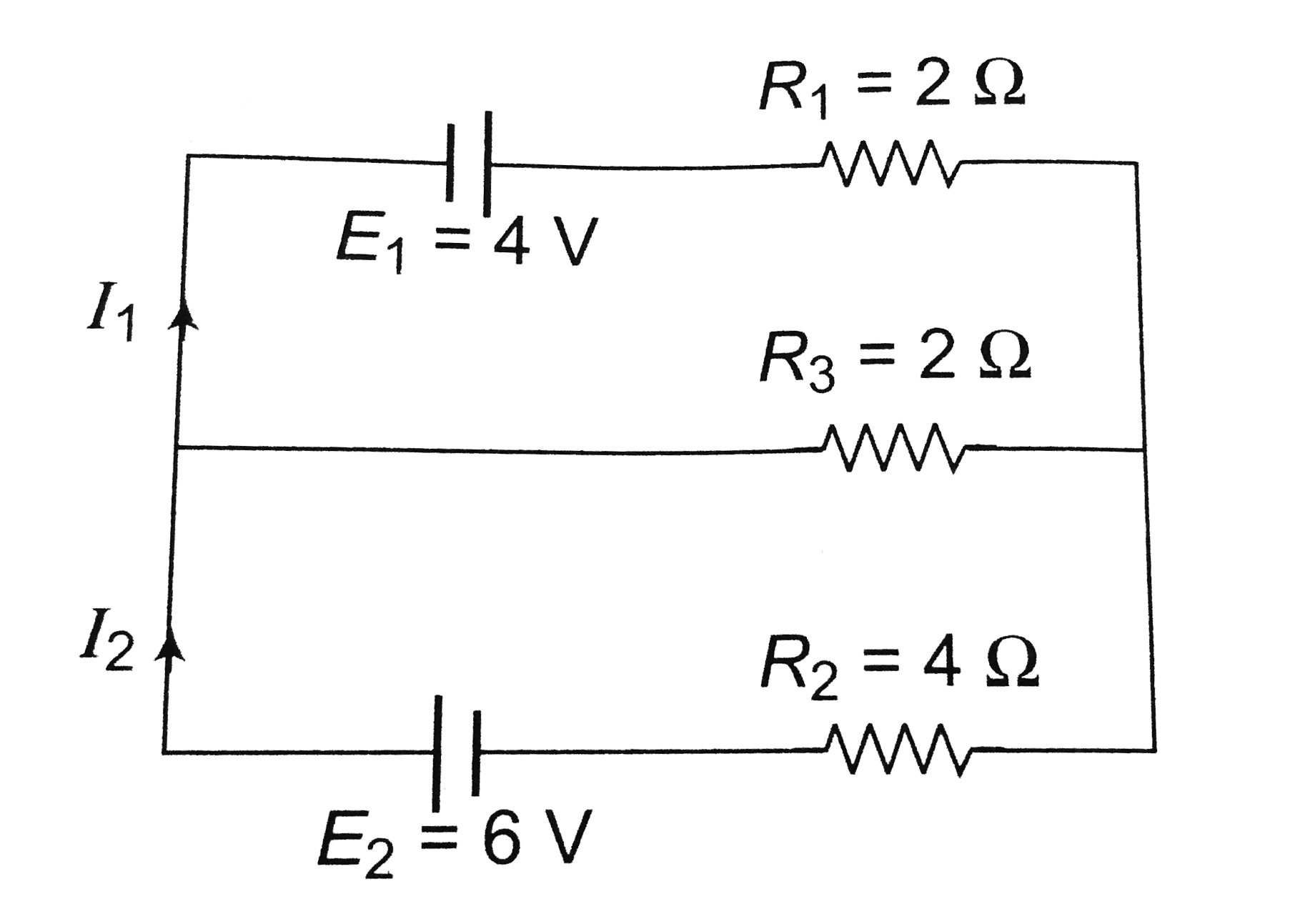 A1.6AB1.8AC1.25AD1.0A
A1.6AB1.8AC1.25AD1.0A
If R={(x',y):x∈W,Y∈W and (x+2y)2=36} then R−1 is__________. (a) {(0,3),(2,3),(1,4),(0,6)} (b) {(0,6),(0,3),(2,2),(4,1)} (c) {(3,0),(2,2),(1,4),(0,6)} (d) {(3,0),(2,2),(1,4),(6,0)}
View SolutionIf f:R→R be a function such that f(x+2y)=f(x)+f(2y)+4xy,∀x,y and f(2)=4 then, f(1)−f(0) is equal to
View SolutionIf r1,r2 and r3 are the radii of the circle x2+y2−4x+6y=5 ,
x2+y2+6x−4y=3 and x2+y2−2x+4y=8
View SolutionIf 5u+3v=13uvandu−v=uv, then (u,v)= _____
View SolutionThe coefficient of correlation between X and Y is 0.6. Their covariance is 4.8 var (x)=9. Find the value of σy.
View Solution