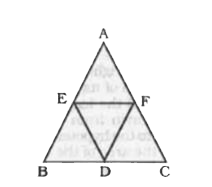
If D, E and Fare the mid points of BC, CA and AB respectively of the ABC then the ratio of area of the parallelogram DEFB and area of the trapezlum CAFD is:
2:3
3:4
1:2
1:3
The correct Answer is:A
To solve the problem of finding the ratio of the area of the parallelogram DEFB to the area of the trapezium CAFD in triangle ABC, we can follow these steps:
1. Identify the Midpoints:
Let D, E, and F be the midpoints of sides BC, CA, and AB of triangle ABC, respectively.
2. Understanding the Parallelogram:
Since D and E are midpoints, line segment DE is parallel to side AB and is half its length (by the midpoint theorem). Similarly, line segment FB is parallel to side AC and is half its length. Thus, DEFB forms a parallelogram.
3. Area of Parallelogram DEFB:
The area of parallelogram DEFB can be expressed in terms of the areas of triangles formed within the triangle ABC. Since DEFB is made up of two triangles (BDF and FED), we can denote the area of each triangle as X. Therefore, the total area of parallelogram DEFB is:
Area of DEFB=2X
4. Understanding the Trapezium:
The trapezium CAFD consists of triangles AEF and CDF. The area of triangle AEF can be denoted as X and the area of triangle CDF can also be denoted as X. Additionally, triangle DEF (which is also part of CAFD) has an area of X. Thus, the total area of trapezium CAFD is:
Area of CAFD=3X
5. Finding the Ratio:
Now, we can find the ratio of the area of parallelogram DEFB to the area of trapezium CAFD:
Ratio=Area of DEFBArea of CAFD=2X3X=23
6. Final Ratio:
Therefore, the ratio of the area of parallelogram DEFB to the area of trapezium CAFD is:
2:3
Let D, E, and F be the midpoints of sides BC, CA, and AB of triangle ABC, respectively.
Since D and E are midpoints, line segment DE is parallel to side AB and is half its length (by the midpoint theorem). Similarly, line segment FB is parallel to side AC and is half its length. Thus, DEFB forms a parallelogram.
The area of parallelogram DEFB can be expressed in terms of the areas of triangles formed within the triangle ABC. Since DEFB is made up of two triangles (BDF and FED), we can denote the area of each triangle as X. Therefore, the total area of parallelogram DEFB is:
Area of DEFB=2X
The trapezium CAFD consists of triangles AEF and CDF. The area of triangle AEF can be denoted as X and the area of triangle CDF can also be denoted as X. Additionally, triangle DEF (which is also part of CAFD) has an area of X. Thus, the total area of trapezium CAFD is:
Area of CAFD=3X
Now, we can find the ratio of the area of parallelogram DEFB to the area of trapezium CAFD:
Ratio=Area of DEFBArea of CAFD=2X3X=23
Therefore, the ratio of the area of parallelogram DEFB to the area of trapezium CAFD is:
2:3
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - TRY YOURSELF
25 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XIII)
70 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
Knowledge Check
If D, E and F are the mid points of BC, CA and AB respectively of the △ABC then the ratio of aria parallelogram DEFB and area of the trapezium CAFD is ,
A2:3B3:4C1:2D1:3If D, E and Fare the mid points of BC, CA and AB respectively of the ABC then the ratio of area of the parallelogram DEFB and area of the trapezlum CAFD is:
A1:2B2:3C3:4D1:1If D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
A1:4B1:2C1:3D2:3
In the adjoining figure D, E and F are the mid-points of the sides BC, CA and AB respectively of ΔABC. Prove that:
(i) □BDEF is a parallelogram
(ii) area of ΔDEF=14× area of ΔABC
(iii) area of □BDEF=12× area of ΔABC

View SolutionIf D, E and F are the mid-points of the sides of an equilateral triangle ABC, then the ratio of the area of triangle DEF and DCF is :
View SolutionD, E and F are the mid-points of the sides BC, CA and AB of a triangle ABC. Show that
BDEF is a parallelogram
View SolutionIn ΔABC,DandE are the midpoint of AB and AC respectively. Find the ratio of the areas of ΔADEandΔABC.

View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
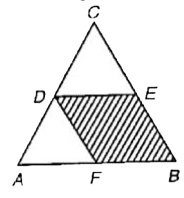
View Solution
