In ΔABC,DandE are the midpoint of AB and AC respectively. Find the ratio of the areas of ΔADEandΔABC.

The correct Answer is:1:4
Clearly DE∣∣BC.
∴ΔADE~ΔABC.
∴ar(ΔADE)ar(ΔABC)=AD2AB2=AD2(2AD)2=14
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7D
22 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7E
30 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7B
19 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
Knowledge Check
ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
A4:1B5:1C3:1Dcan't be determindABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
A4:1B5:1C3:1Dcan't be determinedΔABC is an equilateral triangle and D and E are midpoints of AB and BC respectively. Then the area of ΔABC : the area of the trapezium ADEC is
A5:3B4:1C8:5D4:3
In a ΔABC, F and E are the mid point of AB and AC respectively. G is the centroid of ΔABC. If X, Y and Z are the mid points of EF, FG & GE respectively. Find the ratio of area of ΔXYZ to area of ΔABC.
View SolutionIn ΔABC,D,E,F are respectively the mid points of the sides AB, BC and AC. Find ratio of the area of ΔDEF and area of ΔABC.
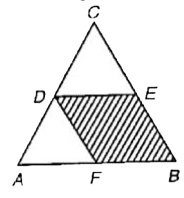
View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
View SolutionIn a ΔABC,D,EandF are the mid-point of sides BC, CA and AB respectively. If area of ΔABC=16cm2, find the area of trapezium FBCE
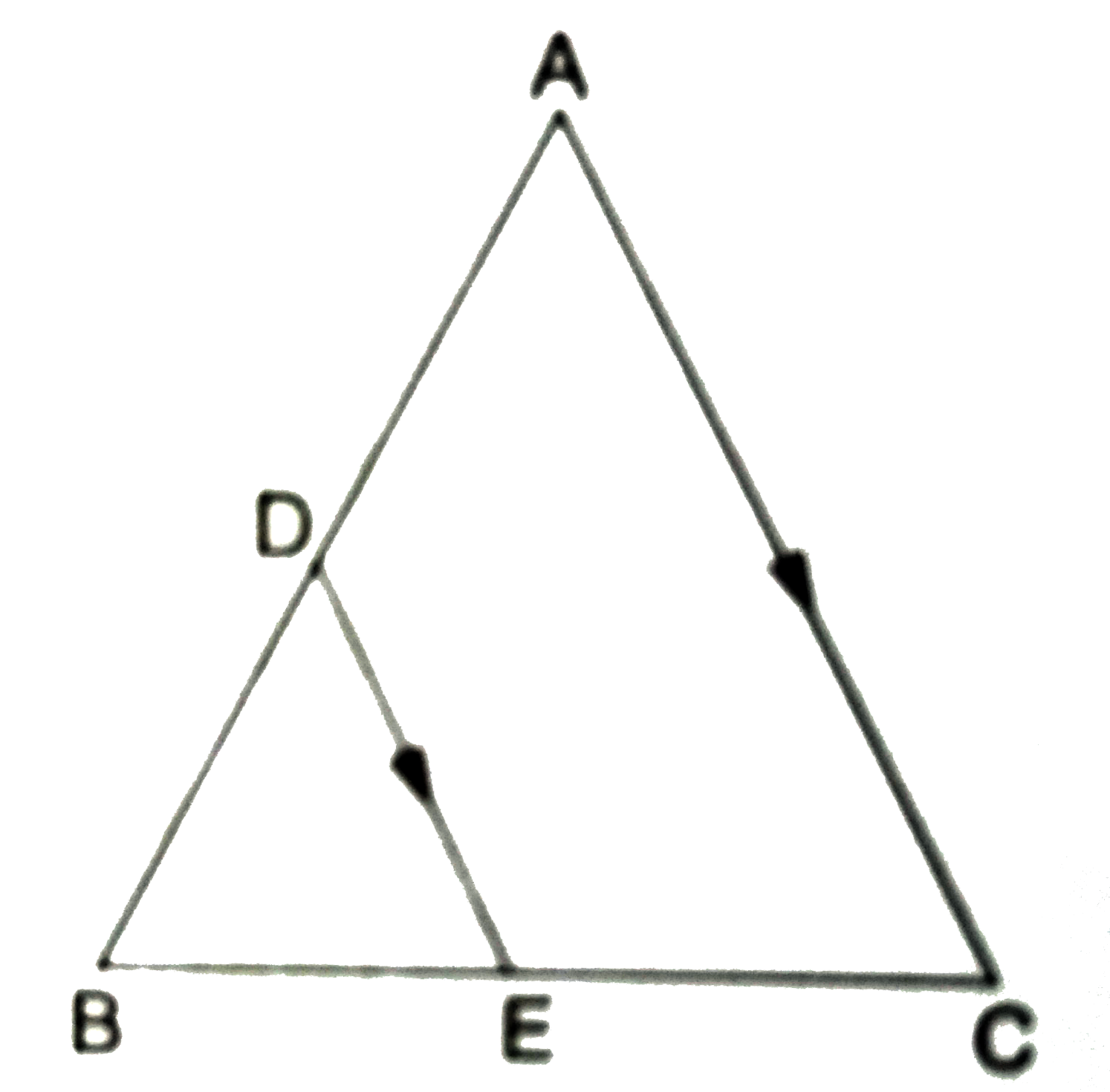
View SolutionIf D is a point on the side AB of ΔABC such that AD:BD=3:2andE is a point on BC such that DE∣∣AC, find the ratio of the areas of ΔABCandΔDBE
View Solution

