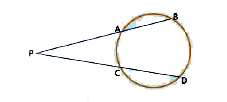
Two chords ABandPQ of a circle intersect at D inside a circle. If AD = 4 cm., DB = 6 cm., QD = 3 cm., the length of PQ is equal to
11 cm
8 cm
9 cm.
10 cm.
The correct Answer is:A
To solve the problem, we will use the intersecting chords theorem, which states that if two chords intersect inside a circle, the products of the lengths of the segments of each chord are equal.
1. Identify the lengths of the segments:
- Given:
- AD=4cm
- DB=6cm
- QD=3cm
- We need to find the length of PQ.
2. Use the intersecting chords theorem:
- According to the theorem:
AD×DB=PD×QD
- Substitute the known values:
4×6=PD×3
3. Calculate PD:
- Calculate the left side:
24=PD×3
- To find PD, divide both sides by 3:
PD=243=8cm
4. Calculate the length of PQ:
- The length of PQ is the sum of PD and QD:
PQ=PD+QD
- Substitute the values:
PQ=8+3=11cm
5. Final Answer:
- The length of PQ is 11cm.
Summary:
The length of chord PQ is 11cm.
- Given:
- AD=4cm
- DB=6cm
- QD=3cm
- We need to find the length of PQ.
- According to the theorem:
AD×DB=PD×QD
- Substitute the known values:
4×6=PD×3
- Calculate the left side:
24=PD×3
- To find PD, divide both sides by 3:
PD=243=8cm
- The length of PQ is the sum of PD and QD:
PQ=PD+QD
- Substitute the values:
PQ=8+3=11cm
- The length of PQ is 11cm.
The length of chord PQ is 11cm.
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XIII)
70 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XIV)
90 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XI)
22 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
Knowledge Check
Chords AB and CD of a circle intersect at a point P inside the circle. If AB = 10 cm, AP = 4 cm and PC = 5 cm, then CD is equal to:
A4.8 cmB6.8 cmC9.8 cmD7.8 cmChords AB and CD of a circle intersect externally at P. If AB=6cm,CD=3cmandPD=5cm, then the length of PB is
A5 cmB7.35 cmC6 cmD4 cmTwo chords AB and PQ of a circle mutually intersect at an outside point D. If AD=12 cm, AB=8 cm, DQ=6 cm then find PQandPD.
AA)2&6BB)2&8CC)3&6DD)5&7
The chords AB and CD of a circle intersect at E. If AE = 12cm, BE = 20.25 cm and CE = 3DE, then the length (in cm) of CE is:
एक वृत्त की दो जीवाएँ AB तथा CD एक-दूसरे को E पर काटती हैं | यदि AE = 12 सेमी, BE =20.25 सेमी तथा CE = 3DE है, तो CE की लंबाई ( सेमी में ) कितनी होगी ?
View SolutionChords AB and CD of a circle Intersect at E. If AE = 9 cm, BE = 12 cm and CE = 3DE, then the length of DE (in cm) is
View SolutionVinod have 20 rupees. He bought 1, 2, 5 rupee stamps. They are different in numbers by the reason of no change, the shop keeper gives 3 one rupee stamps. So how many stamps Vinod have ?
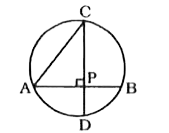
View SolutionTwo mutually perpendicular chords AB and CD intersect at point P inside the circle such that CP=15cm.,PD=8cmandPB=6cm. Find the length AC (in cm.)
View SolutionTwo chords AB and CD of a circle are produced to intersect each other at point P outside the circle. If AB=7 cm, BP = 4.2 cm and PD = 2.8 cm, then the length of CD is: /
एक वृत्त की दो जीवाओं AB और CD को बढ़ाया जाता है जो एक-दूसरे को वृत्त के बाहर बिंदु ? पर काटती हैं| यदि AB = 7 सेमी, BP = 4.2 सेमी तथा तथा PD = 2.8 सेमी है, तो CD की लंबाई कितनी है ?
View Solution