Question

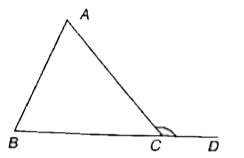
In a triangle ABC, the side BC is extended up to D. Such that $CD=AC$, if $\angle BAD={109}^{\circ }$ and $\angle ACB={72}^{\circ }$ then the value of $\angle ABC$is

Options

A. `35^@`
B. `60^@`
C. ` 40^@`
D. `45^@`

Answer

A

Explanation

To solve the problem step by step, we will analyze the triangle ABC with the given conditions.

Step 1: Draw the Diagram
Draw triangle ABC and extend side BC to point D such that CD = AC. Mark the angles as given: ∠BAD = 109° and ∠ACB = 72°.

Hint: Always start by visualizing the problem with a diagram. It helps in understanding the relationships between the angles and sides.

Step 2: Identify Isosceles Triangle
Since CD = AC, triangle ACD is isosceles. Therefore, the angles opposite to equal sides are equal. Let the angles ∠CAD and ∠CDA both be θ.

Hint: Recall that in an isosceles triangle, the angles opposite the equal sides are equal.

Step 3: Set Up the Equation for Angles in Triangle ACD
The sum of angles in triangle ACD is:
\( \angle CAD + \angle ACD + \angle CDA = 180° \)
Substituting the known values:
\( θ + 72° + θ = 180° \)
This simplifies to:
\( 2θ + 72° = 180° \)

Hint: Use the property that the sum of angles in a triangle is always 180°.

Step 4: Solve for θ
Rearranging the equation gives:
\( 2θ = 180° - 72° \)
\( 2θ = 108° \)
\( θ = 54° \)

Hint: Isolate the variable to find its value.

Step 5: Analyze Triangle ABD
Now, consider triangle ABD. We know:
- ∠BAD = 109°
- ∠CAD = θ = 54°

Let ∠ABC = α (the angle we want to find). The sum of angles in triangle ABD gives us:
\( ∠BAD + ∠ABC + ∠CAD = 180° \)
Substituting the known values:
\( 109° + α + 54° = 180° \)

Hint: Again, apply the triangle angle sum property.

Step 6: Solve for α
Rearranging the equation gives:
\( α = 180° - 109° - 54° \)
\( α = 180° - 163° \)
\( α = 17° \)

Hint: Carefully perform the arithmetic to ensure accuracy.

Final Answer
Thus, the value of ∠ABC is:
\( \boxed{17°} \)