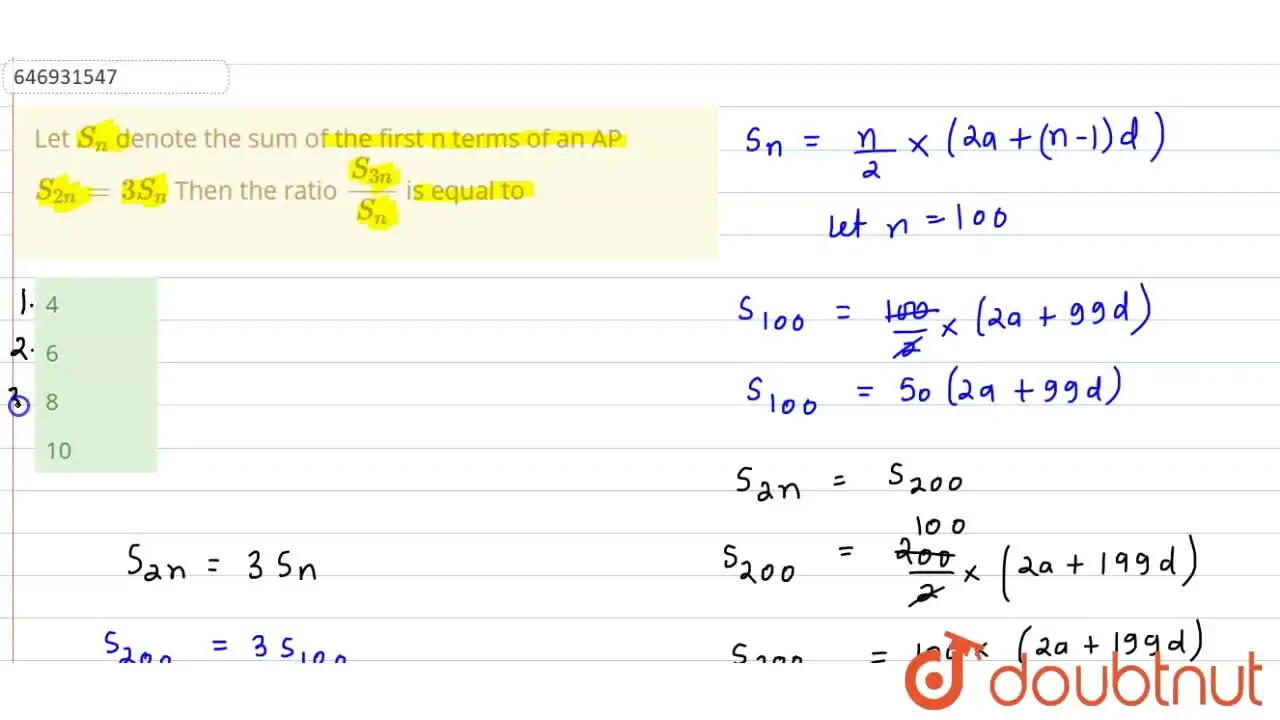
Let Sn denote the sum of the first n terms of an AP
S2n=3Sn Then the ratio S3nSn is equal to
4
6
8
10
The correct Answer is:B
Step by step video, text & image solution for Let S_n denote the sum of the first n terms of an AP S_(2n)=3S_n Then the ratio S_(3n)/S_n is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - KIRAN PUBLICATIONCHAPTER - SEQUENCE AND SERIES EXERCISE - TYPE V19videos
SEQUENCE AND SERIES
- BOOK - KIRAN PUBLICATIONCHAPTER - RATIO AND PROPORTION EXERCISE - TEST YOURSELF19videos
RATIO AND PROPORTION
- BOOK - KIRAN PUBLICATIONCHAPTER - SIMPLE INTERSETEXERCISE - TEST YOURSELF25videos
SIMPLE INTERSET
Similar Questions
Let Sn denote the sum of the first n terms of an AP
S2n=3Sn Then the ratio S3nSn is equal toView SolutionLet Sn denote the sum of the first n terms of an AP. If S2n=3S_n,thenS_(3n):S_n is equal to
View SolutionLet Sn denotes the sum of n terms of an AP. If S2n=3Sn, then the ratio S3nSn is equal to
View SolutionLet Sn denotes the sum of the first of n terms of A.P. and S2n=3Sn . then the ratio S3n:Snis equal to
View SolutionSn denote the sum of the first n terms of an A.P. If S2n=3Sn , then S3n:Sn is equal to
View SolutionLet Sn denote the sum of first n terms of an A.P. If S2n=3Sn, then find the ratio S3n/Sn.
View Solution(1) .Let Sn denote the sum of the first 'n' terms and S2n=3Sn. Then, the ratio S3n:Sn is: (2) let S1(n) be the sum of the first n terms arithmetic progression 8,12,16,..... and S2(n) be the sum of the first n terms of arithmatic progression 17,19,21,....... if S1(n)=S2(n) then this common sum is
View SolutionLet Sn denote the sum of the first n tem of an A.P. If S2n=3Sn then prove that S3nSn=6.
View SolutionLet Sn denote the sum of the first n terms of an AP
S2n=3Sn Then the ratio S3nSn is equal toView SolutionLet Sn denotes the sum of first n terms of an AP and 3Sn=S2n.
Q. What is S3n:Sn equal to?View Solution