The railway fares of air conditioned sleeper and ordinary sleeper class are in the ratio 4:1. The number of passengers travelled by air conditioned sleeper and ordinary sleeper classes were in the ratio 3:25. If the total collection was Rs. 37,000, how much did air conditioner sleeper passengers pay ?
Rs. 15,000
Rs. 10,000
Rs. 12,000
Rs. 16,000
The correct Answer is:C
To solve the problem step by step, we will follow the ratios provided and calculate the total fare paid by the air-conditioned sleeper passengers.
Step 1: Define the Ratios
The fares of the air-conditioned sleeper and ordinary sleeper classes are in the ratio of 4:1.
Let:
- The fare for air-conditioned sleeper (AC) = 4x
- The fare for ordinary sleeper (ORD) = 1x
Step 2: Define the Passenger Ratios
The number of passengers traveling in air-conditioned sleeper and ordinary sleeper classes are in the ratio of 3:25.
Let:
- Number of AC passengers = 3y
- Number of ORD passengers = 25y
Step 3: Calculate Total Collection from Each Class
Now, we can calculate the total collection from each class:
- Total collection from AC passengers = Number of AC passengers×Fare for AC=3y×4x=12xy
- Total collection from ORD passengers = Number of ORD passengers×Fare for ORD=25y×1x=25xy
Step 4: Calculate Total Collection
The total collection from both classes is:
Total Collection=Collection from AC+Collection from ORD=12xy+25xy=37xy
Step 5: Set Up the Equation
We know the total collection is Rs. 37,000:
37xy=37000
Step 6: Solve for xy
To find xy, divide both sides by 37:
xy=3700037=1000
Step 7: Calculate the Amount Paid by AC Passengers
Now, we need to find the amount paid by the air-conditioned sleeper passengers:
Amount paid by AC passengers=12xy=12×1000=12000
Conclusion
Thus, the amount paid by the air-conditioned sleeper passengers is Rs. 12,000.
---
The fares of the air-conditioned sleeper and ordinary sleeper classes are in the ratio of 4:1.
Let:
- The fare for air-conditioned sleeper (AC) = 4x
- The fare for ordinary sleeper (ORD) = 1x
The number of passengers traveling in air-conditioned sleeper and ordinary sleeper classes are in the ratio of 3:25.
Let:
- Number of AC passengers = 3y
- Number of ORD passengers = 25y
Now, we can calculate the total collection from each class:
- Total collection from AC passengers = Number of AC passengers×Fare for AC=3y×4x=12xy
- Total collection from ORD passengers = Number of ORD passengers×Fare for ORD=25y×1x=25xy
The total collection from both classes is:
Total Collection=Collection from AC+Collection from ORD=12xy+25xy=37xy
We know the total collection is Rs. 37,000:
37xy=37000
To find xy, divide both sides by 37:
xy=3700037=1000
Now, we need to find the amount paid by the air-conditioned sleeper passengers:
Amount paid by AC passengers=12xy=12×1000=12000
Thus, the amount paid by the air-conditioned sleeper passengers is Rs. 12,000.
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - RATIO AND PROPORTION EXERCISE - TEST YOURSELF19videos
RATIO AND PROPORTION
- BOOK - KIRAN PUBLICATIONCHAPTER - RATIO AND PROPORTION EXERCISE - TYPE-VIII5videos
RATIO AND PROPORTION
- BOOK - KIRAN PUBLICATIONCHAPTER - PROFIT AND LOSS EXERCISE - TEST YOURSELF23videos
PROFIT AND LOSS
- BOOK - KIRAN PUBLICATIONCHAPTER - SEQUENCE AND SERIES EXERCISE - TEST YOURSELF20videos
SEQUENCE AND SERIES
Similar Questions
In an express train, the number of passengers travelling in AC sleeper class, first class and sleeper class are in the ratio 1:2:3 and the fares of each classes are in the ratio 5:4:2. If the total income from this train is Rs 54000, then what is the income from AC sleeper class?
View SolutionIn an express train, the number of passengers travelling in A.C. sleeper class, first class and sleeper class are in the ratio 1 : 2 : 3 and the fares to each of these classes are in the ratio 5:4:2. If the total income from this train is 45600, then the income from the A.C. sleeper class is Rs.6000 b. R.8000 c. Rs.10000 d. Rs.12000
View Solution
Knowledge Check
- Question 1 - Select One
The ratio of the first and second class fares between two railway stations is 4 : 1 and that of the number of passengers travelling by first and second classes is 1 : 40. If on a specific day Rs 1,100 is collected as total fare, the amount collected from the first class passengers is :
ARs.275BRs.315CRs.137.50DRs.100 - Question 2 - Select One
The ratio of the first and second class fares between two railway stations is 4 : 1 and that of the number of passengers travelling by first and second classes is 1 : 40. If on a specific day Rs 1,100 is collected as total fare, the amount collected from the first class passengers is :
ARs 315BRs 275CRs 137.50DRs100 - Question 3 - Select One
The ratio of 1st and 2nd classes train fairs between two stations is 3 : 1 and that of the number of pas- sengers travelling between these stations by 1st and 2nd classes is 1 : 50. If on a particular day, X 2650 be collected from the passengers travelling between these stations, then find the amount collected from 2nd class passengers.
A? 750B? 850C? 1000D? 1250
The first Class fare from Chennai to Coimbatore is Rs. 557 and the sleeper class fare is Rs. 165. How much would a family of 4 members save by choosing to travel by sleeper class?
View SolutionRailway fares of 1st, 2nd and 3rd class between two stations were in the ratio of 8 6 : 3. The fares of 1st and 2nd class were subsequently reduced by 16and112 respectively. If during a year the ratio 12 between the passengers of 1st, 2nd and 3rd classes was 9 :12 : 26 and the total amount collected by the sale of tickets was Rs, 1088, then find the collection from the passengers of 1st class. Rs.260 b. Rs.280 c. Rs.300 d. Rs.320
View SolutionStudy the given information carefully and answer the questions that follow.
The table given below shows the capacity of 5 buses (A, B, C, D, E) and the percentage of seats that were filled out of the total seating capacity of the buses.
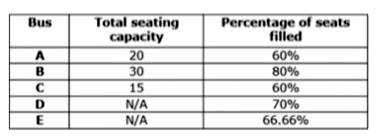
Some of the data is missing and is denoted as N/A
A) The total number fo vacant seats in all the 5 nuses together were 40
B) The number of seats in buses D and E together were 65
20% of the passengers on bus D got down at Yarivali bus stop and 2 passengers boarded the bus from the same bus stop. The conductor started charging from Yarivali bus stop. The number of adults and children on he bus were in the ratio 2:1. If the total collection of bus fare was Rs. 800, what was the bus fare for each child? Consider, there were no other stoppages till the final stop, The bus ticket was free till Yarivali bus stop and children's tricket price was half of that of adult.View SolutionOne day, the ratio of the number of first class and second class passengers who travelled were in the ratio 1:30. the ratio of the first and second class fares is 3:1. the total amount collected from the passerngers that day was Rs 66000. find the amount collected from the first class passengers (in Rs)
View SolutionThe ratio of the rail fares of first class and second class between the stations is 3:1 .The ratio of first class passenger and second class passengers is 1:50 .One day Rs. 1,325 is received as rail fare from passengers travelling both sides then the fare received from second class passangers is ?
View Solution
